Home » 国际竞赛 » 数模国际竞赛 » HiMCM数模竞赛 » Details

HIMCM数学建模竞赛真题获取，备战2023-2024年HIMCM比赛

Category: HiMCM数模竞赛, 国际竞赛 Date: 2023年6月28日下午5:04

数学建模是一门将数学知识应用于实际问题解决的重要领域。对于参加即将举行的HiMCM比赛的同学们来说，竞争激烈，想要在比赛中取得优异成绩就需要在最后关头进行强化训练。而对于那些着眼未来的同学来说，更需要提前准备，通过学习历年比赛的经典真题来增强自己的能力。

相信经过数模学术活动的“洗礼”，对于你挑战高层次的学术科研也必然打下坚实基础。

2021年HiMCM赛题

问题A：储存太阳

2021年HiMCM美国数学建模活动今日开赛！赛题公布，你有把握冲刺O奖吗？

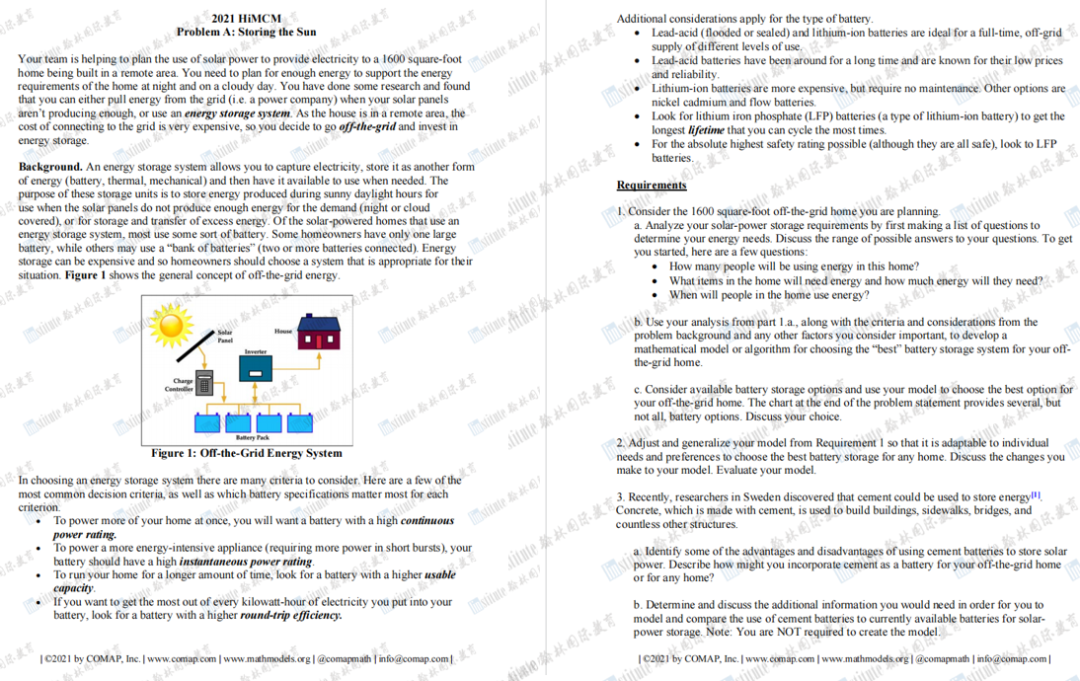
你的团队需要为一个偏远地区的1600平方英尺的住宅提供太阳能电力，满足家庭在晚上和阴天的能源需求。由于房子偏远，连接到电网的成本非常昂贵，所以你决定投资于能源存储。

对于储能系统和相关电池规格标准，题目中都给出了详细的介绍，学生需要为1600平方英尺的家庭做好能源需求规划，并给出合适的模型。同时讨论水泥电池的优缺点，并与太阳能电池进行比较，给出可行性建议。

问题B：应对干旱

2021年HiMCM美国数学建模活动今日开赛！赛题公布，你有把握冲刺O奖吗？

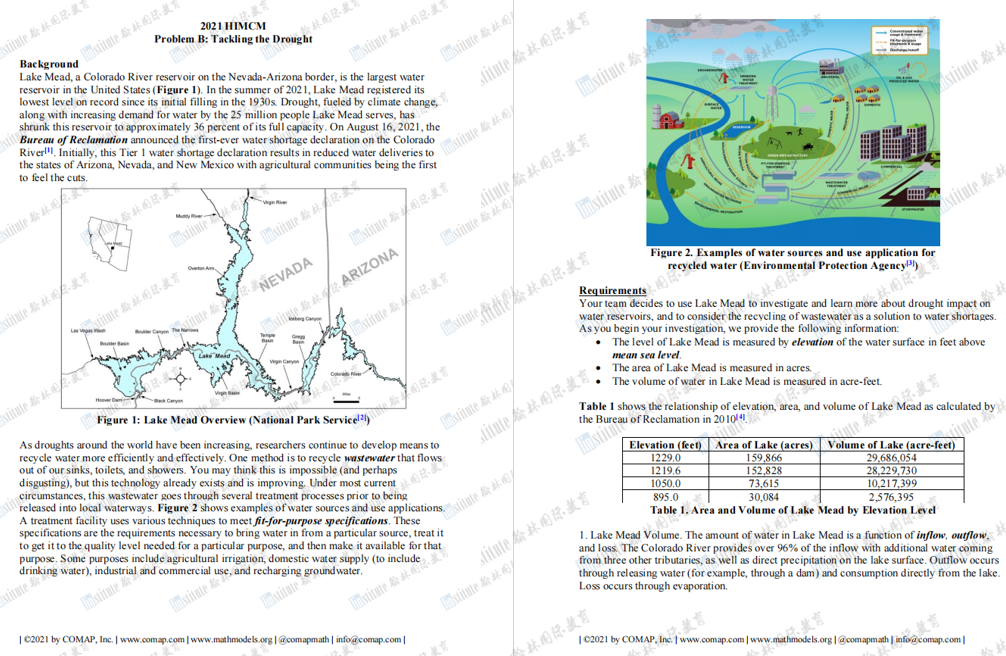
米德湖是美国最大的水库，但2021年的夏天米德湖达到了它自20世纪30年代初次蓄水以来的最低水平。由于气候变化引发的干旱，同时随着米德湖所服务的2500万人对水的需求的增加，
水库容量已经缩小到36%。

你的团队决定用米德湖案例来调查和了解更多关于干旱对水库的影响。学生需要讨论不同因素对米德湖体积和水位的影响，根据提供的历史数据简单讨论总体模型，对比不同时期的干旱，并开发两个模型分别预测2025、2030年和2050年的米德湖水位。根据模型和预测，考虑废水循环的可行性计划。

HiMCM2022年真题参考

Problem A: The Need for Bees

(and not just for honey)

Honeybees, along with a few other key animals, are critical to human existence on our planet. Along with honey production, these insects provide the vital role of pollination of many trees and plants that provide food for our survival. In 2007, the term Colony Collapse Disorder (CCD) was created to describe the decline of honeybee populations around the world. Bee decline can be attributed to factors such as viruses, pesticides, predators, habitat destruction, and environmental conditions.

Some information to consider (but you may find other helpful information online):

· Honeybees can travel up to 20 km, but typically stay within 6 km of their hive.

· A typical honeybee hive contains between 20,000 and 80,000 honeybees.

· A single honeybee can visit approximately 2,000 flowers or more in a single day.

· Because of the high workload during summertime, most honeybees work themselves to death, resulting in a shorter lifespan.

· During autumn and wintertime, honeybees may live a bit longer (four to six months).

· A honeybee’s level of activity, pollen consumption, and protein abundance impacts its lifespan.

Requirements

1. Develop a model to determine the population of a honeybee colony over time.

2. Conduct sensitivity analysis on your model to determine which factors (e.g., lifespans, egg laying rates, fertilized/unfertilized egg ratios, or other factors) have the greatest impact on honeybee colony size.

3. Model and predict how many honeybee hives you will need to support pollination of a 20-acre

(81,000 square meters) parcel of land containing crops that benefit from pollination.

4. Create a non-technical, one-page blog or infographic for a website that provides the information you developed.

Your PDF solution of no more than 25 total pages should include:

· One-page Summary Sheet.

· Table of Contents.

· Your complete solution.

· One-page blog or infographic.

· References list.

Note: The HiMCM Contest has a 25-page limit to your PDF solution submission. All aspects of your submission count toward the 25-page limit (Summary Sheet, Table of Contents, Reference List, and any Appendices).

Problem B: CO2 and Global Warming

Prior to the Industrial Revolution, carbon dioxide (CO2) in the atmosphere was consistently around 280 parts per million (ppm). The concentration of CO2 in the atmosphere reached 377.7 ppm in March of 2004, resulting in the largest 10-year average increase up to that time. According to scientists from National Oceanographic and Atmospheric Administration (NOAA) and Scripps Institution of Oceanography (SIO) the monthly mean CO2 concentration level peaked at 421 ppm in May 2022. An Organisation for Economic Co-Operations and Development (OECD) report predicts a CO2 level of 685 ppm by 2050.

The editors of Scientific Today magazine have asked your team to address these claims of the current reported and future predictions of CO2 concentration levels. They provided two data sets (CO2 Data Set 1 & Temps Data Set 2) to assist in your research.

Requirements

1. Do you agree with CO2 level claims? Use CO2 Data Set 1 to analyze CO2 changes.

a. Do you agree that the March 2004 increase of CO2 resulted in a larger increase than observed over any previous 10-year period? Why or why not?

b. Fit various (more than one) mathematical models to the data to describe past, and predict future, concentration levels of CO2 in the atmosphere.

c. Use each of your models to predict the CO2 concentrations in the atmosphere in the year 2100. Do any of your models agree with claims and predictions that the CO2 concentration level will reach 685 ppm by 2050? If not by 2050, when do your models predict the concentration of CO2 reaching 685 ppm?

d. Which model do you consider most accurate? Why?

2. What’s the relationship between temperature and CO2? Many scientists think that there is a relationship between warming global temperatures and the concentration of CO2 in the atmosphere.

Use your work in part 1 and Temps Data Set 2 to assist in your comparison of land-ocean temperatures and CO2 concentration levels.

a. Build a model to predict future land-ocean temperatures changes. When does your model predict the average land-ocean temperature will change by 1.25°C, 1.50°C, and 2°C compared to the base period of 1951-1980?

b. Build a model to analyze the relationship (if any) between CO2 concentrations and landocean temperatures since 1959. Explain the relationship or justify that there is no relationship.

c. Extend your model from part 2.b. into the future. How far into the future is your model reliable? What concerns, if any, do you have with your model’s ability to predict future CO2 concentration levels and/or land-ocean temperatures?

3. Prepare a non-technical article