- 翰林提供学术活动、国际课程、科研项目一站式留学背景提升服务!
- 400 888 0080
Edexcel A Level Maths: Pure:复习笔记8.3.2 Particular Solutions
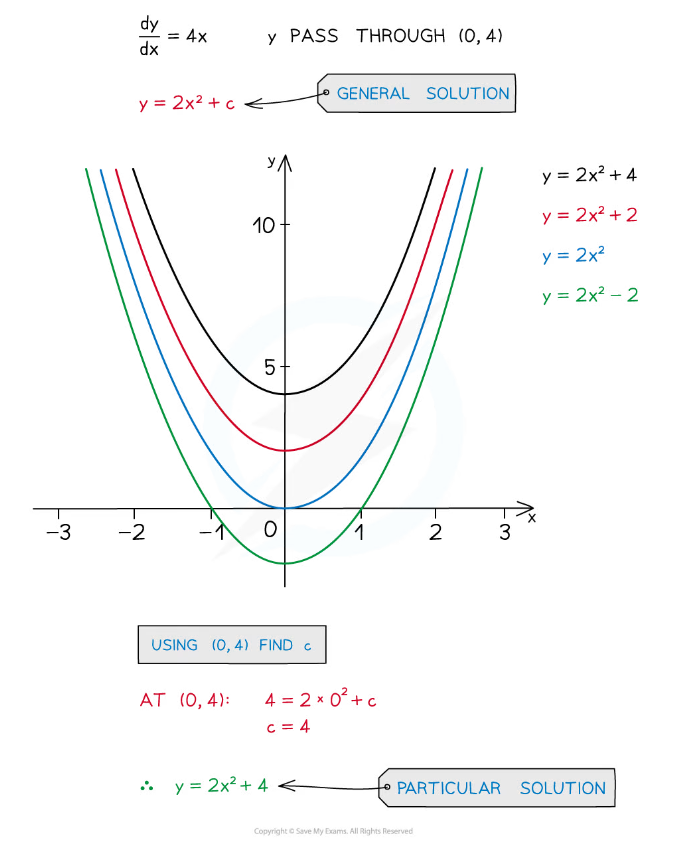
Particular Solutions
What is a particular solution?
- Ensure you are familiar with General Solutions first
- With extra information, the constant of integration, c, can be found
- This means the particular solution (from the family of solutions) can be found
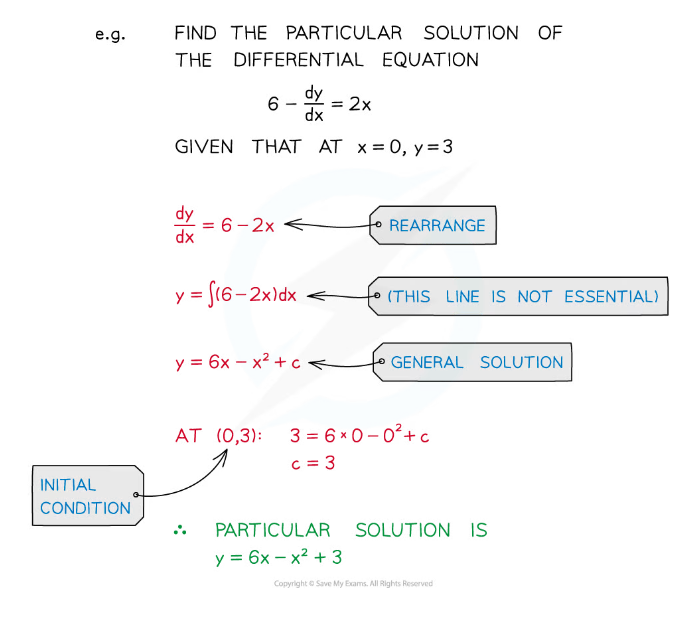
What is a boundary condition/initial condition?
- A boundary condition is a piece of extra information that lets you find the particular solution
- For example knowing y = 4 when x = 0 in the preceding example
- In a model this could be a particle coming to rest after a certain time, ie v = 0 at time t

- Differential equations are used in modelling, experiments and real-life situations
- A boundary condition is often called an initial condition when it gives the situation at the start of the model or experiment
- This is often linked to time, so t = 0
- It is possible to have two boundary conditions
- eg a particle initially at rest has velocity, v = 0 and acceleration, a = 0 at time, t = 0
- for a second order differential equation you need two boundary conditions to find the particular solution
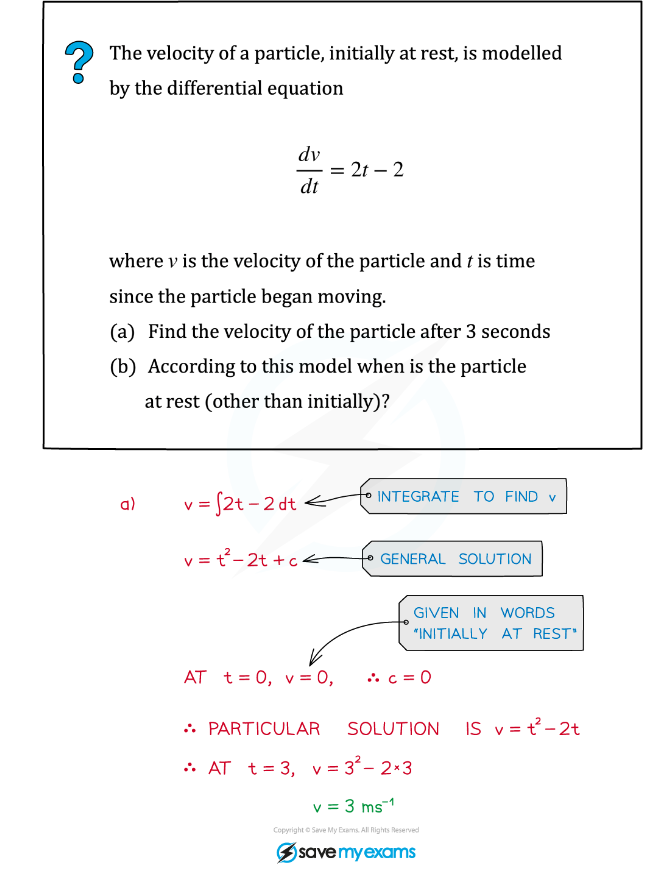
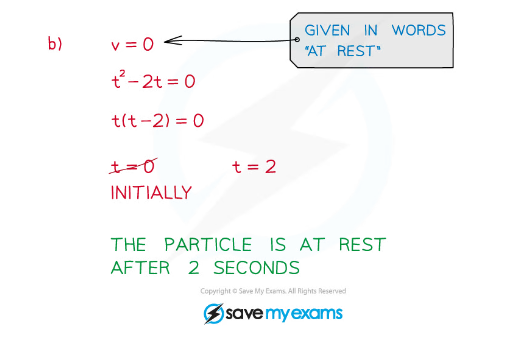
Worked Example
转载自savemyexams

在线登记
最新发布
© 2025. All Rights Reserved. 沪ICP备2023009024号-1