Edexcel A Level Maths: Pure:复习笔记2.12.1 Modelling with Functions
Modelling with Functions
What is a mathematical model?
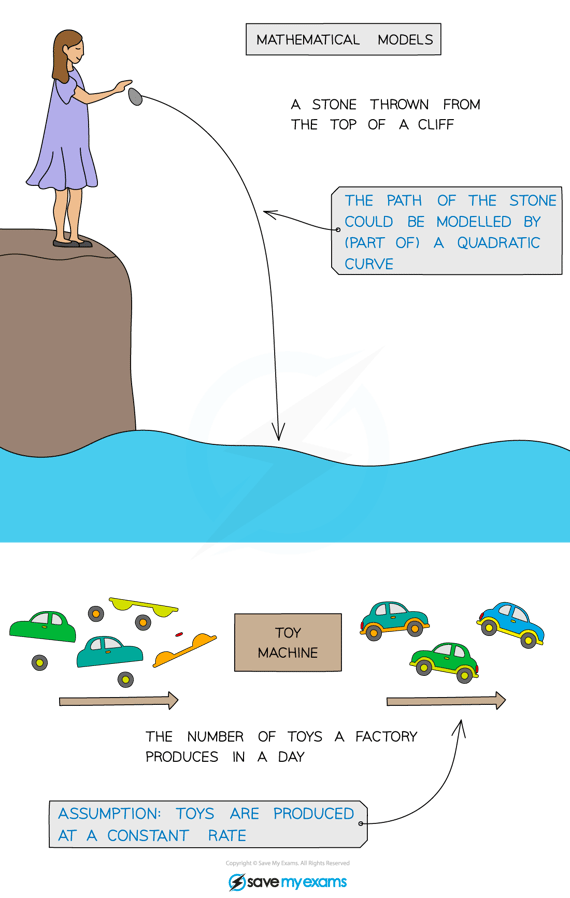
- A mathematical model simplifies a real-world situation so it can be described using mathematics which can then be used to make predictions
- The path a stone will take if thrown from the top of a cliff
- The number of toys a factory can produce in a day
- Assumptions about the situation are made in order to simplify the mathematics
- Air resistance on the stone can be ignored
- The machines/people at the factory produce toys at a constant rate
- Models can be refined (improved) if further information is available or the model is compared to real-world data
- The mass of the stone needs to be considered
- 30-minutes downtime per day is allowed for machine repairs/maintenance
How do I solve modelling problems?
- There will be no one-size-fits-all step-by-step guide to solving modelling questions
- A combination of skills and problem-solving skills will be needed
Exam Tip
- Read – and re-read – the question carefully.Try to “get involved” in the context of the question – however boring it may be!
- Imagine what happens to a stone as you throw it from a cliff
- What would it be like to manage a toy factory?
- Draw a diagram – sketch a graph of the function being used as the model.
- If you are completely stuck try “doing something” with the maths. For example, if there’s a quadratic function – sketch it, factorise it, solve it. Or if there’s a polynomial – use factor theorem and algebraic division to factorise it.
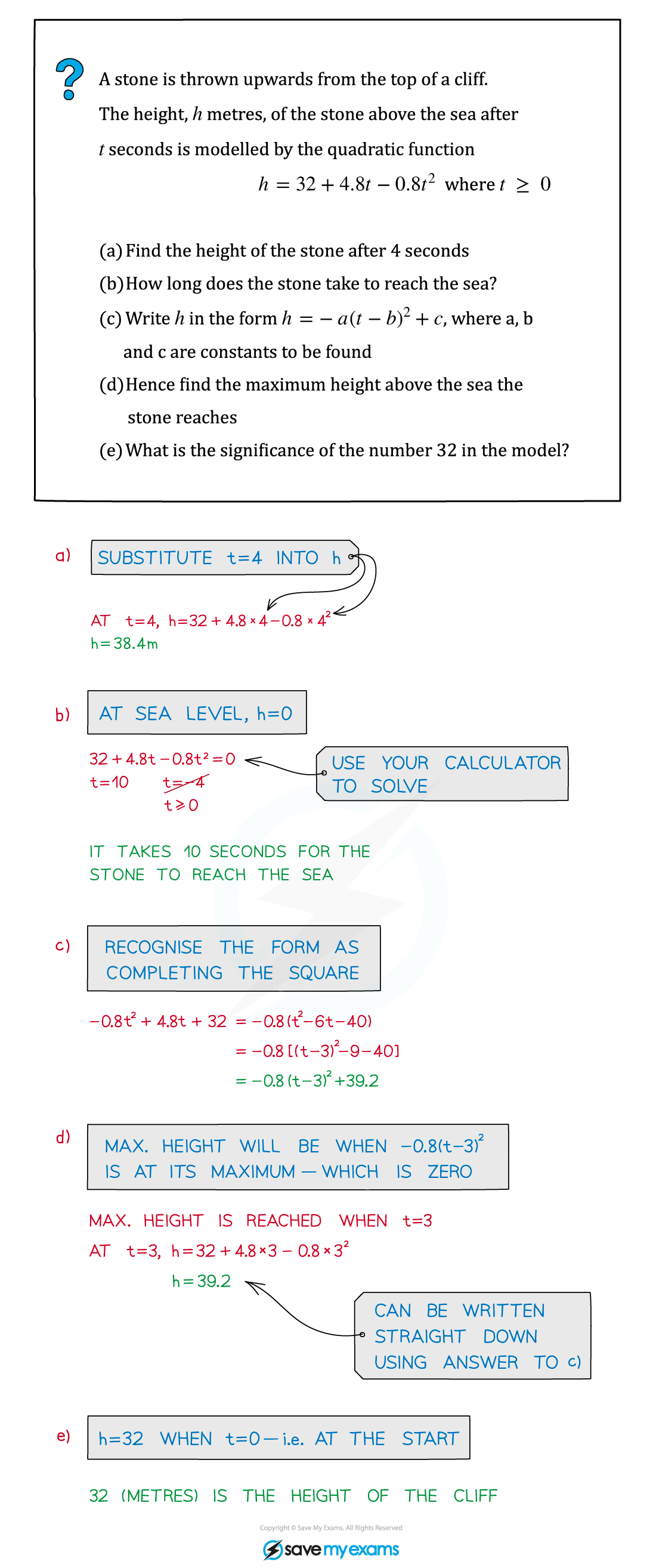
Worked Example
转载自savemyexams
国际竞赛真题资料-点击免费领取！
美高学分项目重磅来袭！立即了解
AMC8备考管家服务全新上线！
