- 翰林提供学术活动、国际课程、科研项目一站式留学背景提升服务!
- 400 888 0080
Edexcel A Level Further Maths: Core Pure:复习笔记5.2.3 Integrating with Partial Fractions
Integrating with Partial Fractions
What is meant by partial fractions with quadratic denominators?
- For linear denominators the denominator of the original fraction can be factorised such that the denominator becomes a product of linear terms of the form
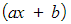
- With squared linear denominators, the same applies, except that some (usually just one) of the factors on the denominator may be squared, i.e.
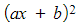
- In both the above cases it can be shown that the numerators of each of the partial fractions will be a constant
- For this course, quadratic denominators refer to fractions that contain a quadratic factor (that cannot be factorised) on the denominator
- the denominator of the quadratic partial fraction will be of the form
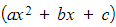 ; very often
; very often  leaving it as
leaving it as 
- the numerator of the quadratic partial fraction could be of linear form,
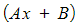
- the denominator of the quadratic partial fraction will be of the form
How do I find partial fractions involving quadratic denominators?
- STEP 1 Factorise the denominator as far as possible (if not already done so)
-
- Sometimes the numerator can be factorised too
- STEP 2 Split the fraction into a sum with
- the linear denominator having an (unknown) constant numerator
- the quadratic denominator having an (unknown) linear numerator
- STEP 3 Multiply through by the denominator to eliminate fractions
- STEP 4 Substitute values into the identity and solve for the unknown constants
- Use the root of the linear factor as a value of
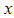 to find one of the unknowns
to find one of the unknowns - Use any two values for
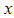 to form two equations to solve simultaneously
to form two equations to solve simultaneously
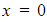 is a good choice if this has not already been used with the linear factor
is a good choice if this has not already been used with the linear factor
- Use the root of the linear factor as a value of
- STEP 5 Write the original as partial fraction
How do I integrate the fraction with the quadratic denominator?
- The quadratic denominator will be of the form
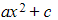
- If it is not then you can get it to look like this by completing the square
- Split into to fraction
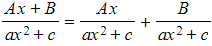
- Integrate
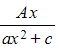 using logarithms to get
using logarithms to get 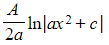
- Integrate
 using the formula booklet or using a trigonometric or hyperbolic substitution
using the formula booklet or using a trigonometric or hyperbolic substitution
- If a and c have the same sign then use
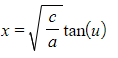
- If a and c have different signs then use
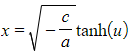
- Or in this case you can factorise using surds and then use partial fractions
- If a and c have the same sign then use
Worked Example
Find 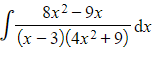


转载自savemyexams

早鸟钜惠!翰林2025暑期班课上线

最新发布
© 2025. All Rights Reserved. 沪ICP备2023009024号-1








