CIE A Level Physics复习笔记17.1.3 Calculating Acceleration & Displacement in SHM

• The acceleration of an object oscillating in simple harmonic motion is:

a = −⍵²x

• Where:
  • a = acceleration (m s^-2)
  • ⍵ = angular frequency (rad s^-1)
  • x = displacement (m)
• This is used to find the acceleration of an object in SHM with a particular angular frequency ⍵ at a specific displacement x
• The equation demonstrates:
  • The acceleration reaches its maximum value when the displacement is at a maximum ie. x = x₀ (amplitude)
  • The minus sign shows that when the object is displacement to the right, the direction of the acceleration is to the left

The acceleration of an object in SHM is directly proportional to the negative displacement

• The graph of acceleration against displacement is a straight line through the origin sloping downwards (similar to y = − x)
• Key features of the graph:
  • The gradient is equal to − ⍵²
  • The maximum and minimum displacement x values are the amplitudes −x₀ and +x₀
• A solution to the SHM acceleration equation is the displacement equation:

x = x₀sin(⍵t)

• Where:
  • x = displacement (m)
  • x₀ = amplitude (m)
  • t = time (s)

• This equation can be used to find the position of an object in SHM with a particular angular frequency and amplitude at a moment in time
  • Note: This version of the equation is only relevant when an object begins oscillating from the equilibrium position (x = 0 at t = 0)
• The displacement will be at its maximum when sin(⍵t) equals 1 or − 1, when x = x₀
• If an object is oscillating from its amplitude position (x = x₀ or x = − x₀ at t = 0) then the displacement equation will be:

x = x₀cos(⍵t)

• This is because the cosine graph starts at a maximum, whilst the sine graph starts at 0

These two graphs represent the same SHM. The difference is the starting position

Step 1: Write down the SHM displacement equation

• • Since the mass is released at t = 0 at its maximum displacement, the displacement equation will be with the cosine function:

x = x₀ cos(⍵t)

Step 2: Calculate angular frequency

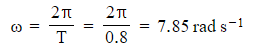• • Remember to use the value of the time period given, not the time where you are calculating the displacement from

Step 3: Substitute values into the displacement equation

x = 4.3 cos (7.85 × 0.3) = –3.0369… = –3.0 cm (2 s.f)

• • Make sure the calculator is in radians mode
  • The negative value means the mass is 3.0 cm on the opposite side of the equilibrium position to where it started (3.0 cm above it)