- 翰林提供学术活动、国际课程、科研项目一站式留学背景提升服务!
- 400 888 0080
CIE A Level Physics复习笔记13.1.3 Circular Orbits in Gravitational Fields
Circular Orbits in Gravitational Fields
- Since most planets and satellites have a near circular orbit, the gravitational force FG between the sun or another planet provides the centripetal force needed to stay in an orbit
- Both the gravitational force and centripetal force are perpendicular to the direction of travel of the planet
- Consider a satellite with mass m orbiting Earth with mass M at a distance r from the centre travelling with linear speed v
![]()
- Equating the gravitational force to the centripetal force for a planet or satellite in orbit gives:
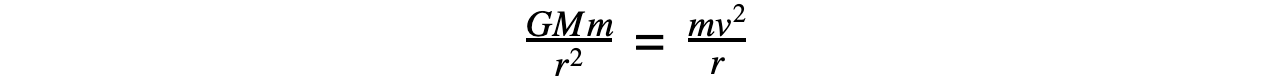
- The mass of the satellite m will cancel out on both sides to give:
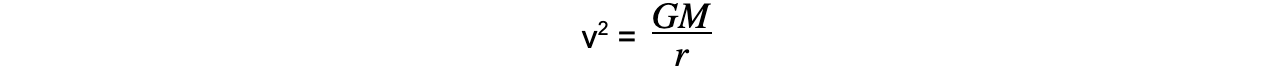
- This means that all satellites, whatever their mass, will travel at the same speed v in a particular orbit radius r
- Recall that since the direction of a planet orbiting in circular motion is constantly changing, it has centripetal acceleration

A satellite in orbit around the Earth travels in circular motion
Kepler’s Third Law of Planetary Motion
- For the orbital time period T to travel the circumference of the orbit 2πr, the linear speed v can be written as
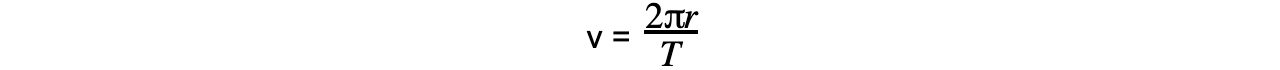
- This is a result of the well-known equation, speed = distance / time
- Substituting the value of the linear speed v into the above equation:
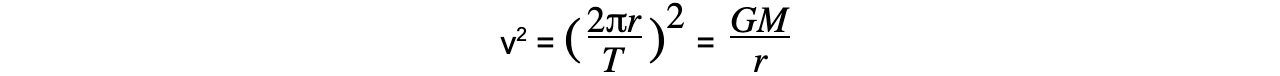
- Rearranging leads to Kepler’s third law equation:
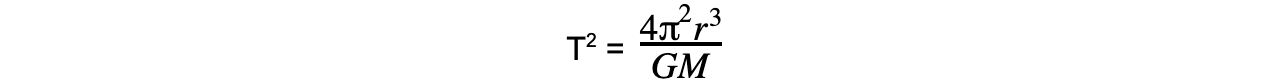
- The equation shows that the orbital period T is related to the radius r of the orbit. This is known as Kepler’s third law:
For planets or satellites in a circular orbit about the same central body, the square of the time period is proportional to the cube of the radius of the orbit
- Kepler’s third law can be summarised as:
![]()
Maths Tip
- The ∝ symbol means ‘proportional to’
- Find out more about proportional relationships between two variables in the “proportional relationships ” section of the A Level Maths revision notes
Worked Example
A binary star system constant of two stars orbiting about a fixed point B.The star of mass M1 has a circular orbit of radius R1 and mass M2 has a radius of R2. Both have linear speed v and an angular speed ⍵ about B.

State the following formula, in terms of G, M2, R1 and R2
(i) The angular speed ⍵ of M1
(ii) The time period T for each star in terms of angular speed ⍵
(1) The angular speed of ⍵ of M1
Step 1: Equating the centripetal force of mass M1 to the gravitational force between M1 and M2

Step 2: M1 cancels on both sides

Step 3: Rearrange for angular velocity ⍵
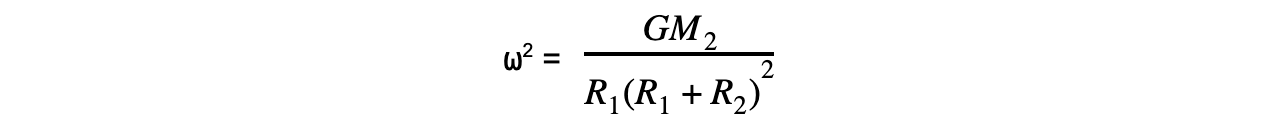
Step 4: Square root both sides
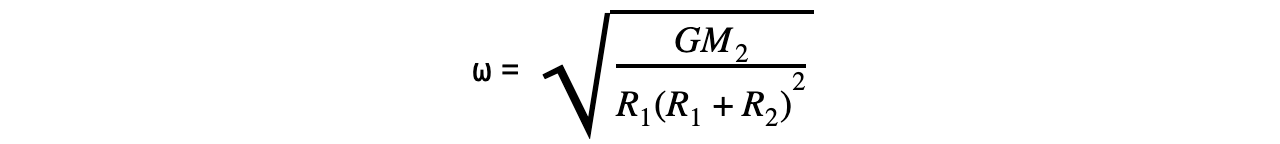
(2) The time period T for each star in terms of angular speed ⍵
Step 1: Angular speed equation with time period T
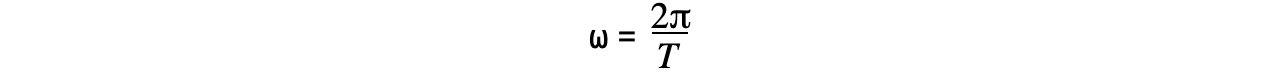
Step 2: Rearrange for T
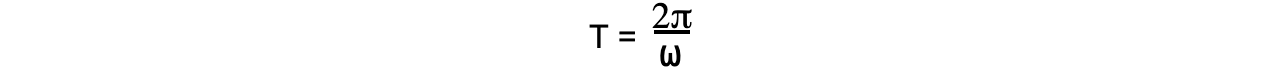
Step 3: Substitute in ⍵
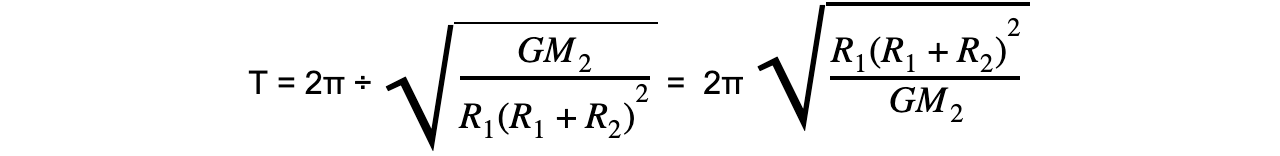
Exam Tip
Many of the calculations in the Gravitation questions depend on the equations for circular motion. Be sure to revisit these and understand how to use them!
转载自savemyexams


最新发布
© 2025. All Rights Reserved. 沪ICP备2023009024号-1








