AQA A Level Maths: Pure复习笔记7.3.1 First Principles Differentiation - Trigonometry

How do I derive the derivatives of trigonometric functions from first principles?

• Recall that for a function f(x) the definition of the derivative from first principles (see First Principles Differentiation) is: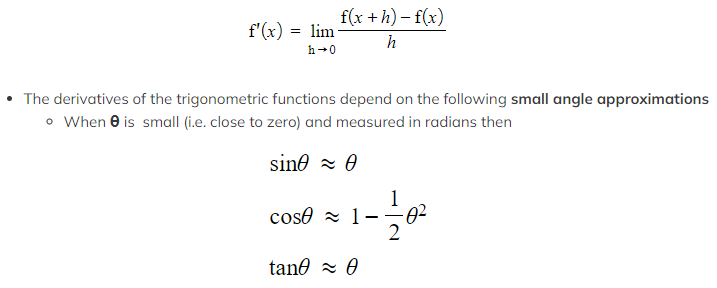The small angle approximations allow us to produce the following intermediate limit results:

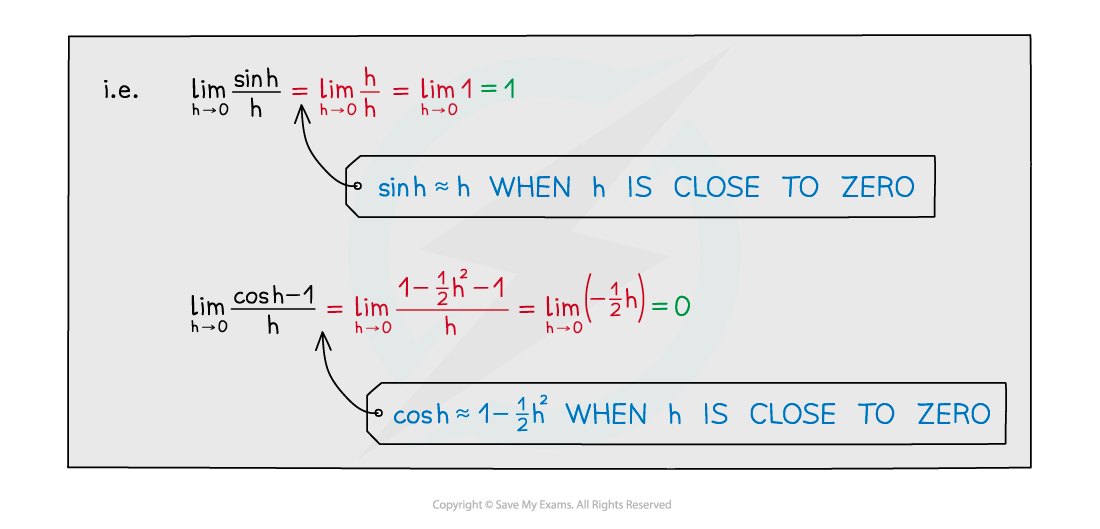
• And those intermediate results allow us to find the derivatives of sin and cos

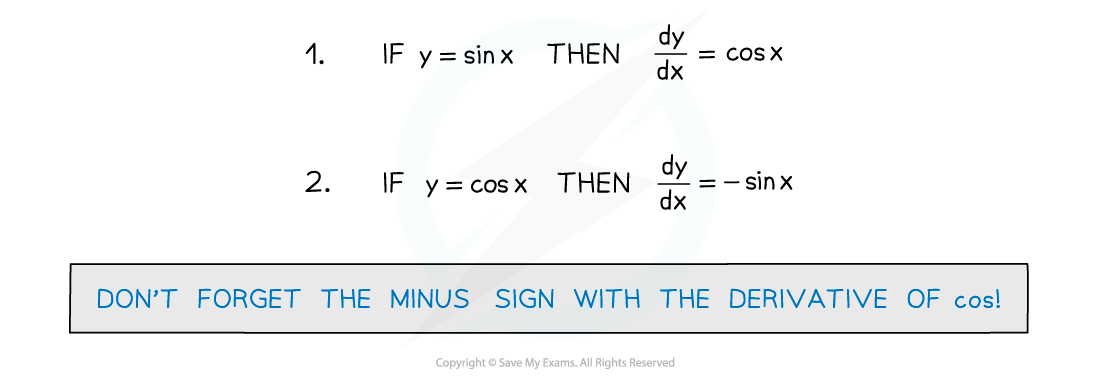
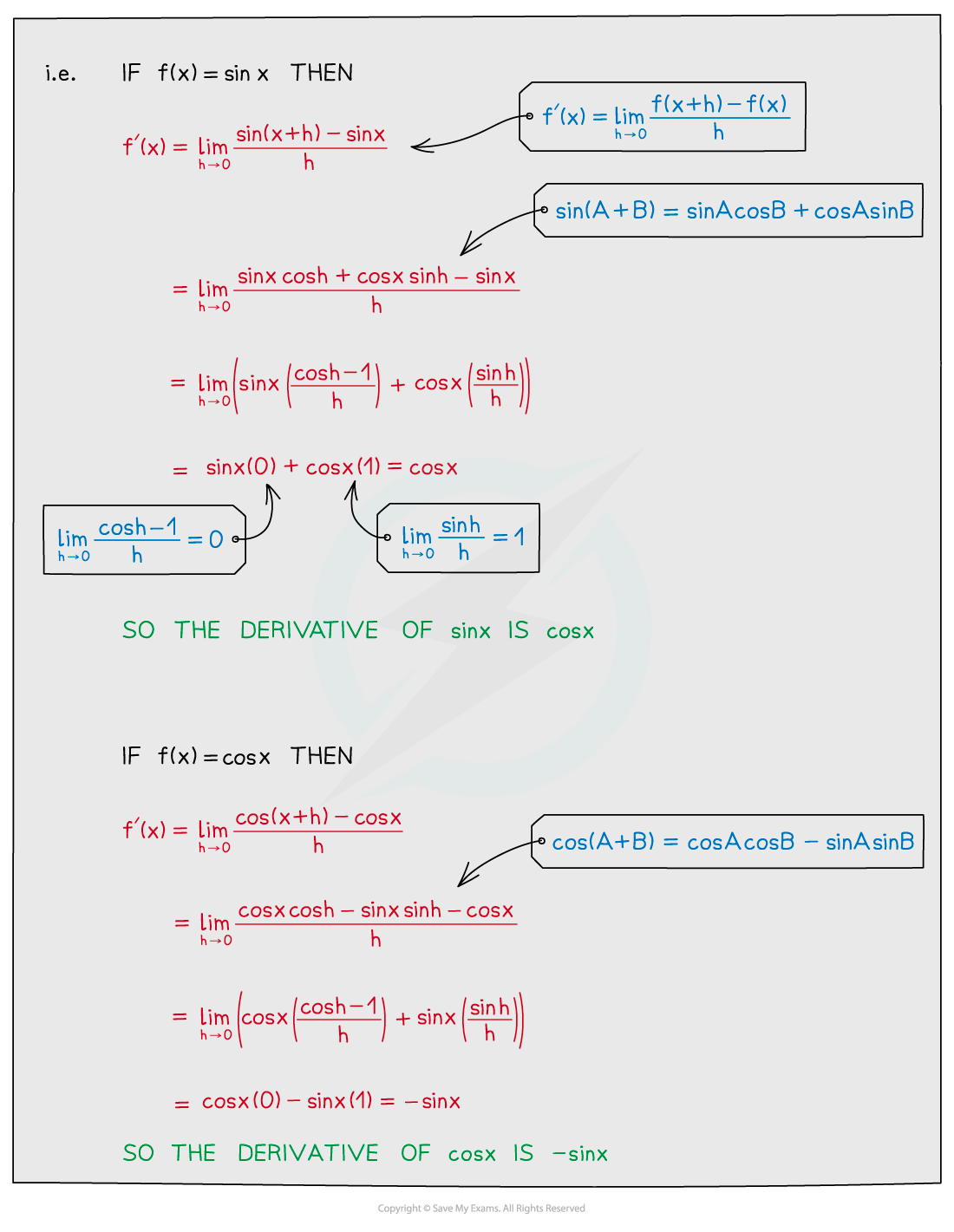
Derivatives of other trigonometric functions

• The derivative of tan is given by the following formula:

• The easiest way to derive this is to use the quotient rule and the derivatives of sin and cos
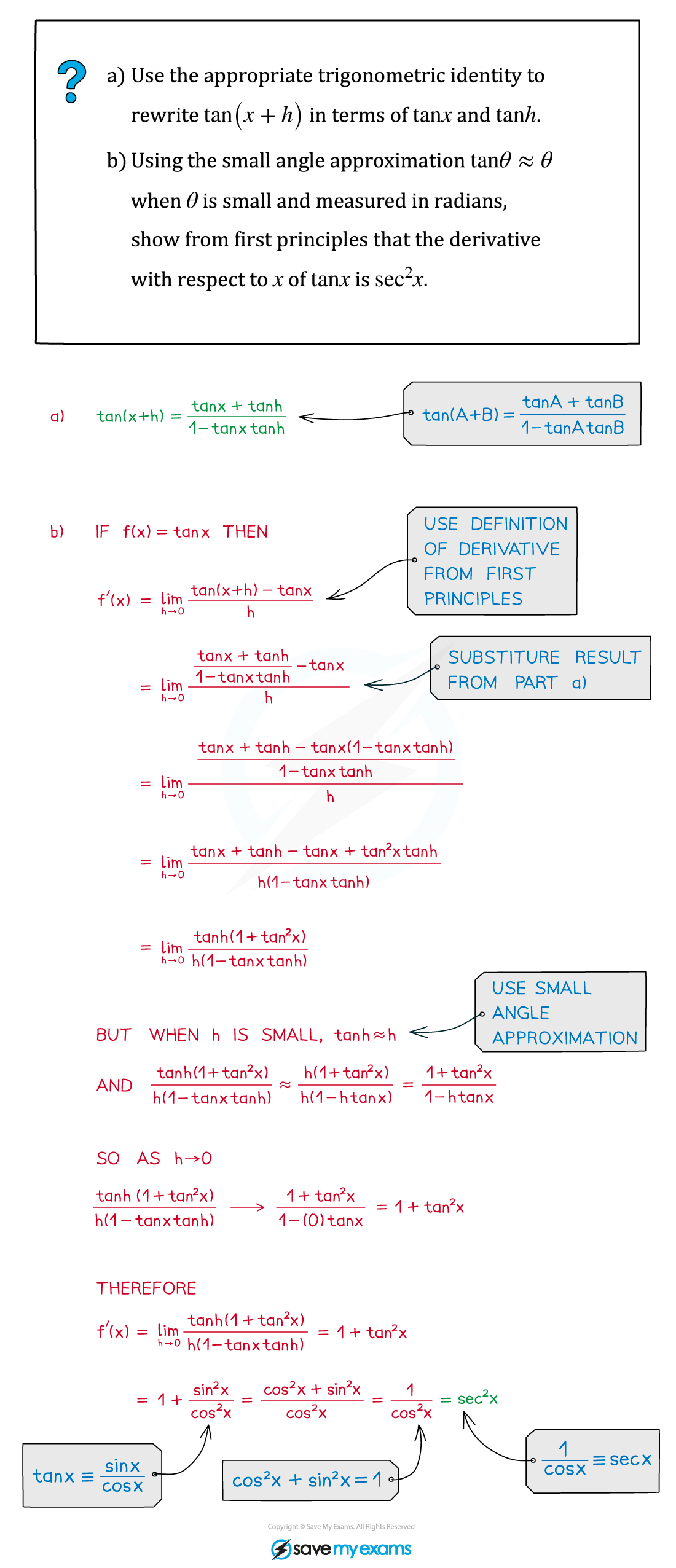
• But it can also be derived from first principles using the small angle approximation for tan (see the Worked Example)
• The general formulae for the derivatives of the trigonometric functions are:
• These formulae follow from combining the derivatives of the three basic functions with the chain rule, but they are worth knowing on their own