AQA A Level Maths: Pure复习笔记5.1.2 Right-Angled Triangles
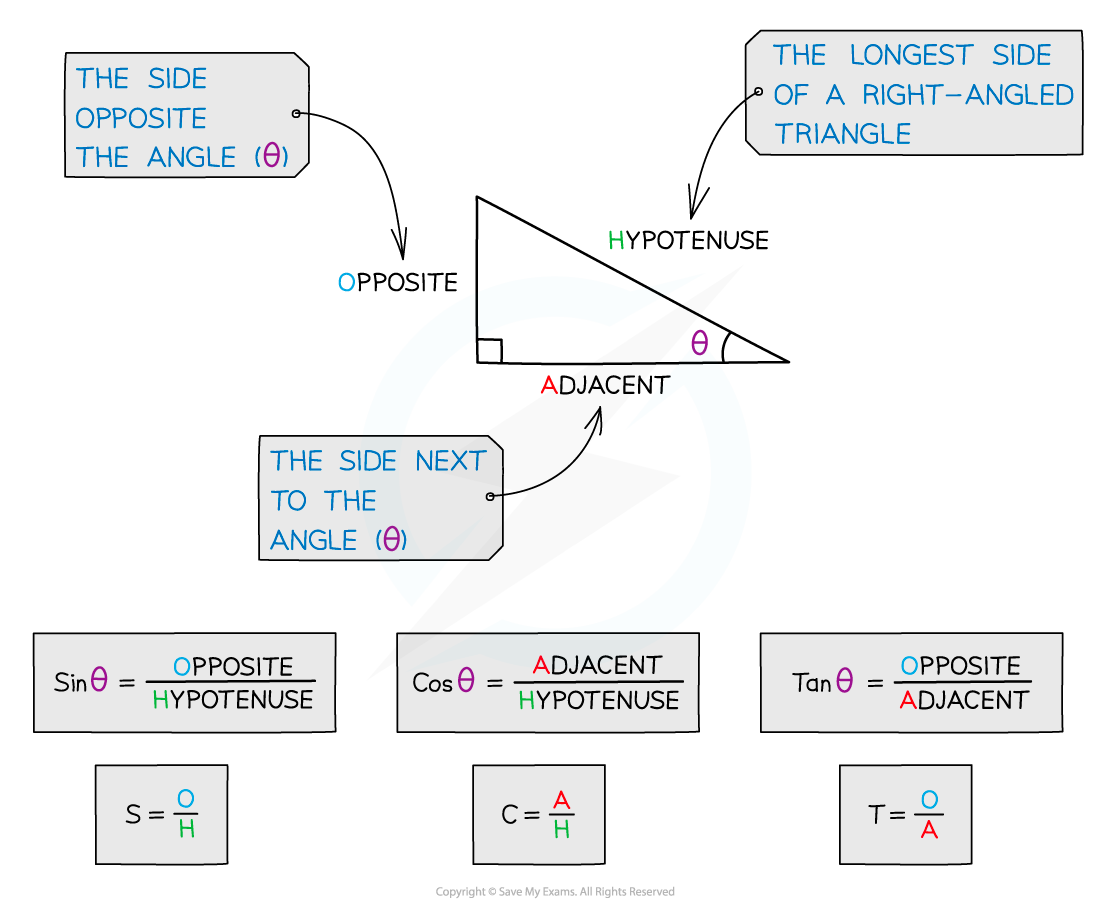
Right-Angled Triangles
What is SOHCAHTOA?
- SOH, CAH, and TOA are an easy way to remember the three basic trigonometry ratios
- They show the relationship between an angle and the different sides of a right-angled triangle which we can label Hypotenuse, Adjacent and Opposite
- We use them to find missing angles and sides for right‑angled triangles
- Hypotenuse is the longest side of a right-angled triangle
- Adjacent is the side next to the angle you are working with (in between the angle and the right angle)
- Opposite is the side opposite the angle you are working with
- We use the inverse trig functions to find missing angles
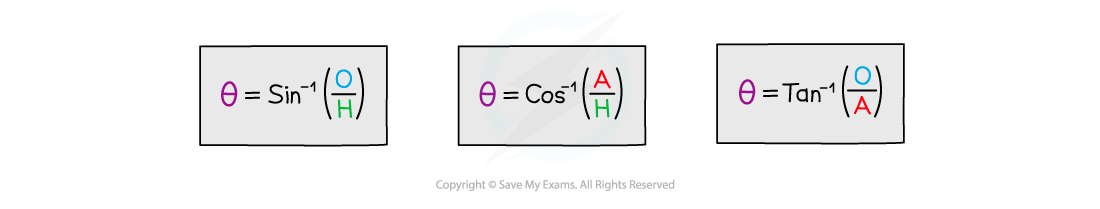
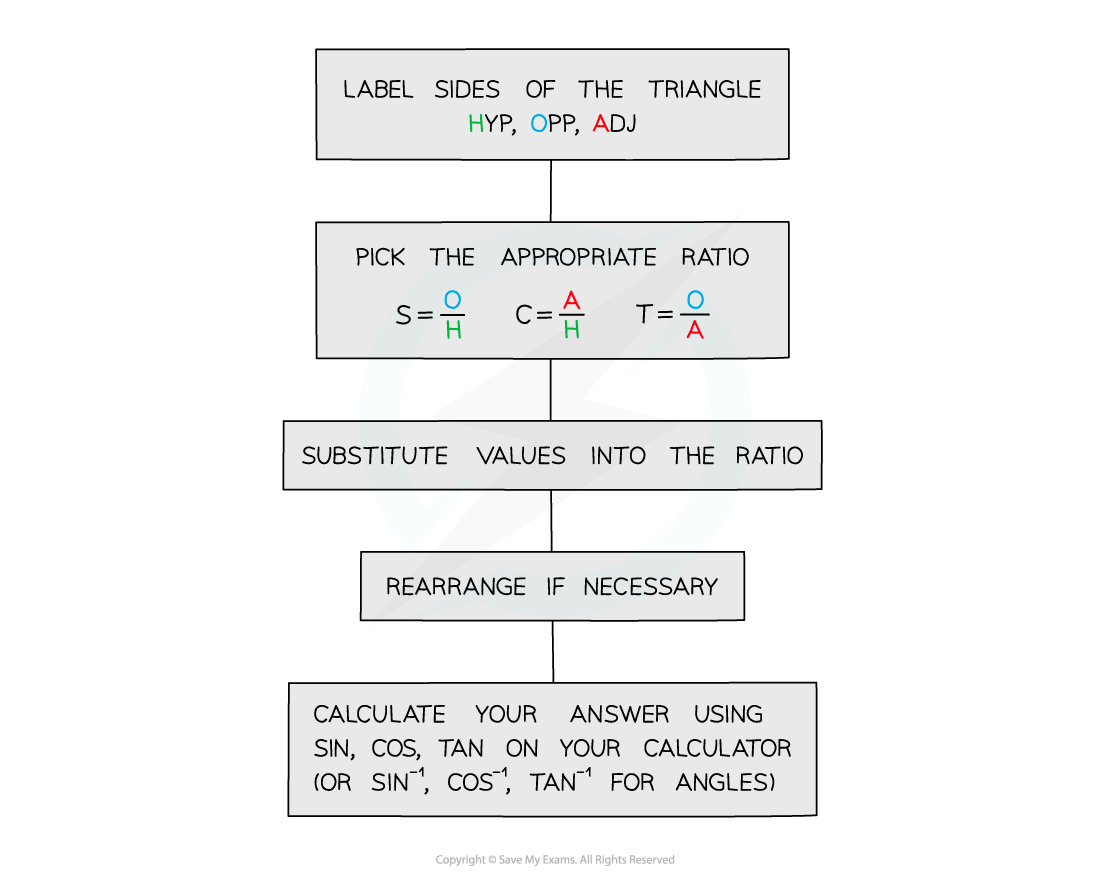
How do I use SOHCAHTOA?
Exam Tip
- Remember SOHCAHTOA are only for calculating missing sides and angles for right‑angled triangles
- For non-right‑angled triangles you will need to use the Sine or Cosine rules.
- Make sure your calculator is in degree mode (D).
- Always check your answers are sensible…
- Should my answer be longer or shorter than the sides I have already i.e. is it the hypotenuse?
- Is my angle sensible…
- Have I remembered to use -1?
- Check if the question asks you to round your answer.
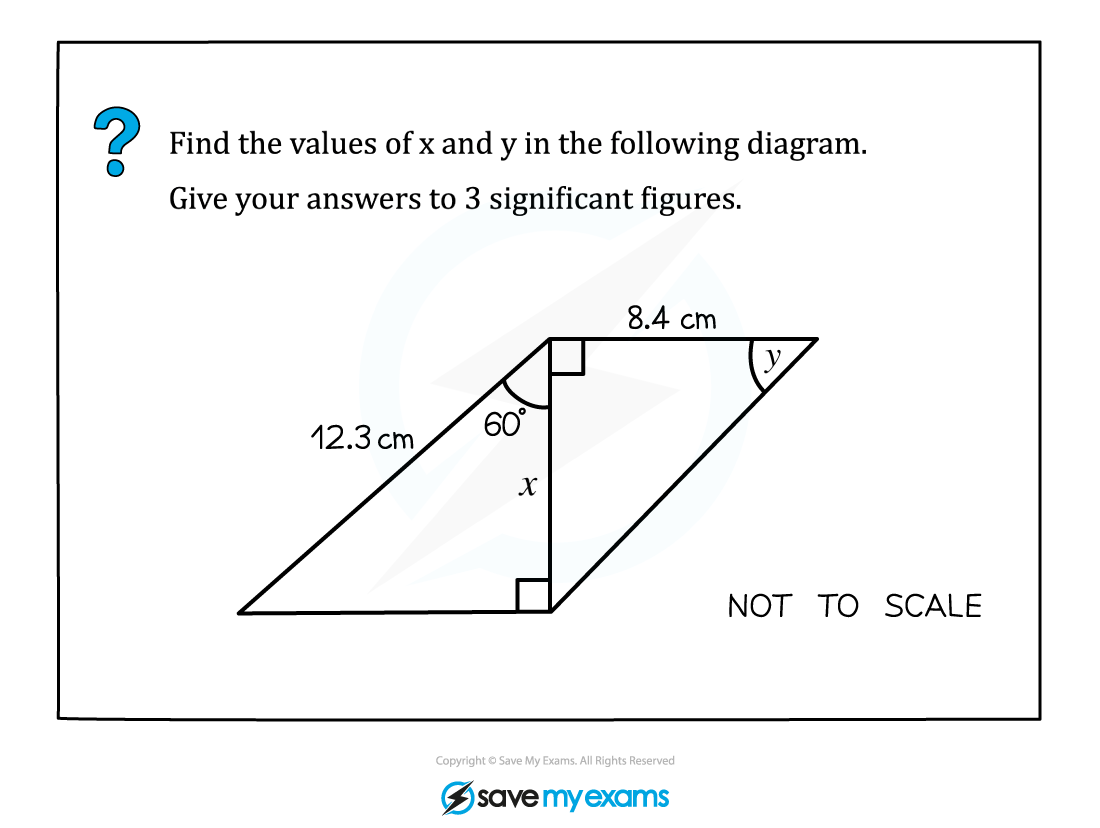
Worked Example
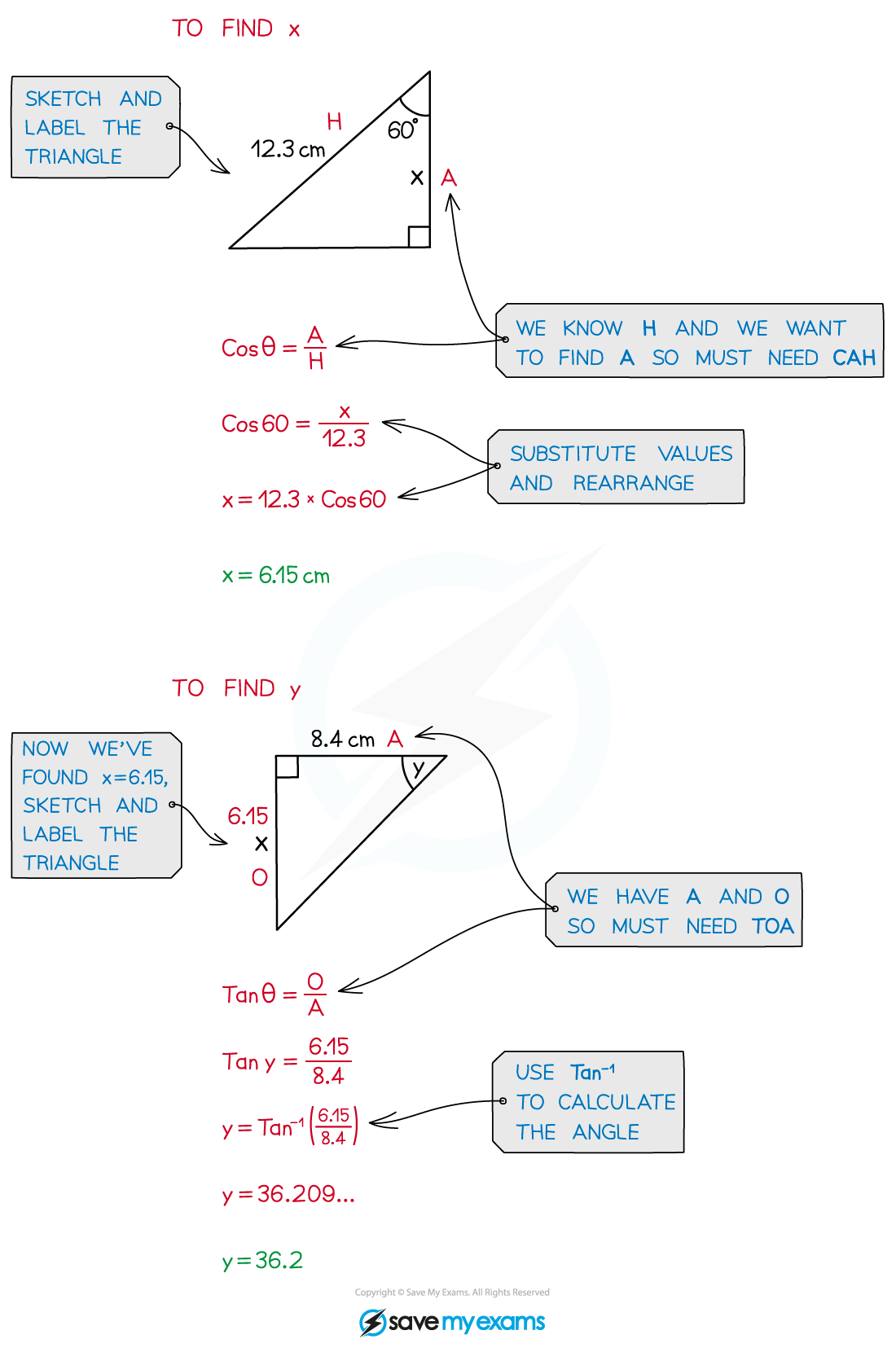
转载自savemyexams
国际竞赛真题资料-点击免费领取！
早鸟钜惠！翰林2025暑期班课上线
美高学分项目重磅来袭！立即了解
