IB DP Physics: SL复习笔记6.1.4 Applications of Circular Motion

Horizontal Circular Motion

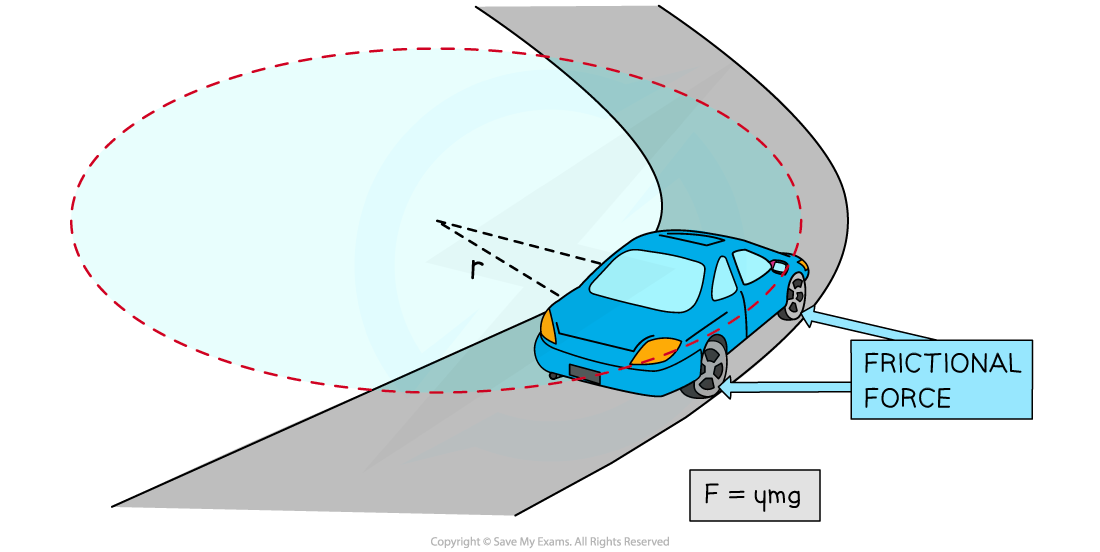
• An example of horizontal circular motion is a vehicle driving on a curved road
• The forces acting on the vehicle are:
  • The friction between the tyres and the road
  • The weight of the vehicle downwards
• In this case, the centripetal force required to make this turn is provided by the frictional force
  • This is because the force of friction acts towards the centre of the circular path
• Since the centripetal force is provided by the force of friction, the following equation can be written:

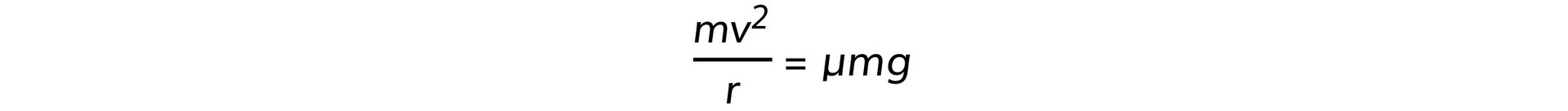
• Where:
  • m = mass of the vehicle (kg)
  • v = speed of the vehicle (m s–1)
  • r = radius of the circular path (m)
  • μ = static coefficient of friction
  • g = acceleration due to gravity (m s–2)

• Rearranging this equation for v gives:

v2 = μgr

• This expression gives the maximum speed at which the vehicle can travel around the curved road without skidding
  • If the speed exceeds this, then the vehicle is likely to skid
  • This is because the centripetal force required to keep the car in a circular path could not be provided by friction, as it would be too large

• Therefore, in order for a vehicle to avoid skidding on a curved road of radius r, its speed must satisfy the equation

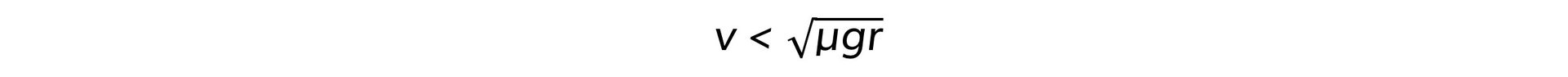
Banking

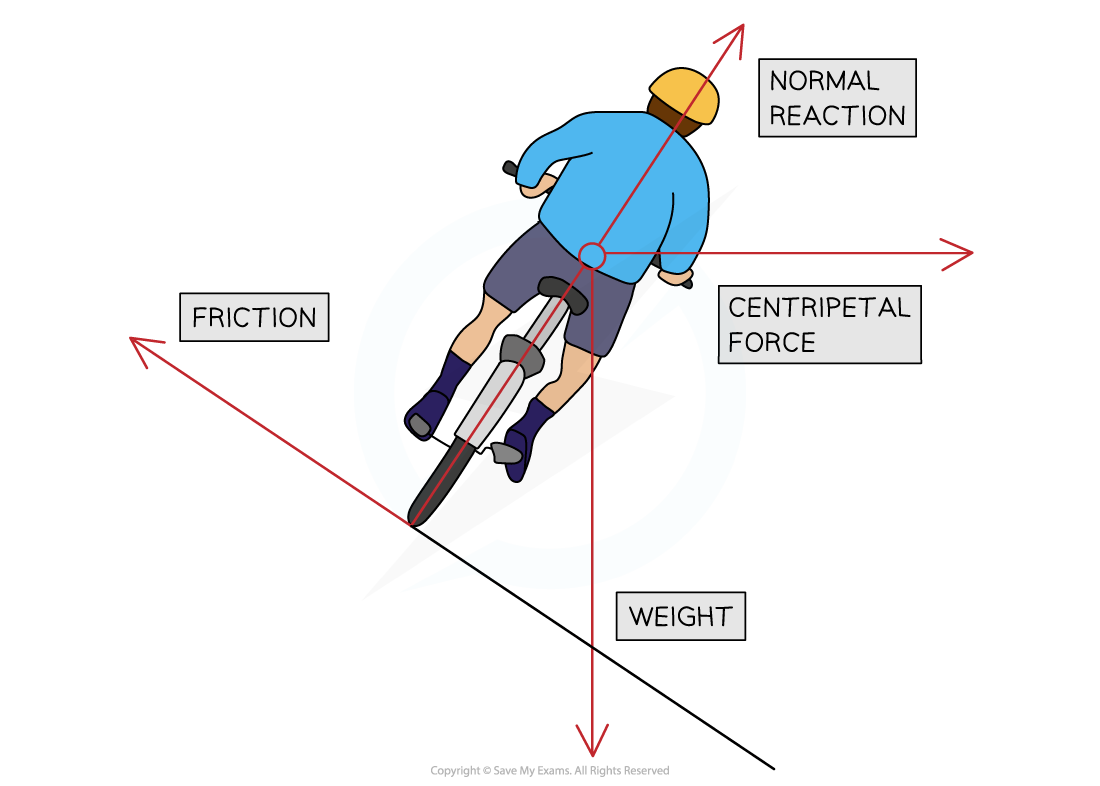
• A banked road, or track, is a curved surface where the outer edge is raised higher than the inner edge
  • The purpose of this is to make it safer for vehicles to travel on the curved road, or track, at a reasonable speed without skidding
• When a road is banked, the centripetal force no longer depends on the friction between the tyres and the road
• Instead, the centripetal force depends solely on the normal force and the weight of the vehicle

Vertical Circular Motion

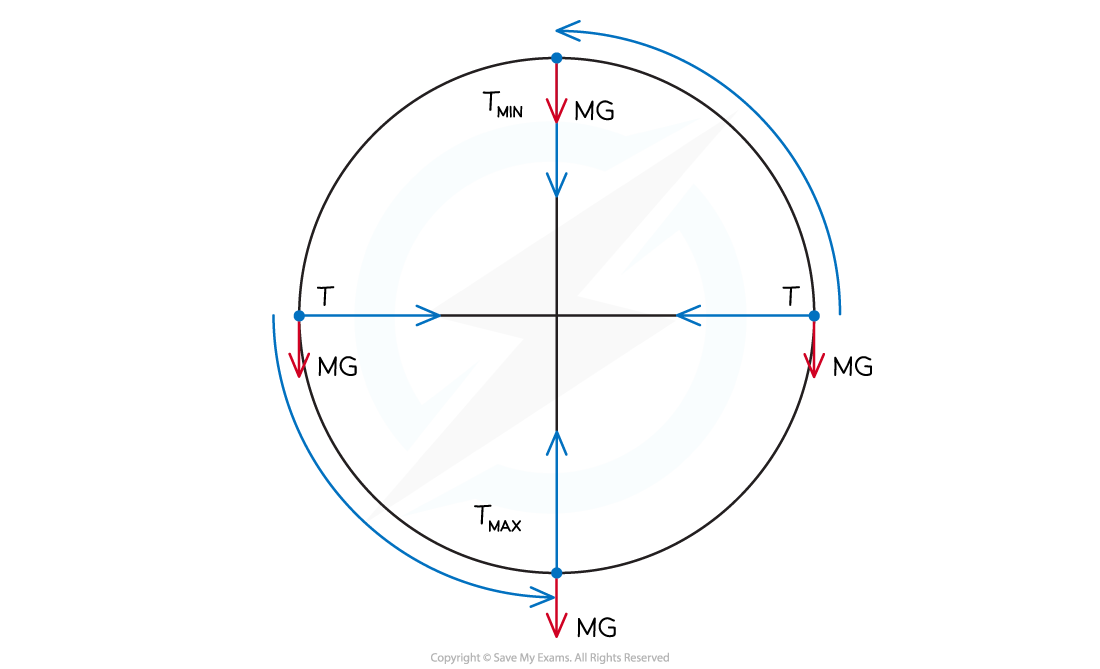
• An example of vertical circular motion is swinging a ball on a string in a vertical circle
• The forces acting on the ball are:
  • The tension in the string
  • The weight of the ball downwards

• As the ball moves around the circle, the direction of the tension will change continuously
• The magnitude of the tension will also vary continuously, reaching a maximum value at the bottom and a minimum value at the top
  • This is because the direction of the weight of the ball never changes, so the resultant force will vary depending on the position of the ball in the circle

• At the bottom of the circle, the tension must overcome the weight, this can be written as:

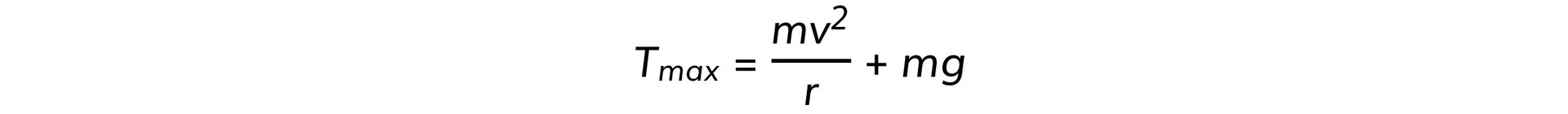
• As a result, the acceleration, and hence, the speed of the ball will be slower at the top
• At the top of the circle, the tension and weight act in the same direction, this can be written as:

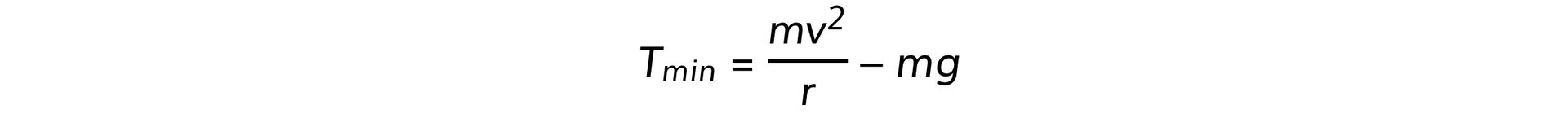
• As a result, the acceleration, and hence, the speed of the ball will be faster at the bottom