IB DP Physics: SL复习笔记4.5.4 Harmonics

• Stationary waves can have different wave patterns, known as harmonics
  • These depend on the frequency of the vibration and the boundary conditions (i.e. fixed and/or free ends)

• The harmonics are the only frequencies and wavelengths that will form standing waves on strings or in pipes

Harmonics on Strings

• The boundary condition is that both ends are fixed
• The simplest wave pattern is a single loop made up of two nodes (i.e. the two fixed ends) and an antinode
  • This is called the first harmonic
  • The wavelength of this harmonic is λ₁ = 2L
  • Using the wave equation, the frequency is f₁ = v/2L, where v is the wave speed of the travelling waves on the string (i.e. the incident wave and the reflected wave)

• As the vibrating frequency increases, more complex patterns arise
  • The second harmonic has three nodes and two antinodes
  • The third harmonic has four nodes and three antinodes

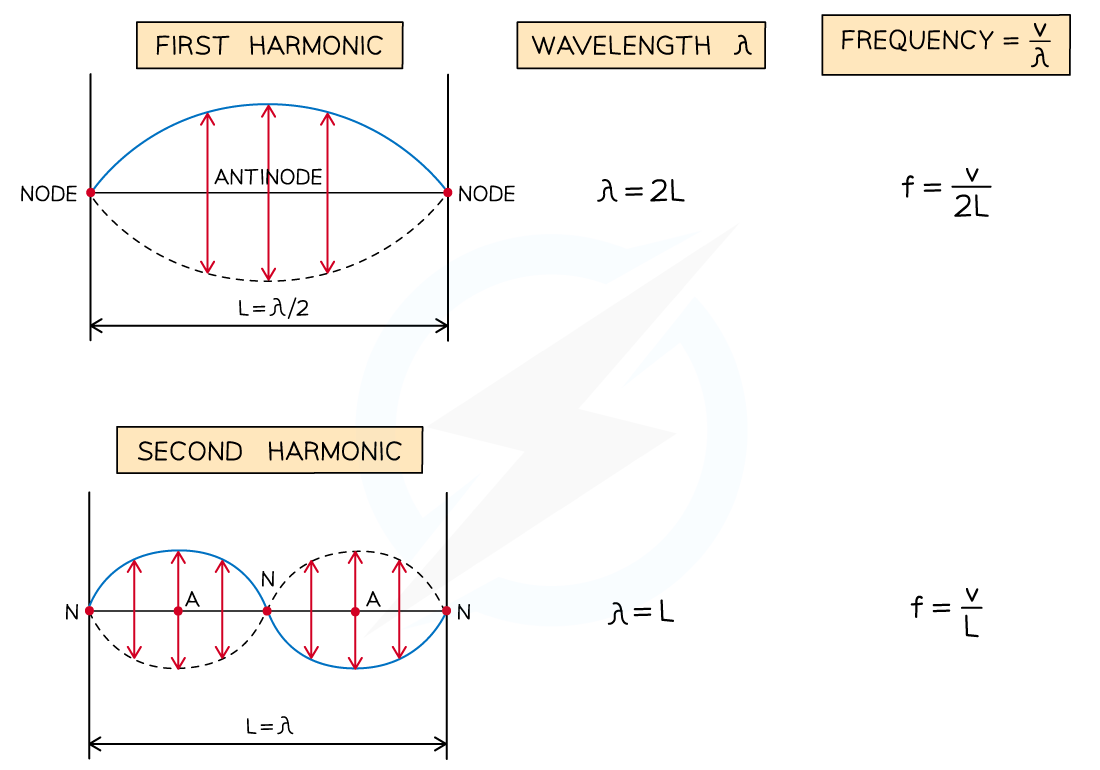
Diagram showing the first three harmonics on a stretched string fixed at both ends

• The nth harmonic will have (n + 1) nodes and n antinodes
• The general expression for the wavelength of the nth harmonic on a string that is fixed at both ends is:

• Where:
  • λn = wavelength in metres (m)
  • L = length of the string in metres (m)
  • n = integer number greater than zero - i.e. 1, 2, 3...
• Knowing the wavelength λn of the standing wave and the speed v of the travelling waves (i.e. incident and reflected), the natural frequency fn of any harmonic can be calculated using the wave equation v = fλ_n, so that:

Harmonics in Pipes

• The boundary conditions vary, since pipes can have:
  • two open ends
  • only one open end

• For a pipe that is open at both ends:
  • The simplest wave pattern is one central node and two antinodes
  • The second harmonic consists of two nodes and three antinodes
  • The nth harmonic will have (n + 1) antinodes and n nodes
  • The expression for the wavelength of the nth harmonic in a pipe of length L is the same as that given above for nth harmonic on a string

Diagram showing the first five harmonics in a pipe open at both ends

• For a pipe that is open at one end:
  • The lowest harmonic is a "half-loop" with one node and one antinode
  • The next possible harmonic will have two nodes and two antinodes
    • This is the third harmonic, not the second one
    • Since only odd harmonics can exist under this boundary condition

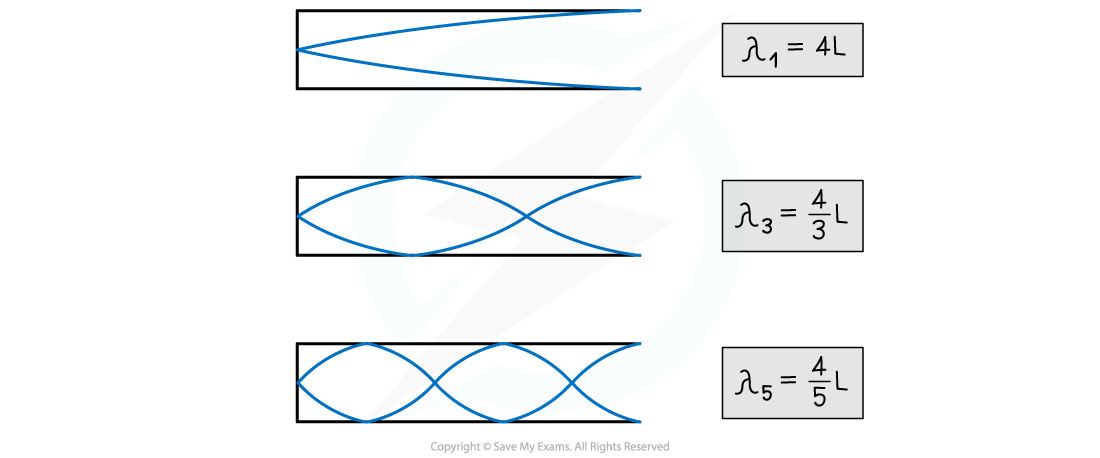
Diagram showing the first three possible harmonics in a pipe open at one end. Only the odd harmonics can form in this case

• • The expression for the wavelength of the nth harmonic in a pipe of length L is:

• • Where this time, n is an odd number - i.e. 1, 3, 5...

• Under both boundary conditions, the natural frequencies are once again calculated from the wavelength of the standing wave and the speed v of the travelling waves using the wave equation

Step 1: Write down the known quantities

• • L = 100 cm = 1.00 m
  • v = 250 m s^–1
  • fn = 15 kHz = 15000 Hz

Note the conversions:

• • The length must be converted from centimetres (cm) into metres (m)
  • The frequency must be converted from kilohertz (kHz) into hertz (Hz)

Step 2: Write down the equation for the frequency of the nth harmonic

Step 3: Rearrange the above equation to calculate the number n of the maximum harmonic detectable by the person

Step 4: Substitute the numbers into the above equation

n = 120

The person can hear up to the 120th harmonic