IB DP Physics: SL复习笔记2.2.5 Applying Newton’s Laws of Motion

• Newton's laws of motion are strong tools to understand the motion of objects with forces acting upon them
• Below are two worked examples demonstrating different situations involving the application of Newton's laws

Step 1: Consider the whole of the system

• • Together the 1 kg and 4 kg blocks are both being pulled along by the 100 N force (since the rope is tight)
  • This is a frictionless flat surface, therefore the only forces in the system are the pulling force and the tension force(s) in the rope
  • Therefore the acceleration can be found using Newton's second law

Step 2: Find the acceleration

• • Using Newton's second law:

F = m × a

• • Rearrange for a

a = F ÷ m

a = 100 ÷ 5 = 20 m s–2  to the left

Step 3: Examine the 4 kg mass only

• • Since the system is moving with an acceleration of 20 m s–2  to the left, the force on only the 4 kg mass can be found

F = m × a

F = 4 × 20 = 80 N to the left

• • The only force acting on the 4 kg mass is the rope and therefore tension force
  • This means there is 20 N pulling force on the 1 kg block only

Step 4: State your answer

• • Since acceleration, a = 20 m s–2  to the left and the tension force = 80 N to the left
  • Therefore, the answer is option D

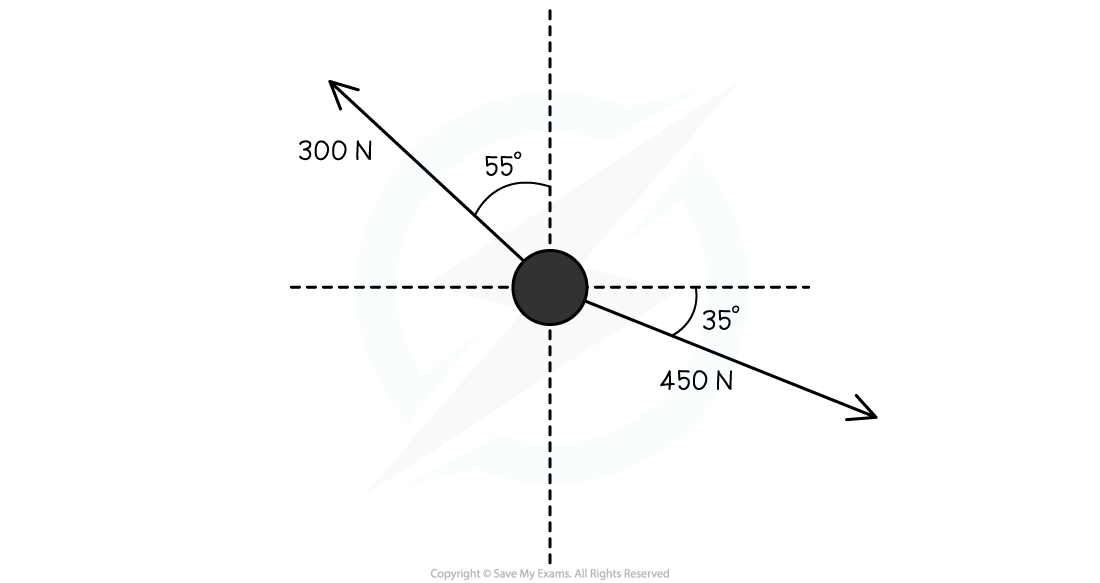
Step 1: Recall Newton's first law

• • Newton's First Law states:

A body will remain at rest or move with constant velocity unless acted on by a resultant force

• • Therefore, for this object to remain stationary, the resultant force must have a magnitude of 0 Newtons

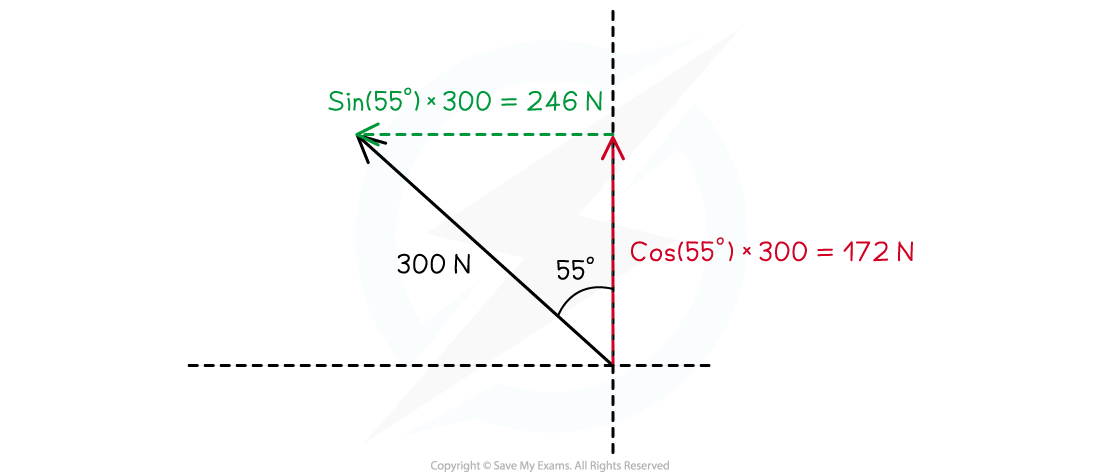
Step 2: Resolve the 300 N force into its horizontal and vertical components

• • The horizontal component can be resolved from:

sin(55°) × 300 = 246 N

• • This is directed to the left

• • The vertical component can be resolved from:

cos(55°) × 300 = 172 N

• • This is in an upwards direction

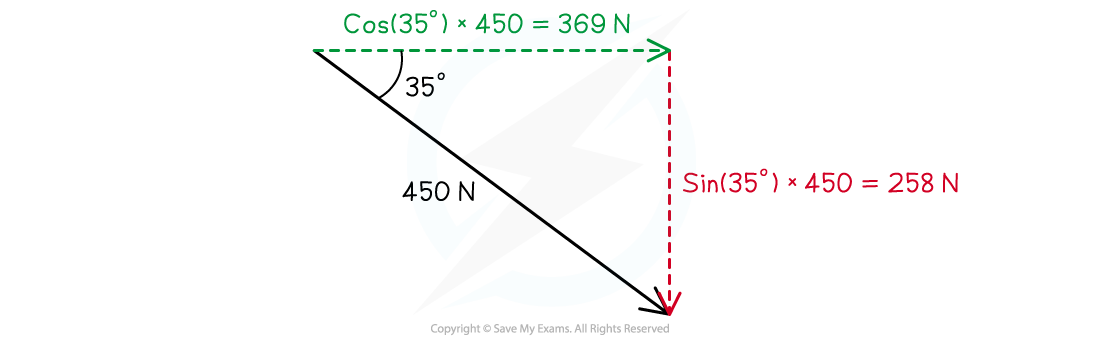
Step 3: Resolve the 450 N force into its horizontal and vertical components

• • The horizontal component can be resolved from:

cos(35°) × 450 = 369 N

• • This is directed to the right

• • The vertical component can be resolved from:

sin(35°) × 450 = 258 N

• • This is in a downwards direction

Step 4: Combine the horizontal components

• • The two forces provide 369 N to the right and 246 N to the left
  • Therefore, since these are opposing directions:

369 – 246 = 123 N to the right

Step 5: Combine the vertical components

• • The two forces provide 258 N downwards and 172 N upwards
  • Therefore, since these are opposing directions:

258 – 172 = 86 N downwards

Step 6: Make a right angle triangle using these two force vectors

• • There should be a longer size 123 N magnitude vector arrow to the right and then a 86 N magnitude vector arrow downwards
  • These can be connected from start to finish by a third vector which is the hypotenuse of this right-angled triangle

Step 7: Use the two vectors magnitudes to find the angle from the horizon

• • Since the vectors are to the right and downwards in this right-angle triangle, neither is the hypotenuse
  • Therefore the angle from the horizontal downwards can be found by using tan:

tan(θ°) = Opp. ÷ Adj. = 86 ÷ 123

θ° = tan–1(86 ÷ 123) = tan–1(0.699)

θ° ≈ 35°

Step 8: Use Pythagoras theorem or trigonometry to find the magnitude of the resultant force

• • Method 1: Using Pythagoras theorem
    • c² = b² + a² where b and a are the vector magnitudes for the horizontal and vertical components found so far and c is the hypotenuse magnitude

c² = 86² + 123² = 7396 + 15129 = 22525

c = √22 525 ≈ 150 N

• • Method 2: Using trigonometry
    • Using the horizontal component and the angle found in step 7, cos can be used

cos(35°) = Adj. ÷ Hyp. = 123 ÷ Hyp.

• • • Therefore:

123 ÷ cos(35°) = Hyp. ≈ 150 N

Step 9: State the final answer

• • The third force which would cause this object to remain stationary is 150 N right and 35° downwards from the horizontal