IB DP Maths: AI HL复习笔记5.2.3 Related Rates of Change
Related Rates of Change
What is meant by rates of change?
- A rate of change is a measure of how a quantity is changing with respect to another quantity
- Mathematically rates of change are derivatives
Context is important when interpreting positive and negative rates of change
- A positive rate of change would indicate an increase
- e.g. the change in volume of water as a bathtub fills
- A negative rate of change would indicate a decrease
- e.g. the change in volume of water in a leaking bucket
- A positive rate of change would indicate an increase
What is meant by related rates of change?
- Related rates of change are connected by a linking variable or parameter
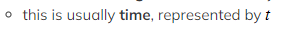seconds is the standard unit for time but this will depend on context
- e.g. Water running into a large bowl
- both the height and volume of water in the bowl change with time
- time is the linking parameter
How do I solve problems involving related rates of change?
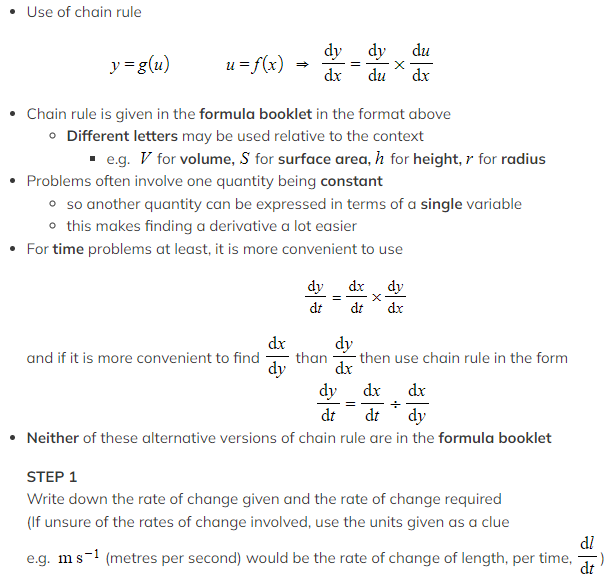STEP 2
Use chain rule to form an equation connecting these rates of change with a third rate
The third rate of change will come from a related quantity such as volume, surface area, perimeter
The third rate of change will come from a related quantity such as volume, surface area, perimeter
STEP 3
Write down the formula for the related quantity (volume, etc) accounting for any fixed quantities
Find the third rate of change of the related quantity (derivative) using differentiation
STEP 4
Substitute the derivative and known rate of cahnge into the equation and solve it
Exam Tip
Worked Example
转载自savemyexams
国际竞赛真题资料-点击免费领取！
欧几里得竞赛 寒假集训班开课了！
USACO寒假集训来袭！点击咨询！
