Home » 国际课程 » IB课程 » Details

IB DP Maths: AI HL复习笔记4.12.6 Type I & Type II Errors

Category: IB课程, 教材笔记, 福利干货 Date: 2022年7月21日下午6:32

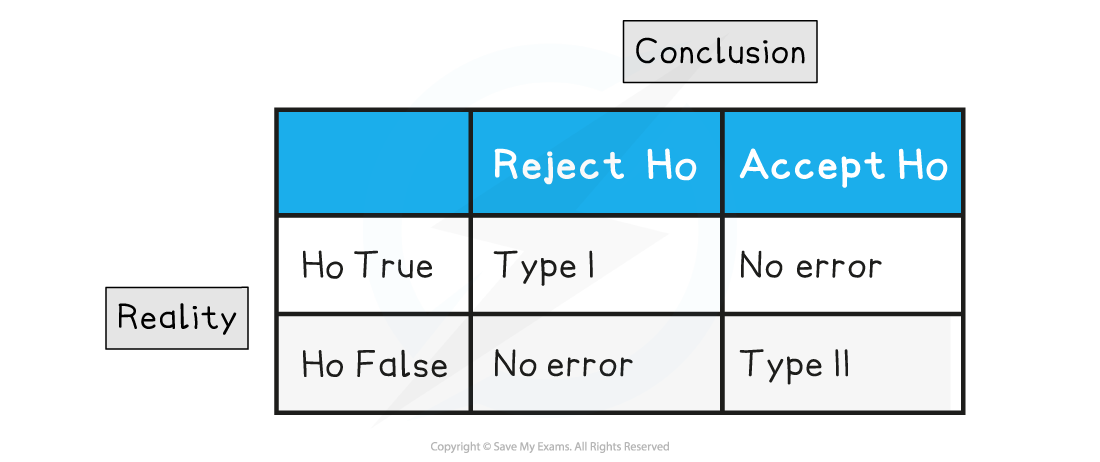
There are four possible outcomes of a hypothesis test:
- H₀ is false and H₀is rejected
- H₀ is true and H₀is not rejected
  - The test is accurate for these two outcomes
- H₀ is true and H₀is rejected
- H₀ is false and H₀is not rejected
  - The test has led to an error for these two outcomes
A Type I error occurs when a hypothesis test gives sufficient evidence to reject H₀despite it being true
- This is sometimes called a “false positive”
- In a court case this would be when the defendant is found guilty despite being innocent
A Type II error is when a hypothesis test gives insufficient evidence to reject H₀despite it being false
- This is sometimes called a “false negative”
- In a court case this would be when the defendant is found innocent despite being guilty

You can calculate the probability of errors occurring before a sample is taken
The probabilities are determined by the critical region
- Equally it is determined by the significance level α%
- Critical regions are determined such that:
  - They keep the probability of a Type I error less than or equal to the significance level
  - They maximise the probability of a Type I error
The probability of a Type I error occurring is equal to the probability of being in the critical region if H₀were true
- P(Type I error) = P(being in the critical region | H₀ is true)
- For a continuous distribution (normal, t, χ²)
  - P(Type I error) = α%
- For a discrete distribution (binomial, Poisson)
  - P(Type I error) ≤ α%
The probability of a Type II error occurring is equal to the probability of not being in the critical region given the actual value of the population parameter
- P(Type II error) = P(not being in critical region | actual population parameter)
Once a sample has been taken you can determine which error could have occurred
- If you rejected H₀then you could have made a Type I error
- If you accepted H₀then you could have made a Type II error

You can reduce the probability of a Type I error by reducing the significance level
- However this will increase the probability of a Type II error
You can reduce the probability of a Type II error by increasing the significance level
- However this will increase the probability of a Type I error
The only way to reduce both probabilities is by increasing the size of the sample

If an exam question asks you to find the probability of a Type I or II error then double check that the test has not been carried out yet
The examiner could test your understanding of errors by asking you to state which error could not have occurred once the test has been carried out