- 翰林提供学术活动、国际课程、科研项目一站式留学背景提升服务!
- 400 888 0080
IB DP Maths: AI HL复习笔记1.1.2 Approximation & Estimation
Approximating Values
How do I know what to round my answer to?
- Unless otherwise told, always round your answers to 3 significant figures (3 s.f.)
- The first non-zero digit is the first significant digit
- The first digit after the third significant digit determines whether to 'round up' (5) or 'leave it alone' (<5)
- where the ‘it’ we are rounding up or leaving alone is the third significant figure
-
- Your final answer will have three significant digits and the rest will be zero
- Any zero after the first significant digit is still significant
- For large numbers be careful not to change the place value of the significant digits, you will have to fill in any zeros after the third significant figure
- If your GDC is in scientific mode it may display unnecessary zeros after the decimal point, you do not need to copy these
- Your final answer will have three significant digits and the rest will be zero
- Look out for any questions that ask you to round your answer in a different way
- Questions often ask for 2 decimal places (2 d.p.)
- Your final answer will only have 2 digits after the decimal point
- For 2 d.p. it is the third digit after the decimal place that determines whether to 'round up' (5) or 'leave it alone' (<5)
- Questions often ask for 2 decimal places (2 d.p.)
- If you are working with a currency you must choose the appropriate degree of accuracy
- For most this will be a whole number
- E.g. yen, yuan, peso
- For others this will be to 2 decimal places
- E.g. dollars, euro, pounds
- It will be clear from the question which currency you are using and how you should round your answer
- The question will state the name of the currency and the symbol you should use as a unit
- E.g. YEN, ¥
- For most this will be a whole number
Are there cases when I always have to round up?
- Yes - there are cases when it makes sense to always round up (or down)
- These normally involve finding the minimum or maximum number of objects
 Exam Tip
Exam Tip
- In the exam you should always give non exact answers correct to 3 significant figures unless otherwise told
- This means you must round using a higher degree of accuracy within your working to ensure that your final answer is rounded correctly
- Where possible always use exact values within your working rather than rounding mid way through a question
Worked Example
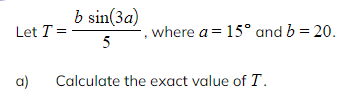
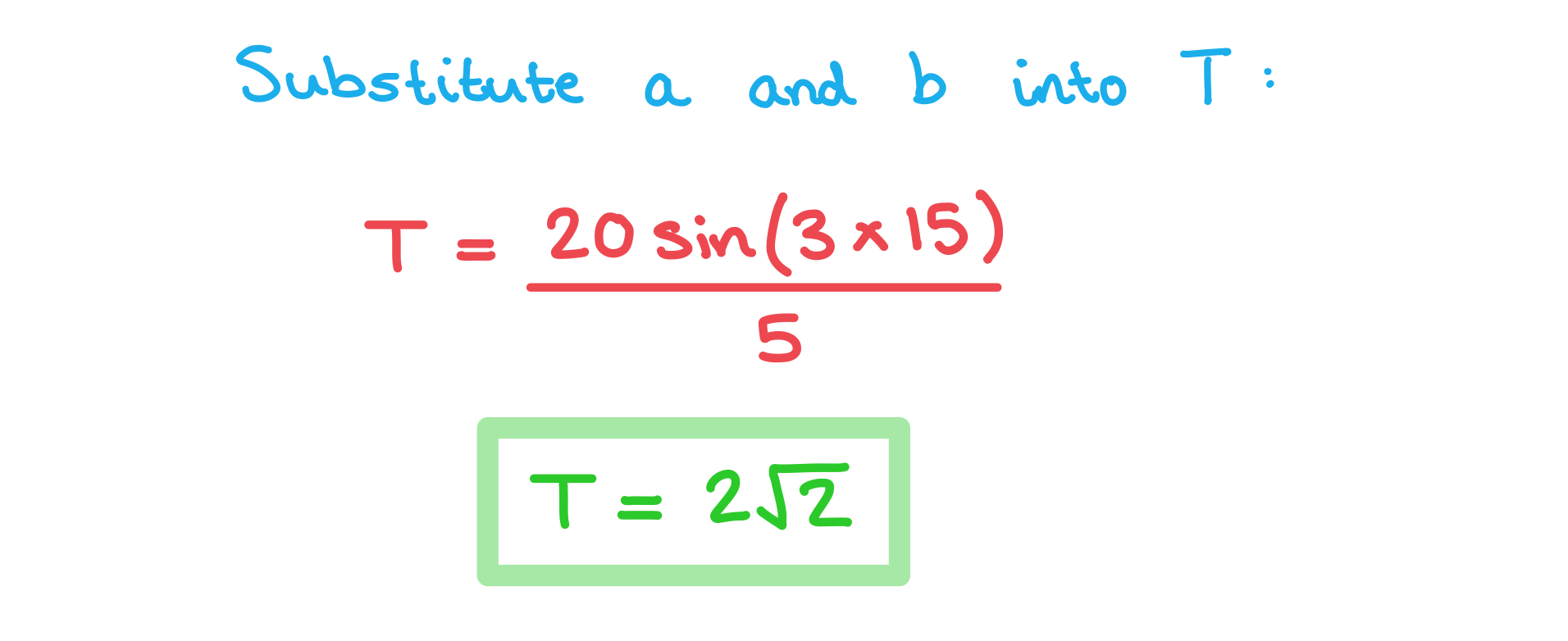
b)Give your answer from part a) correct to two decimal places.

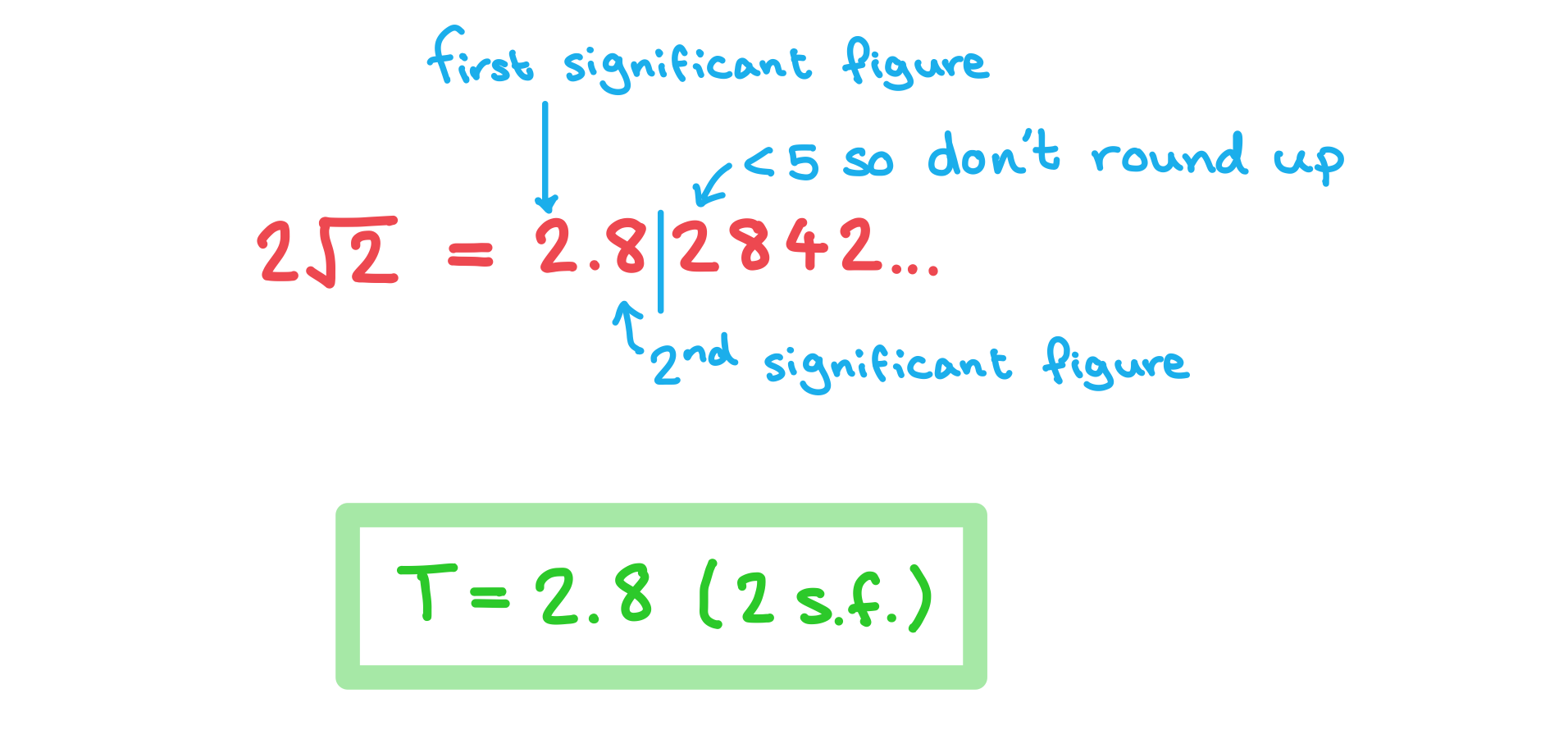
c)Give your answer from part a) correct to two significant figures.
Upper & Lower Bounds
What are bounds?
- Bounds are the smallest (lower bound, LB) and largest (upper bound, UB) numbers that a rounded number can lie between
- It simply means how low or high the number could have been before it was rounded
 How do we find bounds?
How do we find bounds?
- The basic rule is “half up, half down”
- To find the upper bound add on half the degree of accuracy
- To find the lower bound take off half the degree of accuracy
- Remember that the upper bound is the cut off point for the greatest value that the number could have been rounded from but will not actually round to the number itself
How do we calculate using bounds?
- Find bounds before carrying out the calculation and then use the rules:
- To add or multiply UB = UB + UB and LB = LB x LB etc
- To divide UB = UB / LB and LB = LB / UB
- To subtract UB = UB - LB and LB = LB – UB
- Use logic to decide which bound to use within the calculation
- For example if you are finding the maximum volume of a sphere with the radius given correct to 1 decimal place substitute the upper bound of the radius into your calculation for the volume
Exam Tip
- When in an exam environment it can be easy to make silly errors in questions like this, read the question carefully to determine which parts bounds need to be found for
- This will normally be any part in the question that has been rounded
Worked Example


Percentage Error
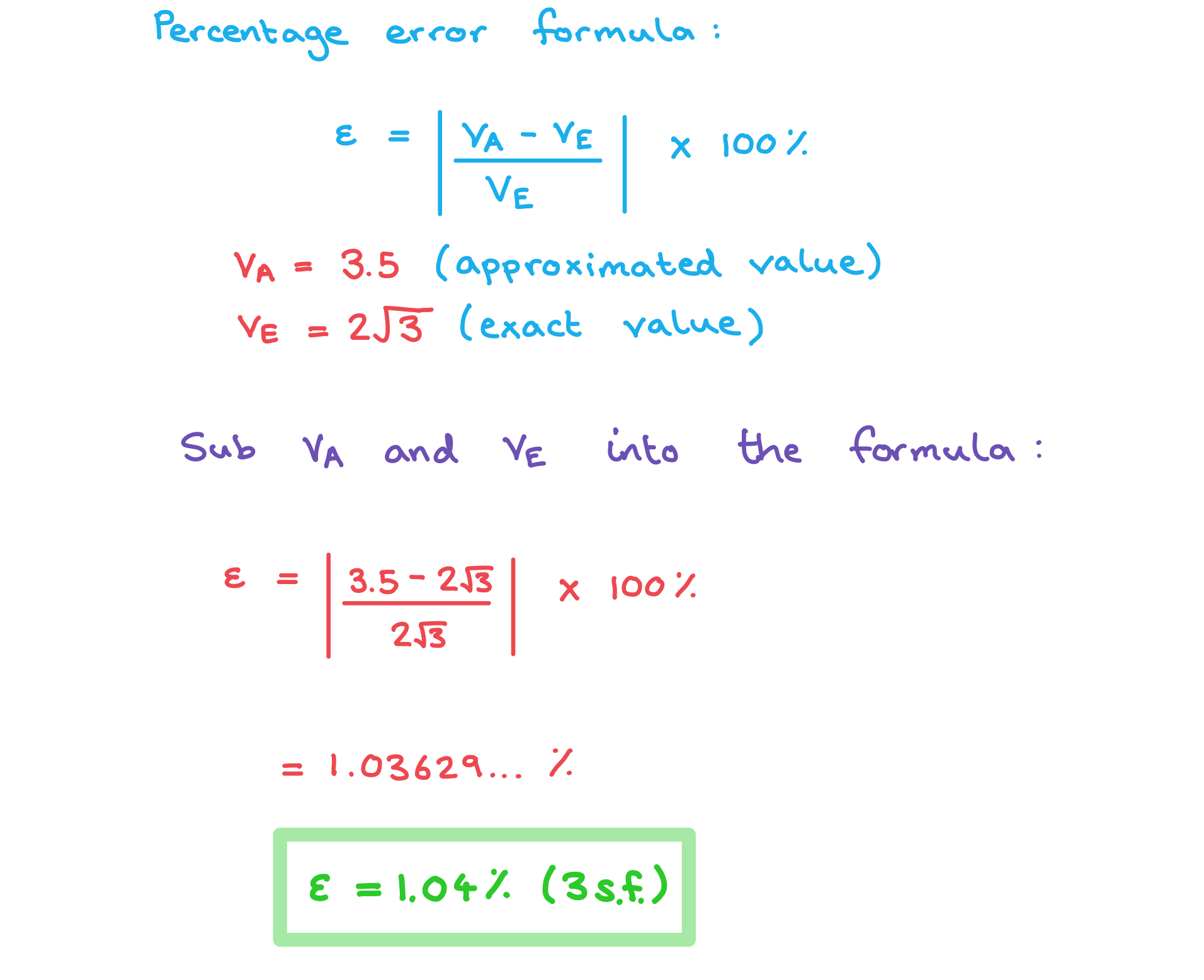
What is percentage error?
- Percentage error is how far away from the actual value an estimated or rounded answer is
- Percentage error can be calculated using the formula
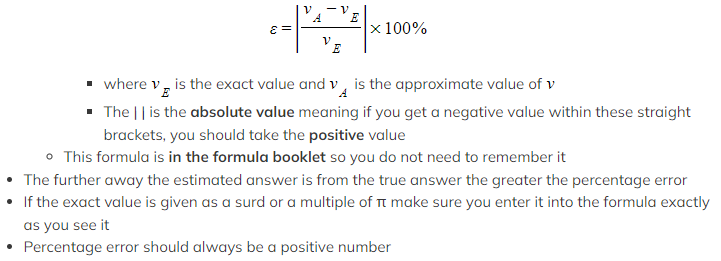
- Percentage error can be calculated using the formula
Exam Tip
- In the exam percentage error will usually be a part of a bigger question on another topic, make sure you know how to find the formula for it in the formula book so that you are prepared to answer these questions
Worked Example
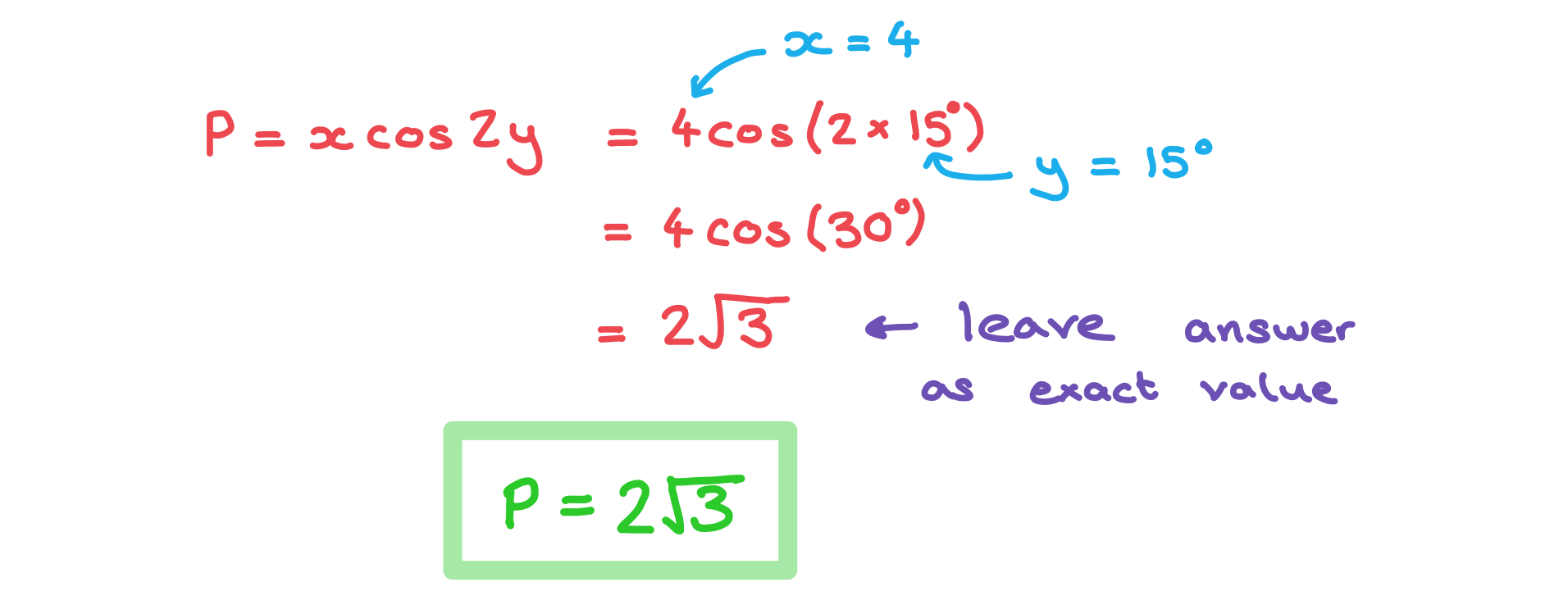
Accuracy & Estimation
What are exact values?
- Exact values forms that represent the full value of a number
 Why use estimation?
Why use estimation?
- We estimate to find approximate answers to difficult sums
- Or to check our answers are about the right size (order of magnitude)
- For example, if the question is to find a length the answer cannot be negative
- or if we are looking for the mean age of some people an answer of 150 must be incorrect
- Estimating an answer before carrying out a calculation will help you know what you are looking for and determine if your answer is likely to be correct or not
- In real life estimation skills are used every day in many activities
How do I choose the correct answer?
- Sometimes a mathematical argument will lead to more than one answer
- This is common with problems involving quadratics, you will usually have two solutions
- If you have more than one solution after you have solved a problem, always check to see if they are both valid
- Most of the time you can simply use logic to choose the correct answer
- If the problem involves length or area and one of the answers is negative, the true solution will be the positive answer
- Occasionally you will need to see if an answer can be valid
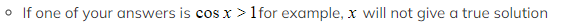 Exam Tip
Exam Tip
- Be aware that your GDC will not always give you an answer as an exact value, this means that you will need to find the exact value by hand
Worked Example
A rectangular floor has an area of 40 m2 to the nearest square metre. It is going to be tiled using square tiles with side length 39.8 cm.
a)Use estimation to find the number of tiles needed to cover the whole area.

b)Given that there are 15 more tiles places length-wise than width-wise, find the approximate length and width of the floor.

转载自savemyexams

早鸟钜惠!翰林2025暑期班课上线

最新发布
© 2025. All Rights Reserved. 沪ICP备2023009024号-1








