Edexcel IGCSE Physics 复习笔记 3.2.4 Snell's Law

• When light enters a denser medium (such as glass) it slows down and bends towards the normal
  • How much the light bends depends on the density of the material

   

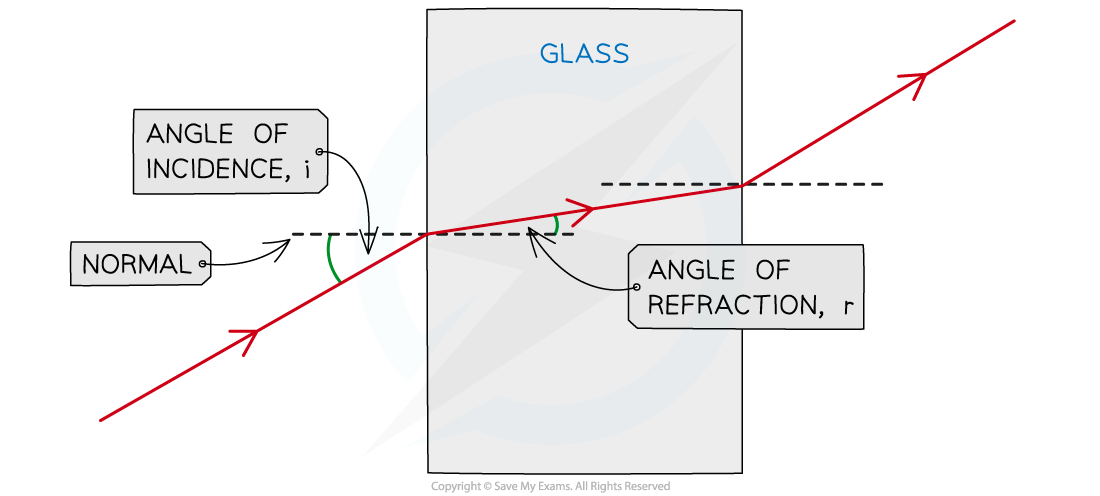
Angle of incidence i and angle of refraction r through a glass block

• If light travels from a less dense to a more dense medium (e.g. air to glass), r < i (bends towards the normal)
• If light travels from a more dense to a less dense medium (e.g. glass to air), r > i (bends away from the normal)

• The angles of incidence and refraction are related by an equation known as Snell's Law:

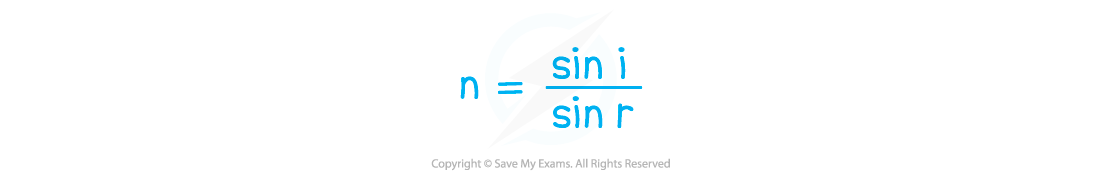
• Where:
  • n = the refractive index of the material
  • i = angle of incidence of the light (°)
  • r = angle of refraction of the light (°)

   

• 'Sin' is the trigonometric function 'sine' which is on a scientific calculator

• This equation can be rearranged with the help of the formula triangle:

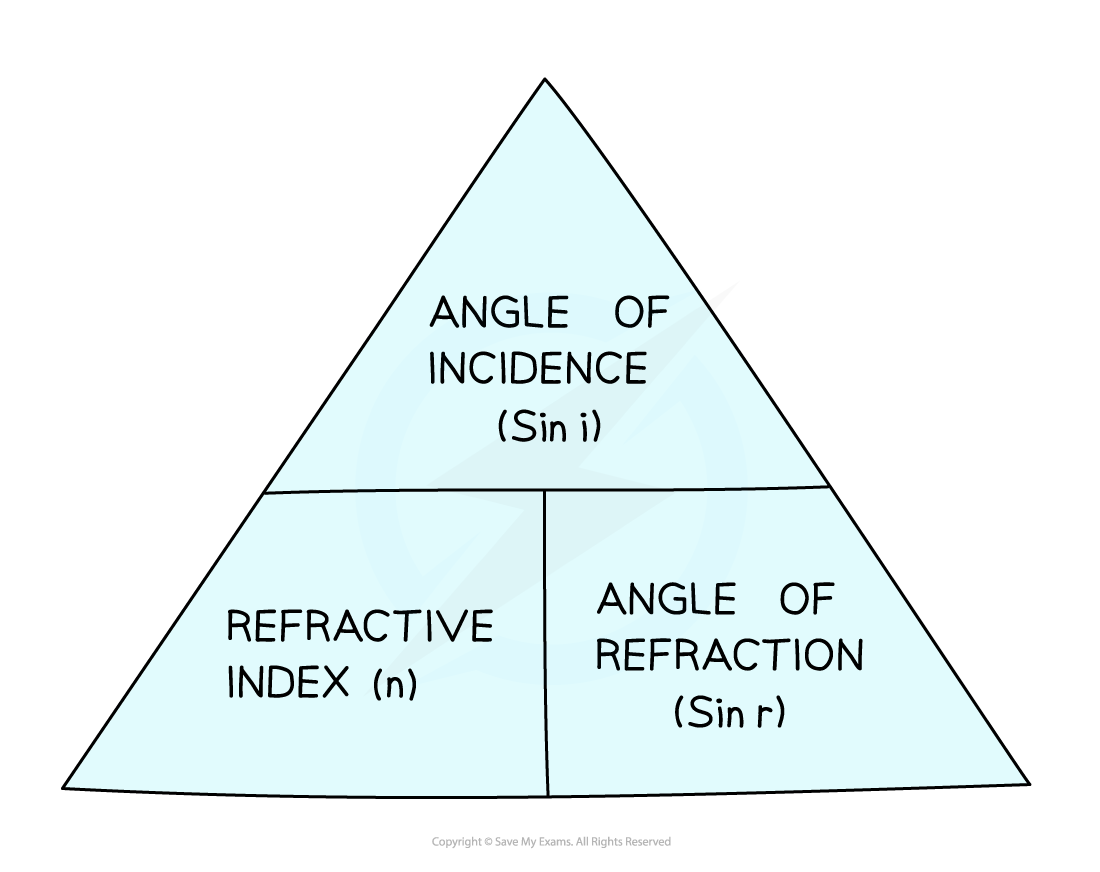
Snell's law formula triangle

• The refractive index is a number which is related to the speed of light in the material (which is always less than the speed of light in a vacuum):

• The refractive index is a number that is always larger than 1 and is different for different materials
  • Objects which are more optically dense have a higher refractive index, eg. n is about 2.4 for diamond
  • Objects which are less optically dense have a lower refractive index, eg. n is about 1.5 for glass

   

• Since refractive index is a ratio, it has no units

Step 1: List the known quantities

• • Refractive index of glass, n = 1.53
  • Angle of incidence, i = 15°

   

Step 2: Write the equation for Snell's Law

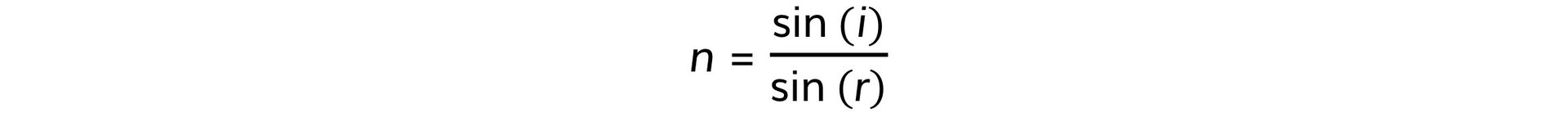
Step 3: Rearrange the equation and calculate sin (r)

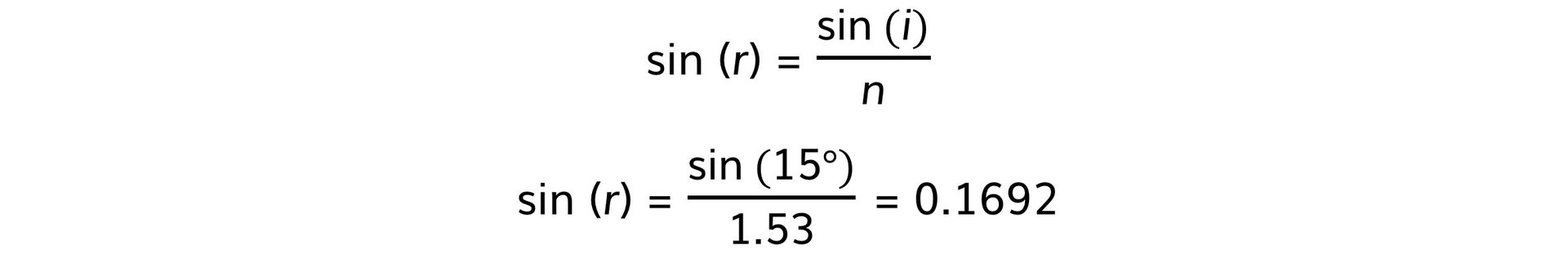
Step 4: Find the angle of refraction (r) by using the inverse sin function

r = sin^–1 (0.1692) = 9.7 = 10°