- 翰林提供学术活动、国际课程、科研项目一站式留学背景提升服务!
- 400 888 0080
AQA A Level Maths: Mechanics复习笔记3.3.1 Resolving Forces & Inclined Planes
Resolving Forces
If a single force is applied at an angle to the direction of motion it will need to be resolved into components of the force that act perpendicular to each other.
Why resolve a force acting at an angle?
- Resolving a force acting at an angle often helps to simplify a problem
- The components of a force parallel and perpendicular to the line of motion allows different types of problems to be solved
- The parallel component of a force acting directly on a particle will be the component that causes an effect on the particle
- The perpendicular component of a force acting directly on a particle will be the component that has no effect on the particle
- Finding the horizontal and vertical components of a force can help solve problems acting in the horizontal plane
- The two components of the force will have the same combined effect as the original force
How do you resolve a force acting at an angle?
- Use trigonometry to resolve a force acting at an angle
- Draw a vector triangle of forces by decomposing the force into its horizontal and vertical components
- The original force will be the hypotenuse of the triangle and the two components will make up the opposite and adjacent sides
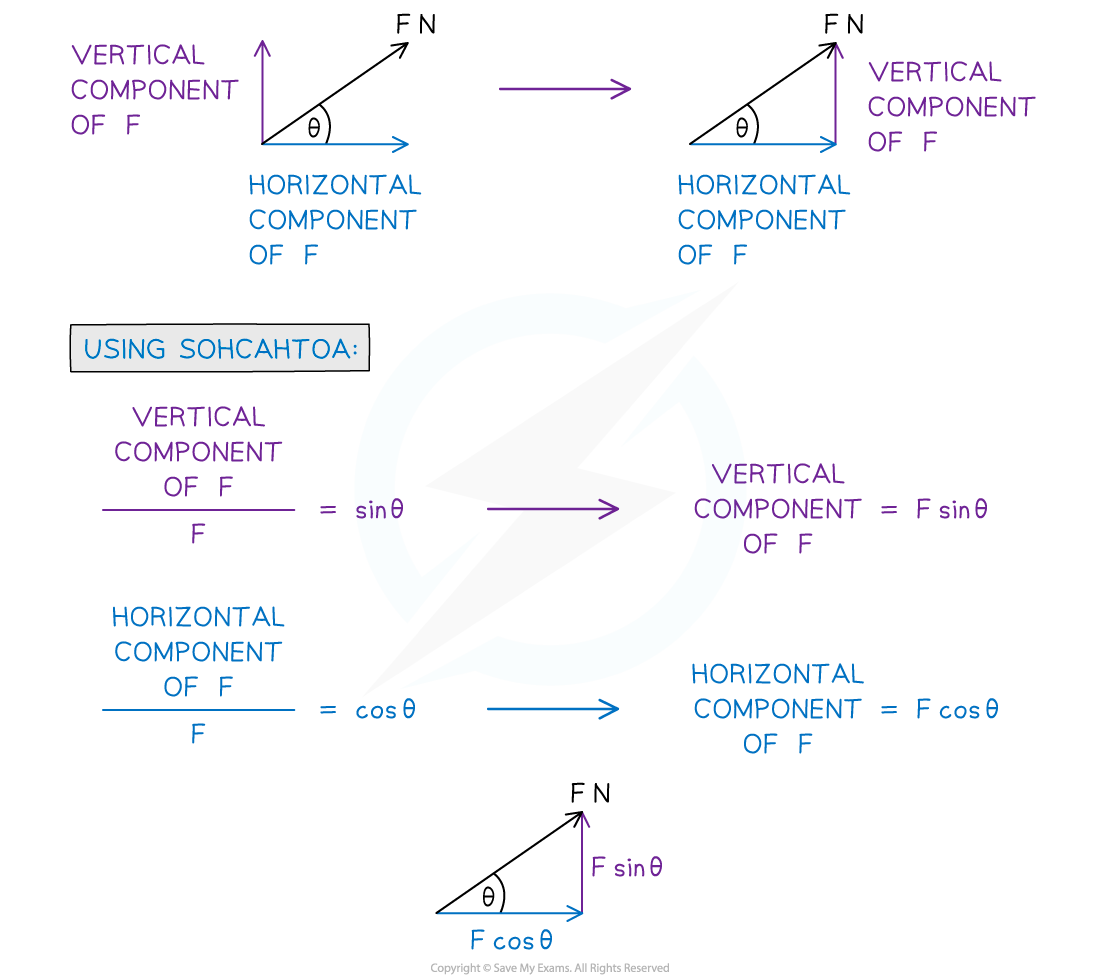
How do we find a resultant force when two or more forces are acting at angles to each other?
- Force is a vector quantity, so finding the resultant of two or more forces is the same as finding the resultant of two or more vectors
- It is possible to use geometry to find the resultant force without decomposing the forces into their horizontal and vertical components
- Use the triangle law for vector addition to calculate the magnitude and direction of a resultant force
- You will need to use trigonometry to find the missing side or angle in the triangle.
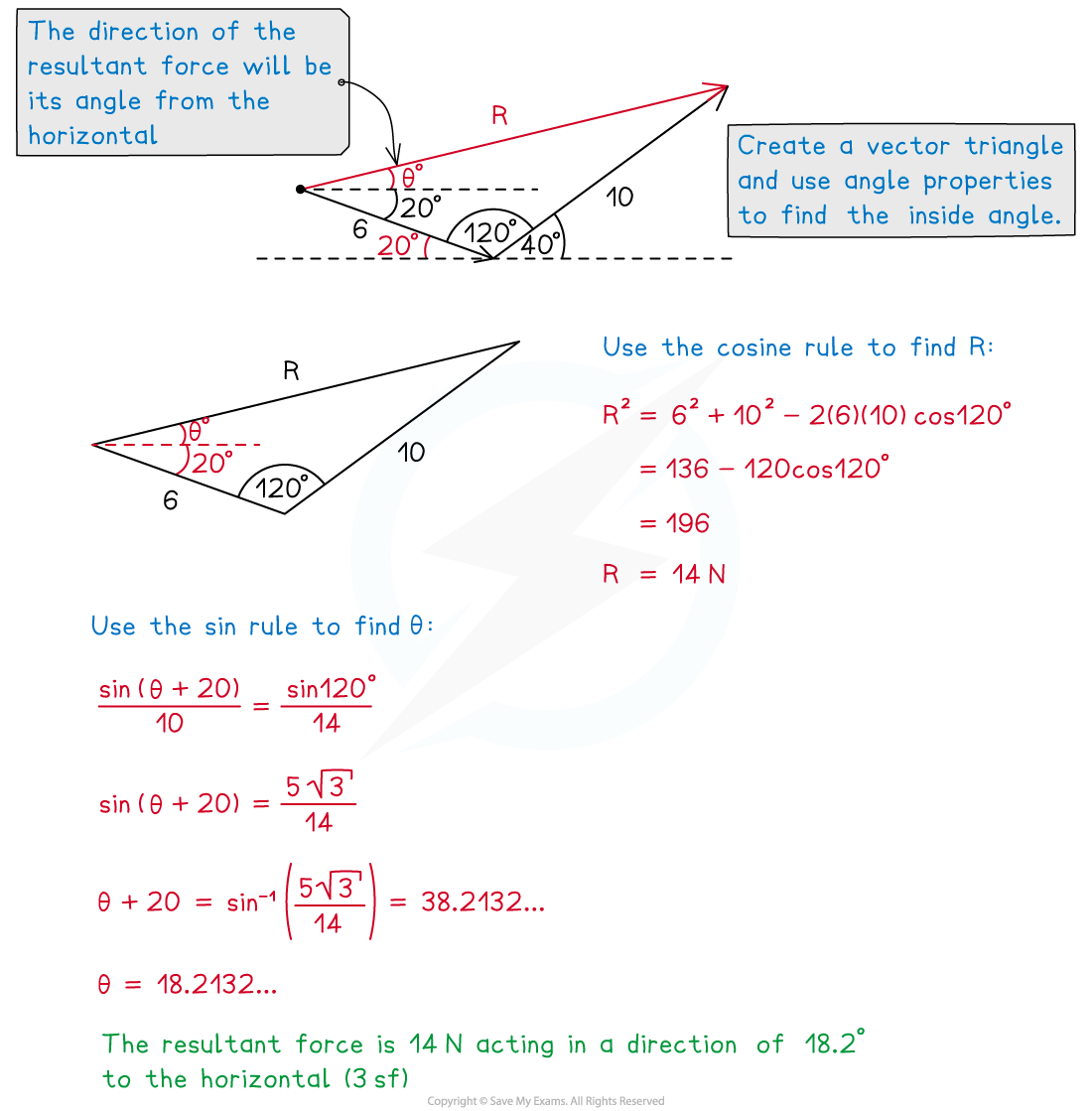
Worked Example

-
- Find the vertical and horizontal components of the 10 N force.
- Explain why the resultant force acting to move the box is
转载自savemyexams

最新发布
© 2025. All Rights Reserved. 沪ICP备2023009024号-1









