IB DP Maths: AA HL复习笔记5.9.2 Further Techniques of Integration
Integration by Substitution
What is integration by substitution?
- Integration by substitution is used when an integrand where reverse chain rule is either not obvious or is not spotted
- in the latter case it is like a “back-up” method for reverse chain rule
How do I use integration by substitution?
Exam Tip
- If a substitution is not given in a question, it is usually because it is obvious
- If you can't see anything obvious, or you find that your choice of substitution doesn't reduce the integrand to something easy to integrate, consider that it may not be a substitution question
Worked Example
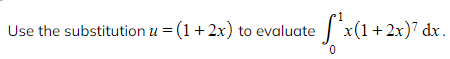
Integration by Parts
What is integration by parts?
What is the formula for integration by parts?
How do I use integration by parts?
In trickier problems other rules of differentiation and integration may be needed
- chain, product or quotient rule
- reverse chain rule, substitution
Can integration by parts be used when there is only a single function?
Exam Tip
Worked Example
Repeated Integration by Parts
When will I have to repeat integration by parts?
How do I apply integration by parts more than once?
What if neither function never becomes simpler when differentiating?
Worked Example
转载自savemyexams
国际竞赛真题资源免费领取
美高学分项目重磅来袭！立即了解
