- 翰林提供学术活动、国际课程、科研项目一站式留学背景提升服务!
- 400 888 0080
AQA A Level Maths: Mechanics复习笔记2.2.1 Using Calculus in 1D
Using Calculus in 1D
How is calculus used in kinematics?
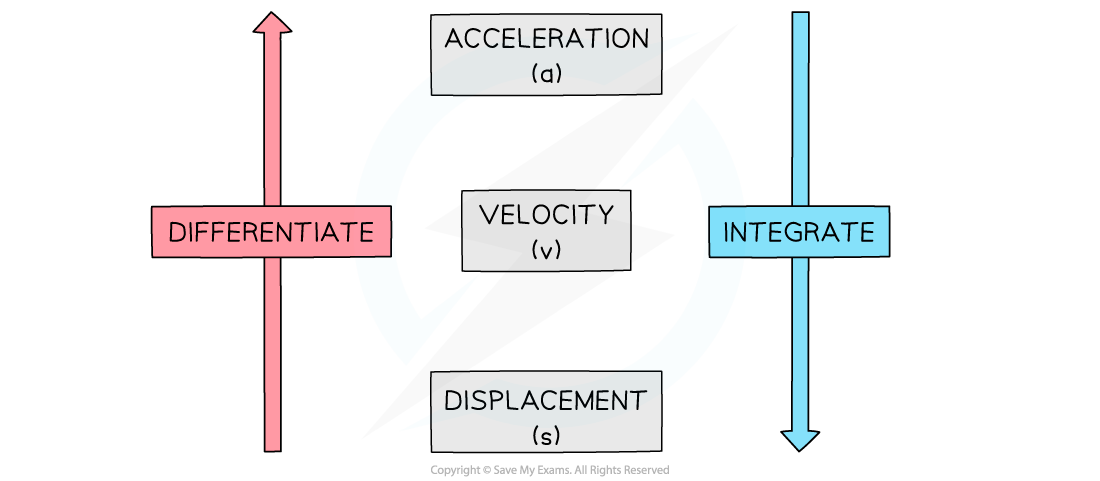
- s, v and a are all functions of time
- Velocity, v, is the rate of change of displacement, s, with respect to time
- Acceleration, a, is the rate of change of velocity, v, with respect to time
- Differentiate to go from s to v and from v to a
- Integrate to go from a to v and from v to s
- There will be a constant of integration, c, each time you integrate
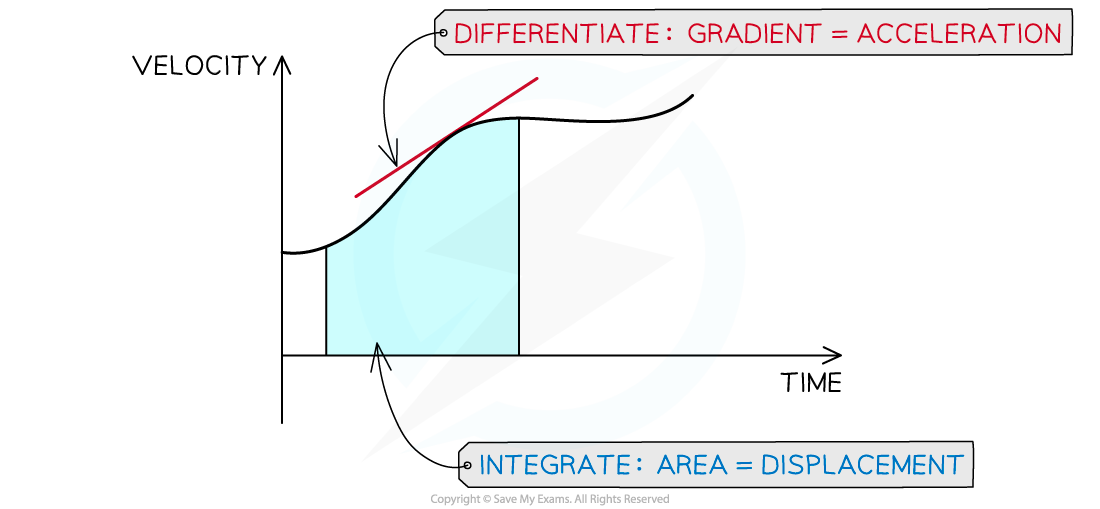
- The use of calculus can be seen using velocity-time graphs
- Acceleration is the gradient which is found using differentiation
- Displacement is the area under the graph which is found using integration
How do I find c (the constant of integration) in kinematics?
- The value of c can be found by substituting known values, as in integration for pure maths
- Information to find c may be given in words – look out for common phrases …
- “… starting from rest …”: this means v = 0 when t = 0
- “… initially …” – this means t = 0
Definite integration in kinematics
- Integrating acceleration between two values of time gives the change in velocity of the object between those times
- Integrating velocity between two values of time gives the displacement of the object between those times
- This could be different to the distance travelled between those times
- To find the total distance travelled you would need to calculate the areas above and below the x-axis separately
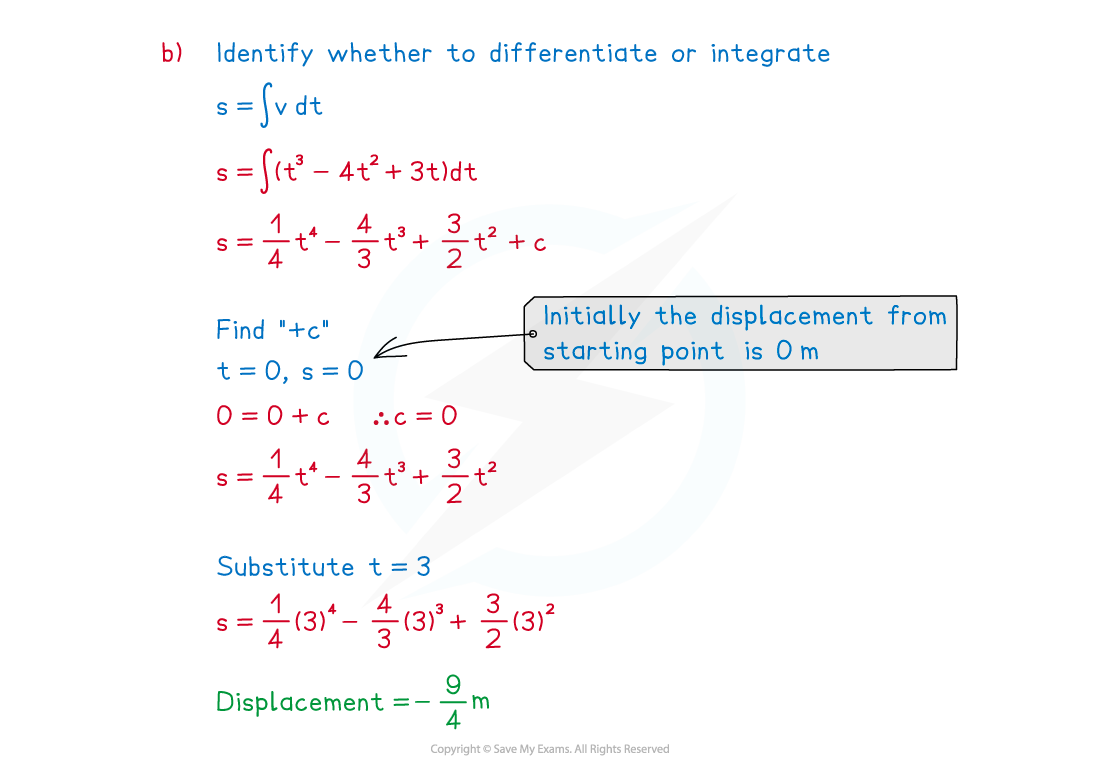
Worked Example
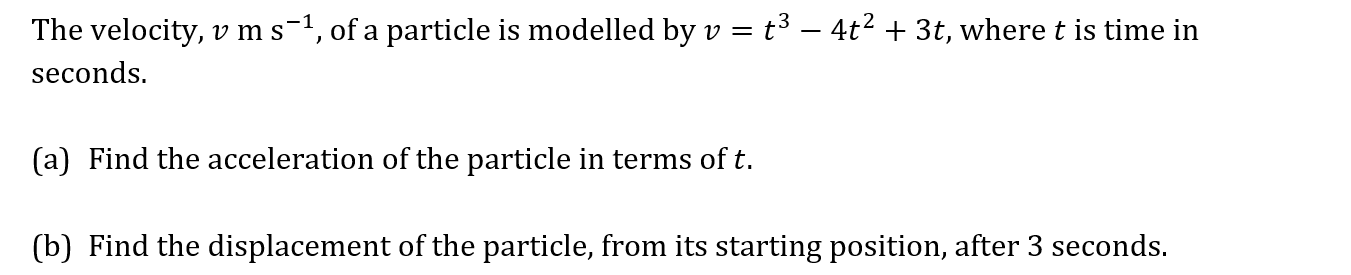
![]()

(b) Find the displacement of the particle, from its starting position, after 3 seconds.
Exam Tip
- Look for key words and phrases that indicate scalar, rather than vector quantities:
- “distance …”, “speed” and “magnitude of …” are scalar
- Displacement, velocity, acceleration are vectors
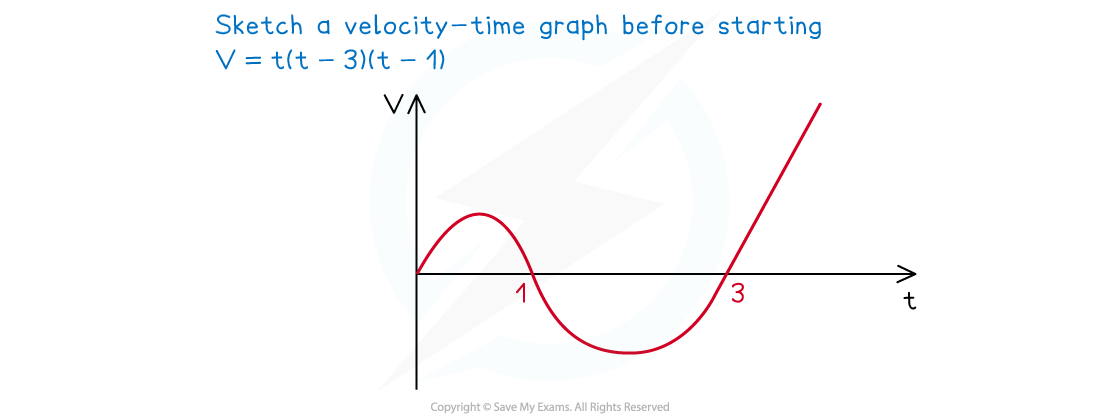
- A sketch of the velocity-time graph is always helpful as you can use it to see important features such as times when the object is stationary. This is especially useful you are asked to find the total distance travelled.
转载自savemyexams

早鸟钜惠!翰林2025暑期班课上线

最新发布
© 2025. All Rights Reserved. 沪ICP备2023009024号-1








