- 翰林提供学术活动、国际课程、科研项目一站式留学背景提升服务!
- 400 888 0080
AQA A Level Physics复习笔记8.2.2 Exponential Decay
Exponential Decay
- In radioactive decay, the number of undecayed nuclei falls very rapidly, without ever reaching zero
- Such a model is known as exponential decay
- The graph of number of undecayed nuclei against time has a very distinctive shape:
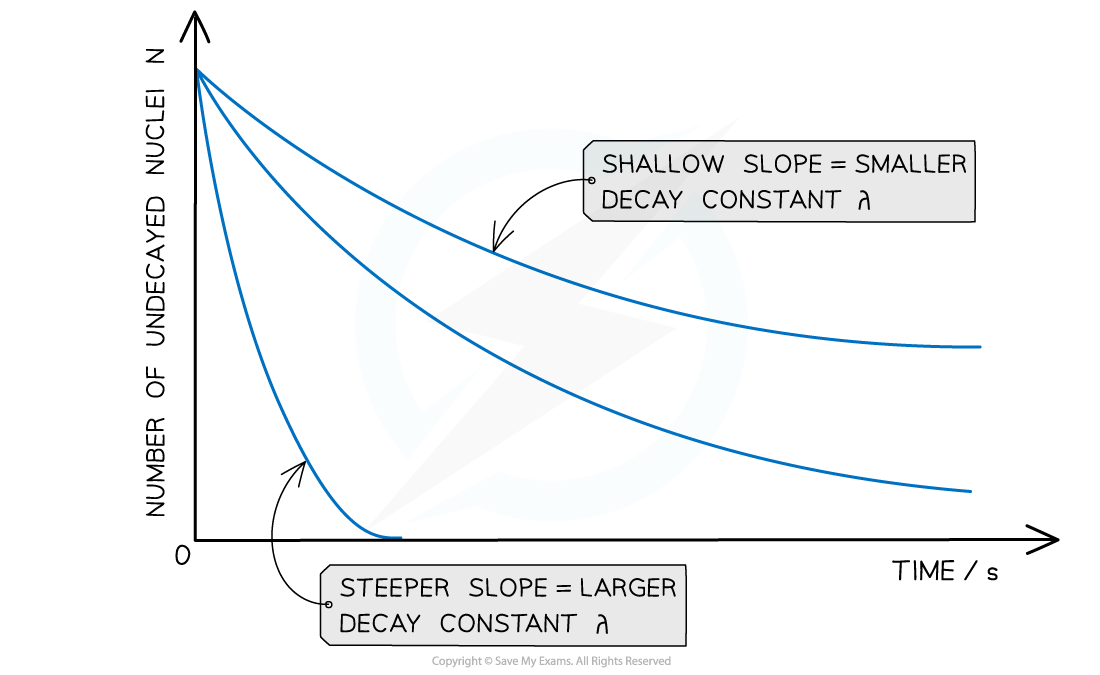
Radioactive decay follows an exponential pattern. The graph shows three different isotopes each with a different rate of decay
- The key features of this graph are:
- The steeper the slope, the larger the decay constant λ (and vice versa)
- The decay curves always start on the y-axis at the initial number of undecayed nuclei (N0)
Equations for Radioactive Decay
- The number of undecayed nuclei N can be represented in exponential form by the equation:
N = N0 e–λt
- Where:
- N0 = the initial number of undecayed nuclei (when t = 0)
- N = number of undecayed nuclei at a certain time t
- λ = decay constant (s-1)
- t = time interval (s)
- The number of nuclei can be substituted for other quantities.
- For example, the activity A is directly proportional to N, so it can also be represented in exponential form by the equation:
A = A0 e–λt
- Where:
- A = activity at a certain time t (Bq)
- A0 = initial activity (Bq)
- The received count rate C is related to the activity of the sample, hence it can also be represented in exponential form by the equation:
C = C0 e–λt
- Where:
- C = count rate at a certain time t (counts per minute or cpm)
- C0 = initial count rate (counts per minute or cpm)
The exponential function e
- The symbol e represents the exponential constant
- It is approximately equal to e = 2.718
- On a calculator it is shown by the button ex
- The inverse function of ex is ln(y), known as the natural logarithmic function
- This is because, if ex = y, then x = ln(y)
Worked Example
Strontium-90 decays with the emission of a β-particle to form Yttrium-90.The decay constant of Strontium-90 is 0.025 year -1.Determine the activity A of the sample after 5.0 years, expressing the answer as a fraction of the initial activity A0.
Step 1: Write out the known quantities
-
- Decay constant, λ = 0.025 year -1
- Time interval, t = 5.0 years
- Both quantities have the same unit, so there is no need for conversion
Step 2: Write the equation for activity in exponential form
A = A0 e–λt
Step 3: Rearrange the equation for the ratio between A and A0
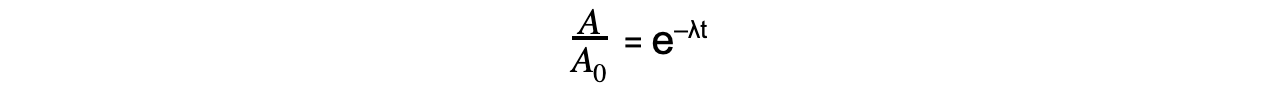
Step 4: Calculate the ratio A/A0
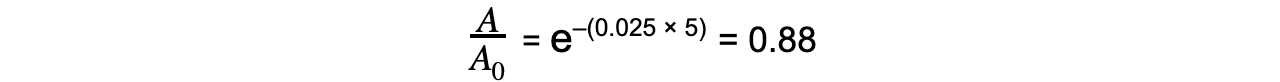
Therefore, the activity of Strontium-90 decreases by a factor of 0.88, or 12%, after 5 years
Using Molar Mass & The Avogadro Constant
Molar Mass
- The molar mass, or molecular mass, of a substance is the mass of a substance, in grams, in one mole
- Its unit is g mol-1
- The number of moles from this can be calculated using the equation:
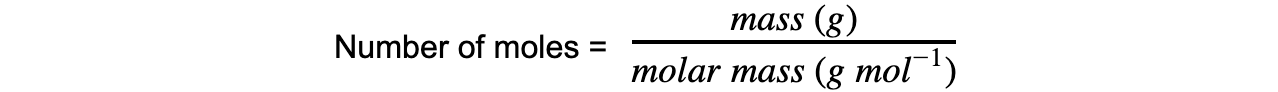
Avogadro’s Constant
- Avogadro’s constant (NA) is defined as:
The number of atoms in one mole of a substance; equal to 6.02 × 1023 mol-1
- For example, 1 mole of sodium (Na) contains 6.02 × 1023 atoms of sodium
- The number of atoms, N, can be determined using the equation:
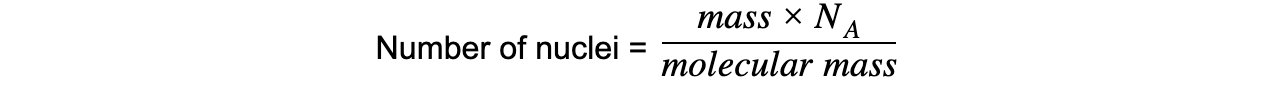
Worked Example
Americium-241 is an artificially produced radioactive element that emits α-particles.In a smoke detector, a sample of americium-241 of mass 5.1 µg is found to have an activity of 5.9 × 105 Bq. The supplier’s website says the americium-241 in their smoke detectors initially has an activity level of 6.1 × 105 Bq.
a) Determine the number of nuclei in the sample of americium-241
b) Determine the decay constant of americium-241
c) Determine the age of the smoke detector in years
Part (a)
Step 1: Write down the known quantities
-
- Mass = 5.1 μg = 5.1 × 10-6 g
- Molecular mass of americium = 241
Step 2: Write down the equation relating number of nuclei, mass and molecular mass
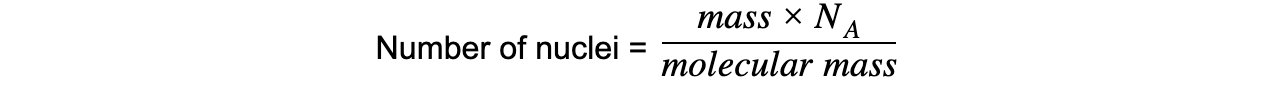
-
- where NA is the Avogadro constant
Step 3: Calculate the number of nuclei
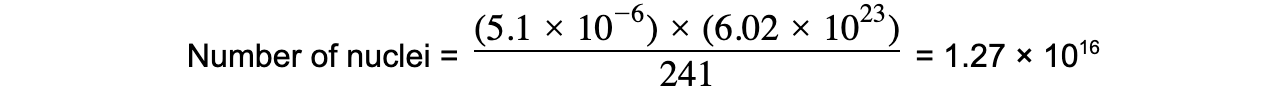
Part (b)
Step 1: Write down the known quantities
-
- Activity, A = 5.9 × 105 Bq
- Number of nuclei, N = 1.27 × 1016
Step 2: Write the equation for activity
Activity, A = λN
Step 3: Rearrange for decay constant λ and calculate the answer
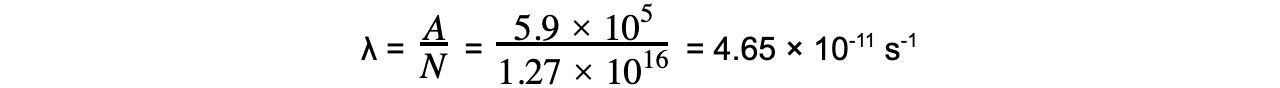
Part (c)
Step 1: Write down the known quantities
-
- Activity, A = 5.9 × 105 Bq
- Initial activity, A0 = 6.1 × 105 Bq
- Decay constant, λ = 4.65 × 10–11 s–1
Step 2: Write the equation for activity in exponential form
A = A0 e–λt
Step 3: Rearrange for time t
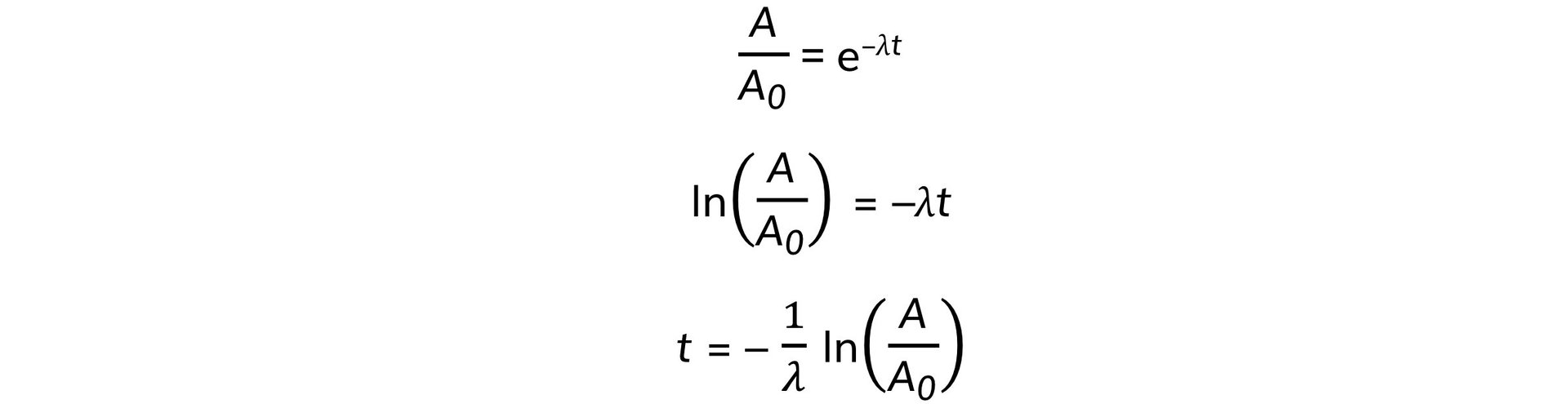
Step 4: Calculate the age of the smoke detector and convert to years

-
- Therefore, the smoke detector is 22.7 years old
转载自savemyexams


最新发布
© 2025. All Rights Reserved. 沪ICP备2023009024号-1








