- 翰林提供学术活动、国际课程、科研项目一站式留学背景提升服务!
- 400 888 0080
AQA A Level Physics复习笔记4.6.2 Area Under a Force-Displacement Graph
Area Under a Force-Displacement Graph
- The work done can also be found from a force-displacement graph
- If the force is not constant and is plotted against the displacement of the object:
- The work done is equal to the area under the force-displacement graph
- This is because:
Work done = Force × Displacement
- The work done is therefore equivalent whether there is:
- A small force over a long displacement
- A large force over a small displacement
- The graph may need to be split up into sections. The total area is the sum of the areas of each section
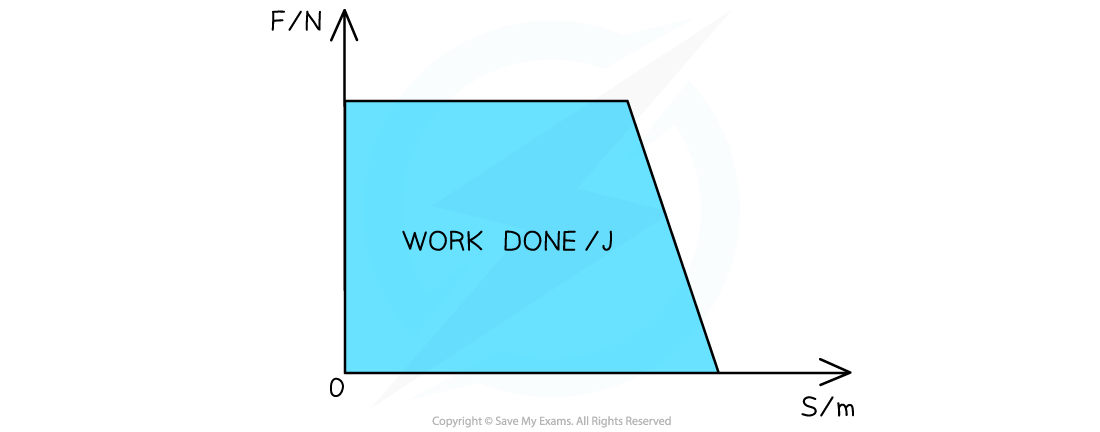
The area underneath the force-displacement graph is the work done
Worked Example
The graph shows how a force varies over a displacement of 80 m.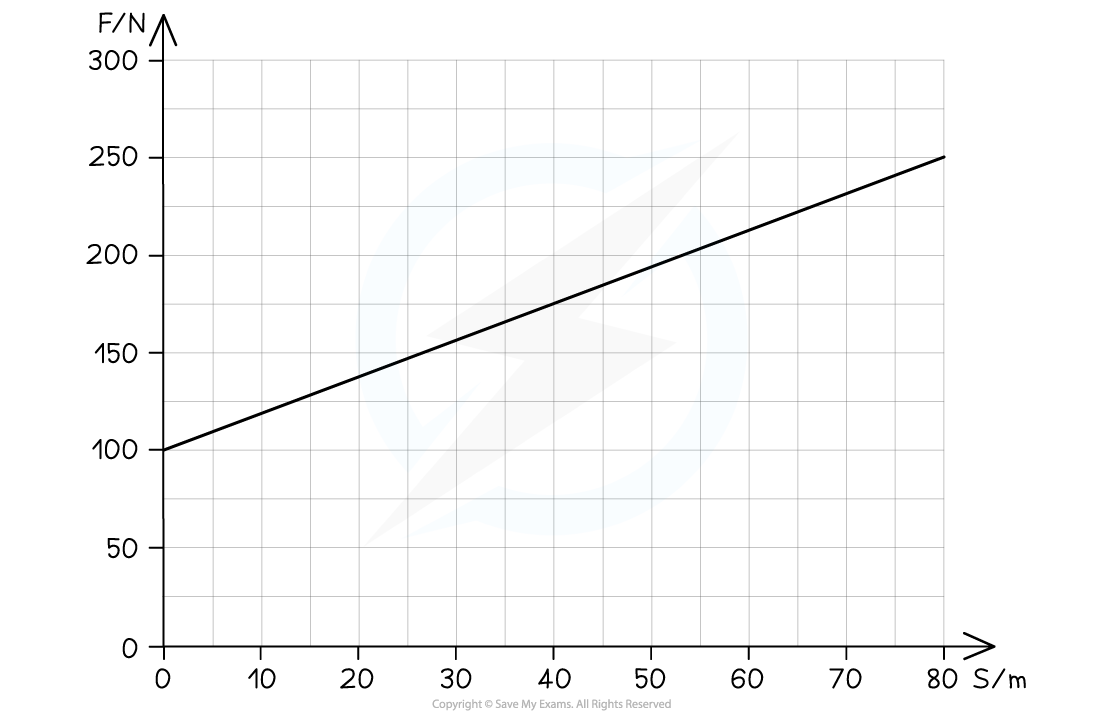 Calculate the work done.
Calculate the work done.
Step 1: Split the graph into sections
The work done is the area under the graph
The total area can be found by splitting the graph into sections A and B

Step 2: Calculate the area of section A
Section A is a right-angled triangle where the area is 0.5 × base × height
0.5 × 80 × (250 – 100) = 6000 J
Step 3: Calculate the area of section B
Section B is a rectangle where the area is base × height
80 × 100 = 8000 J
Step 4: Calculate the total work done
The total work done is the sum of both areas
Work done = 6000 + 8000 = 14 000 J
Exam Tip
Always check the units on the axes when calculating values from a graph. Sometimes the force will be given in kN or the displacement in km. These must be converted into SI units to calculate the work done in J.
Variable Forces
- The force on an object may not always be constant, this is known as a variable force
- This is more representative of a force in real life
- If a force is constant, then the following equations can be used:
W = Fs
P = Fv
- If a force is varying, the above equations cannot be used, instead, work done must be found from the area under the force-displacement graph
- If a varying force increases, then an object’s acceleration increases and vice versa
Worked Example
A person is pulling a suitcase through an airport with a rough surface.They apply a force of 150 N over a distance of 12 m. Afterwards, the person gets progressively tired and the applied force is linearly reduced to 60 N. The total distance through which the suitcase has moved is 25 m.Calculate the work done by the force applied by the person over 25 m.
Step 1: Sketch a force-displacement graph and split it into sections
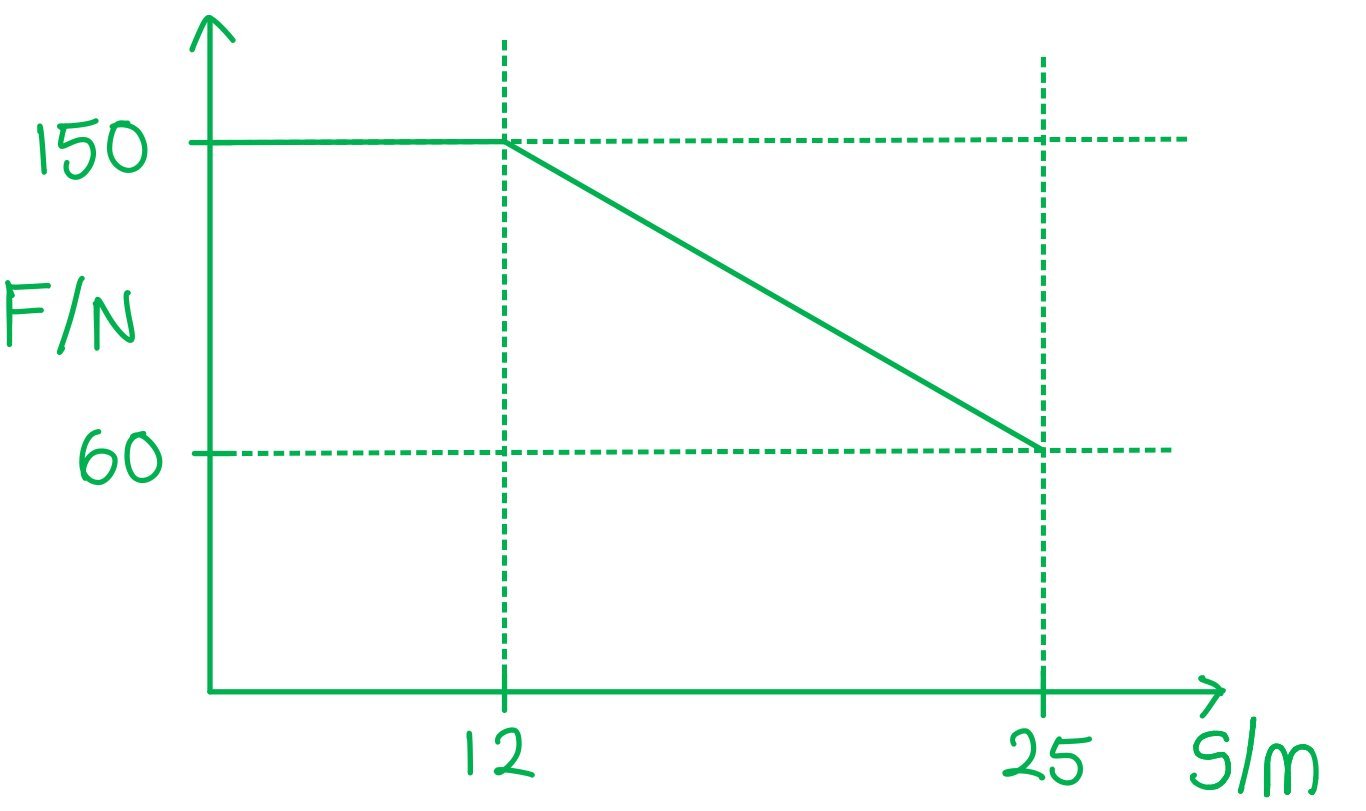
Step 2: Split the graph into sections
-
- The work done is the area under the graph
- The total area can be found by splitting the graph into sections A and B
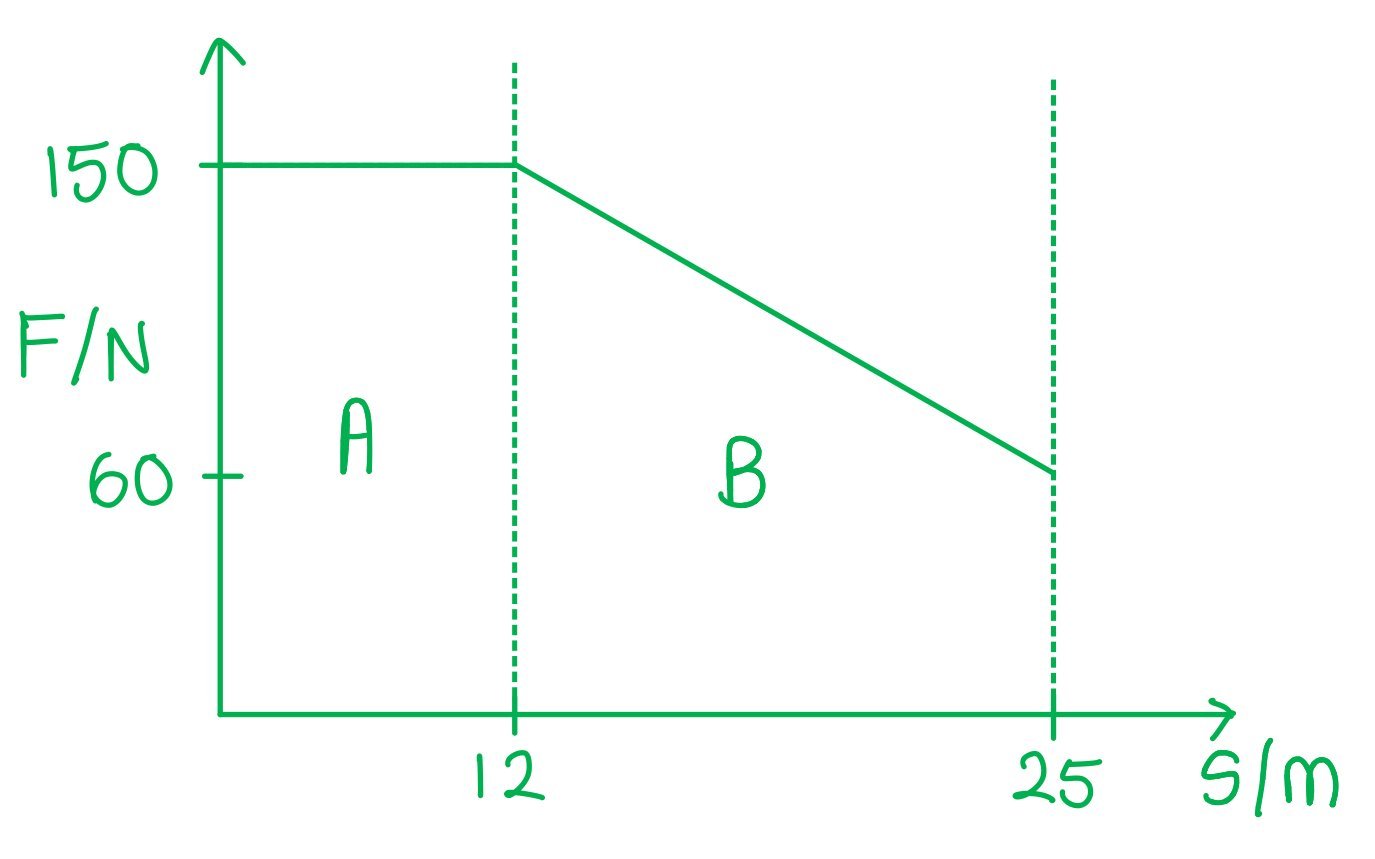
Step 3: Calculate the area of section A
-
- Section A is a rectangle where the area is base × height
12 × 150 = 1800 J
Step 4: Calculate the area of section B
-
- Section B is a trapezium where the area is
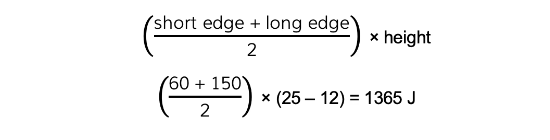 Step 5: Calculate the total work done
Step 5: Calculate the total work done
-
- The total work done is the sum of both areas
Work done = 1800 + 1365 = 3165 J
Exam Tip
When sketching graphs, they don’t have to be to scale. However, it is important to label the key points on the x and y-axis from the question to calculate the areas underneath the graph.
转载自savemyexams

最新发布
© 2025. All Rights Reserved. 沪ICP备2023009024号-1









