紫色彗星数学竞赛真题（2014年初中组）

PURPLE COMET! MATH MEET April 2014
MIDDLE SCHOOL - PROBLEMS
Copyright © Titu Andreescu and Jonathan Kane

Problem 1

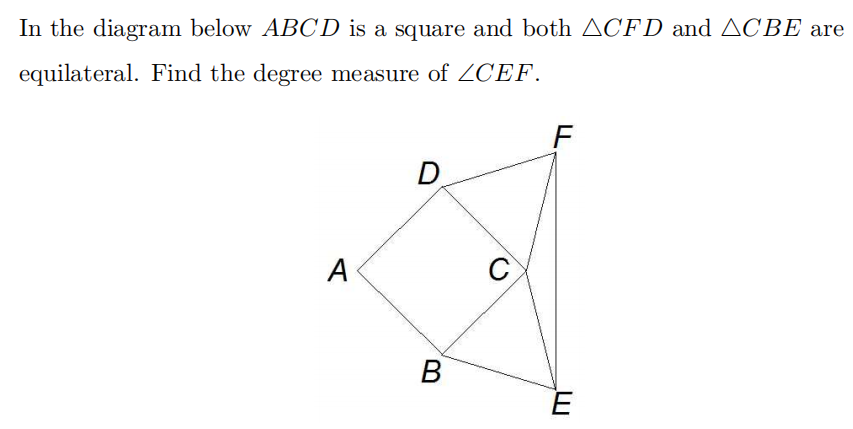
Problem 2

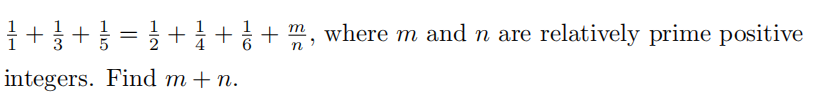
Problem 3
The cross below is made up of five congruent squares. The perimeter of the cross is 72. Find its area.

Problem 4
One-third of the students who attend Grant School are taking Algebra. One-quarter of the students taking Algebra are also on the track team. There are 15 students on the track team who take Algebra. Find the number of students who attend Grant School.
Problem 5
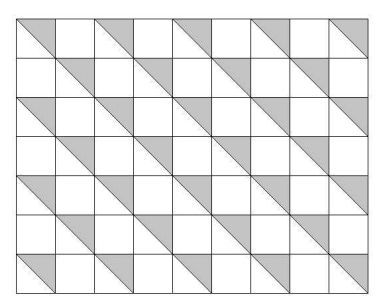
The figure below shows a 9 × 7 arrangement of 2 × 2 squares. Alternate squares of the grid are split into two triangles with one of the triangles shaded. Find the area of the shaded region.

Problem 6
The twenty-first century began on January 1, 2001 and runs through December 31, 2100. Note that March 1, 2014 fell on Saturday, so there were five Mondays in March 2014. In how many years of the twenty-first century does March have five Mondays?
Problem 7
Inside the 7 × 8 rectangle below, one point is chosen a distance √ 2 from the left side and a distance √ 7 from the bottom side. The line segments from that point to the four vertices of the rectangle are drawn. Find the area of the shaded region.

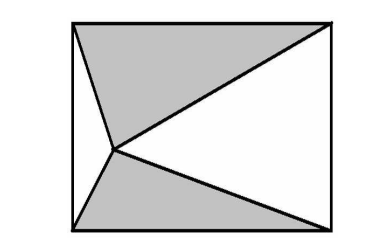
Problem 8
Johan is swimming laps in the pool. At 12:17 he realized that he had just finished one-third of his workout. By 12:22 he had completed eight more laps, and he realized that he had just finished five-elevenths of his workout. After 12:22 how many more laps must Johan swim to complete his workout?
Problem 9
The diagram below shows a shaded region bounded by a semicircular arc of a large circle and two smaller semicircular arcs. The smallest semicircle has radius 8, and the shaded region has area 180π. Find the diameter of the large circle.

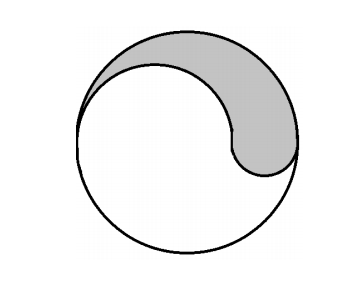
Problem 10
Given that x and y satisfy the two equations

Problem 11
Shenelle has some square tiles. Some of the tiles have side length 5 cm while the others have side length 3 cm. The total area that can be covered by the tiles is exactly 2014 cm². Find the least number of tiles that Shenelle can have.
Problem 12
The first number in the following sequence is 1. It is followed by two 1’s and two 2’s. This is followed by three 1’s, three 2’s, and three 3’s. The sequence continues in this fashion.
1, 1, 1, 2, 2, 1, 1, 1, 2, 2, 2, 3, 3, 3, 1, 1, 1, 1, 2, 2, 2, 2, 3, 3, 3, 3, 4, 4, 4, 4, . . . .
Find the 2014th number in this sequence.
Problem 13
A jar was filled with jelly beans so that 54% of the beans were red, 30% of the beans were green, and 16% of the beans were blue. Alan then removed the same number of red jelly beans and green jelly beans from the jar so that now 20% of the jelly beans in the jar are blue. What percent of the jelly beans in the jar are now red?
Problem 14
Steve needed to address a letter to 2743 Becker Road. He remembered the digits of the address, but he forgot the correct order of the digits, so he wrote them down in random order. The probability that Steve got exactly two of the four digits in their correct positions is , where m and n are relatively prime positive integers. Find m + n.
Problem 15
A large rectangle is tiled by some 1 × 1 tiles. In the center there is a small rectangle tiled by some white tiles. The small rectangle is surrounded by a red border which is five tiles wide. That red border is surrounded by a white border which is five tiles wide. Finally, the white border is surrounded by a red border which is five tiles wide. The resulting pattern is pictured below. In all, 2900 red tiles are used to tile the large rectangle. Find the perimeter of the large rectangle.

扫码免费领取最新年份学术活动真题及解析