Home » 国际竞赛 » 数学国际竞赛 » AMC美国数学竞赛 » Details

1997AIME 真题及答案解析

Category: AMC美国数学竞赛, 国际竞赛 Date: 2019年12月12日上午10:35

1997AIME 真题及答案解析

答案解析请参考文末

Problem 1

How many of the integers between 1 and 1000, inclusive, can be expressed as the difference of the squares of two nonnegative integers?

Problem 2

The nine horizontal and nine vertical lines on an $8times8$ checkerboard form $r$ rectangles, of which $s$ are squares. The number $s/r$ can be written in the form $m/n,$ where $m$ and $n$ are relatively prime positive integers. Find $m + n.$

Problem 3

Sarah intended to multiply a two-digit number and a three-digit number, but she left out the multiplication sign and simply placed the two-digit number to the left of the three-digit number, thereby forming a five-digit number. This number is exactly nine times the product Sarah should have obtained. What is the sum of the two-digit number and the three-digit number?

Problem 4

Circles of radii 5, 5, 8, and $m/n$ are mutually externally tangent, where $m$ and $n$ are relatively prime positive integers. Find $m + n.$

Problem 5

The number $r$ can be expressed as a four-place decimal $0.abcd,$ where $a, b, c,$ and $d$ represent digits, any of which could be zero. It is desired to approximate $r$ by a fraction whose numerator is 1 or 2 and whose denominator is an integer. The closest such fraction to $r$ is $frac 27.$ What is the number of possible values for $r$ ?

Problem 6

Point $B$ is in the exterior of the regular $n$ -sided polygon $A_1A_2cdots A_n$ , and $A_1A_2B$ is an equilateral triangle. What is the largest value of $n$ for which $A_1$ , $A_n$ , and $B$ are consecutive vertices of a regular polygon?

Problem 7

A car travels due east at $frac 23$ miles per minute on a long, straight road. At the same time, a circular storm, whose radius is $51$ miles, moves southeast at $frac 12sqrt{2}$ mile per minute. At time $t=0$ , the center of the storm is $110$ miles due north of the car. At time $t=t_1$ minutes, the car enters the storm circle, and at time $t=t_2$ minutes, the car leaves the storm circle. Find $frac 12(t_1+t_2)$ .

Problem 8

How many different $4times 4$ arrays whose entries are all 1's and -1's have the property that the sum of the entries in each row is 0 and the sum of the entries in each column is 0?

Problem 9

Given a nonnegative real number $x$ , let $langle xrangle$ denote the fractional part of $x$ ; that is, $langle xrangle=x-lfloor xrfloor$ , where $lfloor xrfloor$ denotes the greatest integer less than or equal to $x$ . Suppose that $a$ is positive, $langle a^{-1}rangle=langle a^2rangle$ , and $2<a^2<3$ . Find the value of $a^{12}-144a^{-1}$ .

Problem 10

Every card in a deck has a picture of one shape - circle, square, or triangle, which is painted in one of the three colors - red, blue, or green. Furthermore, each color is applied in one of three shades - light, medium, or dark. The deck has 27 cards, with every shape-color-shade combination represented. A set of three cards from the deck is called complementary if all of the following statements are true:

i. Either each of the three cards has a different shape or all three of the card have the same shape.

ii. Either each of the three cards has a different color or all three of the cards have the same color.

iii. Either each of the three cards has a different shade or all three of the cards have the same shade.

How many different complementary three-card sets are there?

Problem 11

Let $x=frac{sumlimits_{n=1}^{44} cos n^circ}{sumlimits_{n=1}^{44} sin n^circ}$ . What is the greatest integer that does not exceed $100x$ ?

Problem 12

The function $f$ defined by $f(x)= frac{ax+b}{cx+d}$ , where $a$ , $b$ , $c$ and $d$ are nonzero real numbers, has the properties $f(19)=19$ , $f(97)=97$ and $f(f(x))=x$ for all values except $frac{-d}{c}$ . Find the unique number that is not in the range of $f$ .

Problem 13

Let $S$ be the set of points in the Cartesian plane that satisfy

$Big|big| |x|-2big|-1Big|+Big|big| |y|-2big|-1Big|=1.$ If a model of $S$ were built from wire of negligible thickness, then the total length of wire required would be $asqrt{b}$ , where $a$ and $b$ are positive integers and $b$ is not divisible by the square of any prime number. Find $a+b$ .

Problem 14

Let $v$ and $w$ be distinct, randomly chosen roots of the equation $z^{1997}-1=0$ . Let $m/n$ be the probability that $sqrt{2+sqrt{3}}le |v+w|$ , where $m$ and $n$ are relatively prime positive integers. Find $m+n$ .

Problem 15

The sides of rectangle $ABCD$ have lengths $10$ and $11$ . An equilateral triangle is drawn so that no point of the triangle lies outside $ABCD$ . The maximum possible area of such a triangle can be written in the form $psqrt{q}-r$ , where $p$ , $q$ , and $r$ are positive integers, and $q$ is not divisible by the square of any prime number. Find $p+q+r$ .

1997AIME 详细解析

Notice that all odd numbers can be obtained by using $(a+1)^2-a^2=2a+1,$ where $a$ is a nonnegative integer. All multiples of $4$ can be obtained by using $(b+1)^2-(b-1)^2 = 4b$ , where $b$ is a positive integer. Numbers congruent to $2 pmod 4$ cannot be obtained because squares are $-1, 0, 1 pmod 4.$ Thus, the answer is $500+250 = boxed{750}.$
To determine the two horizontal sides of a rectangle, we have to pick two of the horizontal lines of the checkerboard, or ${9choose 2} = 36$ . Similarily, there are ${9choose 2}$ ways to pick the vertical sides, giving us $r = 1296$ rectangles.For $s$ , there are $8^2$ unit squares, $7^2$ of the $2times2$ squares, and so on until $1^2$ of the $8times 8$ squares. Using the sum of squares formula, that gives us $s=1^2+2^2+cdots+8^2=dfrac{(8)(8+1)(2cdot8+1)}{6}=12*17=204$ .Thus $frac sr = dfrac{204}{1296}=dfrac{17}{108}$ , and $m+n=boxed{125}$ .
Let $x$ be the two-digit number, $y$ be the three-digit number. Putting together the given, we have $1000x+y=9xy Longrightarrow 9xy-1000x-y=0$ . Using SFFT, this factorizes to $(9x-1)left(y-dfrac{1000}{9}right)=dfrac{1000}{9}$ , and $(9x-1)(9y-1000)=1000$ .Since $89 < 9x-1 < 890$ , we can use trial and error on factors of 1000. If $9x - 1 = 100$ , we get a non-integer. If $9x - 1 = 125$ , we get $x=14$ and $y=112$ , which satisifies the conditions. Hence the answer is $112 + 14 = boxed{126}$ .
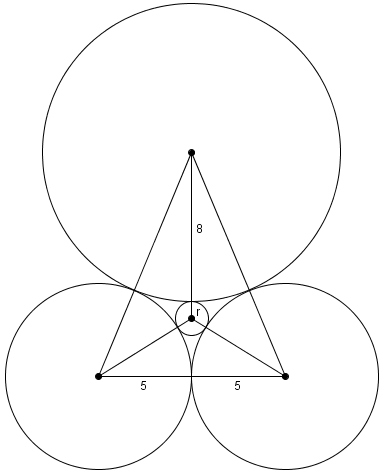If (in the diagram above) we draw the line going through the centers of the circles with radii $8$ and $frac mn = r$ , that line is the perpendicular bisector of the segment connecting the centers of the two circles with radii $5$ . Then we form two right triangles, of lengths $5, x, 5+r$ and $5, 8+r+x, 13$ , wher $x$ is the distance between the center of the circle in question and the segment connecting the centers of the two circles of radii $5$ . By the Pythagorean Theorem, we now have two equations with two unknowns: $begin{eqnarray*} 5^2 + x^2 &=& (5+r)^2 \ x &=& sqrt{10r + r^2} \ && \ (8 + r + sqrt{10r+r^2})^2 + 5^2 &=& 13^2\ 8 + r + sqrt{10r+r^2} &=& 12\ sqrt{10r+r^2}&=& 4-r\ 10r+r^2 &=& 16 - 8r + r^2\ r &=& frac{8}{9} end{eqnarray*}$ So $m+n = boxed{17}$ .
NOTE: It can be seen that there is no apparent need to use the variable x as a 5,12,13 right triangle has been formed.
The nearest fractions to $frac 27$ with numerator $1$ are $frac 13, frac 14$ ; and with numerator $2$ are $frac 26, frac 28 = frac 13, frac 14$ anyway. For $frac 27$ to be the best approximation for $r$ , the decimal must be closer to $frac 27 approx .28571$ than to $frac 13 approx .33333$ or $frac 14 approx .25$ .Thus $r$ can range between $frac{frac 14 + frac{2}{7}}{2} approx .267857$ and $frac{frac 13 + frac{2}{7}}{2} approx .309523$ . At $r = .2679, .3095$ , it becomes closer to the other fractions, so $.2679 le r le .3095$ and the number of values of $r$ is $3095 - 2679 + 1 = boxed{417}$ .
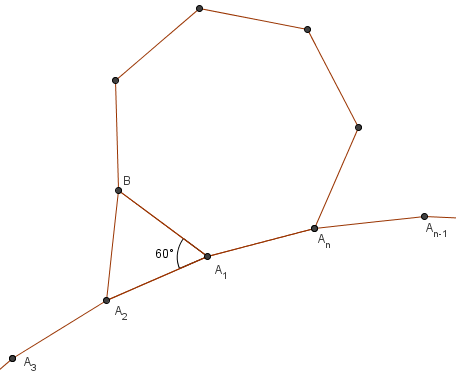Let the other regular polygon have $m$ sides. Using the interior angle of a regular polygon formula, we have $angle A_2A_1A_n = frac{(n-2)180}{n}$ , $angle A_nA_1B = frac{(m-2)180}{m}$ , and $angle A_2A_1B = 60^{circ}$ . Since those three angles add up to $360^{circ}$ , $begin{eqnarray*} frac{(n-2)180}{n} + frac{(m-2)180}{m} &=& 300\ m(n-2)180 + n(m-2)180 &=& 300mn\ 360mn - 360m - 360n &=& 300mn\ mn - 6m - 6n &=& 0 end{eqnarray*}$ Using SFFT, $begin{eqnarray*} (m-6)(n-6) &=& 36 end{eqnarray*}$ Clearly $n$ is maximized when $m = 7, n = boxed{042}$ .
We set up a coordinate system, with the starting point of the car at the origin. At time $t$ , the car is at $left(frac 23t,0right)$ and the center of the storm is at $left(frac{t}{2}, 110 - frac{t}{2}right)$ . Using the distance formula, $begin{eqnarray*} sqrt{left(frac{2}{3}t - frac 12tright)^2 + left(110-frac{t}{2}right)^2} &le& 51\ frac{t^2}{36} + frac{t^2}{4} - 110t + 110^2 &le& 51^2\ frac{5}{18}t^2 - 110t + 110^2 - 51^2 &le& 0\ end{eqnarray*}$ Noting that $frac 12(t_1+t_2)$ is at the maximum point of the parabola, we can use $-frac{b}{2a} = frac{110}{2 cdot frac{5}{18}} = boxed{198}$ .
For more detailed explanations, see related problem (AIME I 2007, 10).

The problem is asking us for all configurations of $4times 4$ grids with 2 1's and 2 -1's in each row and column. We do casework upon the first two columns:
- The first two columns share no two numbers in the same row. There are ${4choose2} = 6$ ways to pick two 1's in the first column, and the second column is determined. For the third and fourth columns, no two numbers can be in the same row (to make the sum of each row 0), so again there are ${4choose 2}$ ways. This gives $6^2 = 36$ .
- The first two columns share one number in the same row. There are ${4choose 1} = 4$ ways to pick the position of the shared 1, then ${3choose 2} = 3$ ways to pick the locations for the next two 1s, and then $2$ ways to orient the 1s. For the third and fourth columns, the two rows with shared 1s or -1s are fixed, so the only things that can be changed is the orientation of the mixed rows, in $2$ ways. This gives $4 cdot 3 cdot 2 cdot 2 = 48$ .
- The first two columns share two numbers in the same row. There are ${4choose 2} = 6$ ways to pick the position of the shared 1s. Everything is then fixed.
Adding these cases up, we get $36 + 48 + 6 = boxed{090}$ .
Looking at the properties of the number, it is immediately guess-able that $a = phi = frac{1+sqrt{5}}2$ (the golden ratio) is the answer. The following is the way to derive that:Since $sqrt{2} < a < sqrt{3}$ , $0 < frac{1}{sqrt{3}} < a^{-1} < frac{1}{sqrt{2}} < 1$ . Thus $langle a^2 rangle = a^{-1}$ , and it follows that $a^2 - 2 = a^{-1} Longrightarrow a^3 - 2a - 1 = 0$ . Noting that $-1$ is a root, this factors to $(a+1)(a^2 - a - 1) = 0$ , so $a = frac{1 pm sqrt{5}}{2}$ (we discard the negative root).Our answer is $(a^2)^{6}-144a^{-1} = left(frac{3+sqrt{5}}2right)^6 - 144left(frac{2}{1 + sqrt{5}}right)$ . Complex conjugates reduce the second term to $-72(sqrt{5}-1)$ . The first term we can expand by the binomial theorem to get $frac 1{2^6}left(3^6 + 6cdot 3^5sqrt{5} + 15cdot 3^4 cdot 5 + 20cdot 3^3 cdot 5sqrt{5} + 15 cdot 3^2 cdot 25 + 6 cdot 3 cdot 25sqrt{5} + 5^3right)$ $= frac{1}{64}left(10304 + 4608sqrt{5}right) = 161 + 72sqrt{5}$ . The answer is $161 + 72sqrt{5} - 72sqrt{5} + 72 = boxed{233}$ .Note that to determine our answer, we could have also used other properties of $phi$ like $phi^3 = 2phi + 1$ .
- Case 1: All three attributes are the same. This is impossible since sets contain distinct cards.
- Case 2: Two of the three attributes are the same. There are ${3choose 2}$ ways to pick the two attributes in question. Then there are $3$ ways to pick the value of the first attribute, $3$ ways to pick the value of the second attribute, and $1$ way to arrange the positions of the third attribute, giving us ${3choose 2} cdot 3 cdot 3 = 27$ ways.
- Case 3: One of the three attributes are the same. There are ${3choose 1}$ ways to pick the one attribute in question, and then $3$ ways to pick the value of that attribute. Then there are $3!$ ways to arrange the positions of the next two attributes, giving us ${3choose 1} cdot 3 cdot 3! = 54$ ways.
- Case 4: None of the three attributes are the same. We fix the order of the first attribute, and then there are $3!$ ways to pick the ordering of the second attribute and $3!$ ways to pick the ordering of the third attribute. This gives us $(3!)^2 = 36$ ways.
Adding the cases up, we get $27 + 54 + 36 = boxed{117}$ .
Note that $frac{sum_{n=1}^{44} cos n}{sum_{n=1}^{44} sin n} = frac {cos 1 + cos 2 + dots + cos 44}{cos 89 + cos 88 + dots + cos 46}$ Now use the sum-product formula $cos x + cos y = 2cos(frac{x+y}{2})cos(frac{x-y}{2})$ We want to pair up $[1, 44]$ , $[2, 43]$ , $[3, 42]$ , etc. from the numerator and $[46, 89]$ , $[47, 88]$ , $[48, 87]$ etc. from the denominator. Then we get: $[frac{sum_{n=1}^{44} cos n}{sum_{n=1}^{44} sin n} = frac{2cos(frac{45}{2})[cos(frac{43}{2})+cos(frac{41}{2})+dots+cos(frac{1}{2})}{2cos(frac{135}{2})[cos(frac{43}{2})+cos(frac{41}{2})+dots+cos(frac{1}{2})} Rightarrow frac{cos(frac{45}{2})}{cos(frac{135}{2})}]$ To calculate this number, use the half angle formula. Since $cos(frac{x}{2}) = pm sqrt{frac{cos x + 1}{2}}$ , then our number becomes: $[frac{sqrt{frac{frac{sqrt{2}}{2} + 1}{2}}}{sqrt{frac{frac{-sqrt{2}}{2} + 1}{2}}}]$ in which we drop the negative roots (as it is clear cosine of $22.5$ and $67.5$ are positive). We can easily simplify this: $begin{eqnarray*} frac{sqrt{frac{frac{sqrt{2}}{2} + 1}{2}}}{sqrt{frac{frac{-sqrt{2}}{2} + 1}{2}}} &=& sqrt{frac{frac{2+sqrt{2}}{4}}{frac{2-sqrt{2}}{4}}} \ &=& sqrt{frac{2+sqrt{2}}{2-sqrt{2}}} cdot sqrt{frac{2+sqrt{2}}{2+sqrt{2}}} \ &=& sqrt{frac{(2+sqrt{2})^2}{2}} \ &=& frac{2+sqrt{2}}{sqrt{2}} cdot sqrt{2} \ &=& sqrt{2}+1 end{eqnarray*}$
And hence our answer is $lfloor 100x rfloor = lfloor 100(1 + sqrt {2}) rfloor = boxed{241}$
First, we use the fact that $f(f(x)) = x$ for all $x$ in the domain. Substituting the function definition, we have $frac {afrac {ax + b}{cx + d} + b}{cfrac {ax + b}{cx + d} + d} = x$ , which reduces to $[frac {(a^2 + bc)x + b(a + d)}{c(a + d)x + (bc + d^2)} =frac {px + q}{rx + s} = x.]$ In order for this fraction to reduce to $x$ , we must have $q = r = 0$ and $p = snot = 0$ . From $c(a + d) = b(a + d) = 0$ , we get $a = - d$ or $b = c = 0$ . The second cannot be true, since we are given that $a,b,c,d$ are nonzero. This means $a = - d$ , so $f(x) = frac {ax + b}{cx - a}$ .The only value that is not in the range of this function is $frac {a}{c}$ . To find $frac {a}{c}$ , we use the two values of the function given to us. We get $2(97)a + b = 97^2c$ and $2(19)a + b = 19^2c$ . Subtracting the second equation from the first will eliminate $b$ , and this results in $2(97 - 19)a = (97^2 - 19^2)c$ , so $[frac {a}{c} = frac {(97 - 19)(97 + 19)}{2(97 - 19)} = 58 .]$ Alternatively, we could have found out that $a = -d$ by using the fact that $f(f(-b/a))=-b/a$ .
This solution is non-rigorous.

Let $f(x) = Big|big||x|-2big|-1Big|$ , $f(x) ge 0$ . Then $f(x) + f(y) = 1 Longrightarrow f(x), f(y) le 1 Longrightarrow x, y le 4$ . We only have a $4times 4$ area, so guessing points and graphing won't be too bad of an idea. Since $f(x) = f(-x)$ , there's a symmetry about all four quadrants, so just consider the first quadrant. We now gather some points:

$f(1) = 0$ $f(0.1) = 0.9$

$f(2) = 1$ $f(0.9) = 0.1$

$f(3) = 0$ $f(1.1) = 0.1$

$f(4) = 1$ $f(1.9) = 0.9$

$f(0.5) = 0.5$ $f(2.1) = 0.9$

$f(1.5) = 1.5$ $f(2.9) = 0.1$

$f(2.5) = 2.5$ $f(3.1) = 0.1$

$f(3.5) = 3.5$ $f(3.9) = 0.9$

We can now graph the pairs of coordinates which add up to $1$ . Just using the first column of information gives us an interesting lattice pattern:

Plotting the remaining points and connecting lines, the graph looks like:

Calculating the lengths is now easy; each rectangle has sides of $sqrt{2}, 3sqrt{2}$ , so the answer is $4(sqrt{2} + 3sqrt{2}) = 16sqrt{2}$ . For all four quadrants, this is $64sqrt{2}$ , and $a+b=boxed{066}$ .
$z^{1997}=1=1(cos 0 + i sin 0)$

By De Moivre's Theorem, we find that ( $k in {0,1,ldots,1996}$ )

$z=cosleft(frac{2pi k}{1997}right)+isinleft(frac{2pi k}{1997}right)$

Now, let $v$ be the root corresponding to $theta=frac{2pi m}{1997}$ , and let $w$ be the root corresponding to $theta=frac{2pi n}{1997}$ . The magnitude of $v+w$ is therefore:

$sqrt{left(cosleft(frac{2pi m}{1997}right) + cosleft(frac{2pi n}{1997}right)right)^2 + left(sinleft(frac{2pi m}{1997}right) + sinleft(frac{2pi n}{1997}right)right)^2}$

$=sqrt{2 + 2cosleft(frac{2pi m}{1997}right)cosleft(frac{2pi n}{1997}right) + 2sinleft(frac{2pi m}{1997}right)sinleft(frac{2pi n}{1997}right)}$

We need $cos left(frac{2pi m}{1997}right)cos left(frac{2pi n}{1997}right) + sin left(frac{2pi m}{1997}right)sin left(frac{2pi n}{1997}right) ge frac{sqrt{3}}{2}$ . The cosine difference identity simplifies that to $cosleft(frac{2pi m}{1997} - frac{2pi n}{1997}right) ge frac{sqrt{3}}{2}$ . Thus, $|m - n| le frac{pi}{6} cdot frac{1997}{2 pi} = lfloor frac{1997}{12} rfloor =166$ .

Therefore, $m$ and $n$ cannot be more than $166$ away from each other. This means that for a given value of $m$ , there are $332$ values for $n$ that satisfy the inequality; $166$ of them $> m$ , and $166$ of them $< m$ . Since $m$ and $n$ must be distinct, $n$ can have $1996$ possible values. Therefore, the probability is $frac{332}{1996}=frac{83}{499}$ . The answer is then $499+83=boxed{582}$ .
Consider points on the complex plane $A (0,0), B (11,0), C (11,10), D (0,10)$ . Since the rectangle is quite close to a square, we figure that the area of the equilateral triangle is maximized when a vertex of the triangle coincides with that of the rectangle. Set one vertex of the triangle at $A$ , and the other two points $E$ and $F$ on $BC$ and $CD$ , respectively. Let $E (11,a)$ and $F (b, 10)$ . Since it's equilateral, then $Ecdottext{cis}60^{circ} = F$ , so $(11 + ai)left(frac {1}{2} + frac {sqrt {3}}{2}iright) = b + 10i$ , and expanding we get $left(frac {11}{2} - frac {asqrt {3}}{2}right) + left(frac {11sqrt {3}}{2} + frac {a}{2}right)i = b + 10i$ .

We can then set the real and imaginary parts equal, and solve for $(a,b) = (20 - 11sqrt {3},22 - 10sqrt {3})$ . Hence a side $s$ of the equilateral triangle can be found by $s^2 = AE^2 = a^2 + AB^2 = 884 - 440sqrt{3}$ . Using the area formula $frac{s^2sqrt{3}}{4}$ , the area of the equilateral triangle is $frac{(884-440sqrt{3})sqrt{3}}{4} = 221sqrt{3} - 330$ . Thus $p + q + r = 221 + 3 + 330 = boxed{554}$ .

$f(1) = 0$	$f(0.1) = 0.9$
$f(2) = 1$	$f(0.9) = 0.1$
$f(3) = 0$	$f(1.1) = 0.1$
$f(4) = 1$	$f(1.9) = 0.9$
$f(0.5) = 0.5$	$f(2.1) = 0.9$
$f(1.5) = 1.5$	$f(2.9) = 0.1$
$f(2.5) = 2.5$	$f(3.1) = 0.1$
$f(3.5) = 3.5$	$f(3.9) = 0.9$