- 翰林提供学术活动、国际课程、科研项目一站式留学背景提升服务!
- 400 888 0080
2001AIME I真题及答案解析
2001AIME I真题及答案解析
答案解析请参考文末
Problem 1
Find the sum of all positive two-digit integers that are divisible by each of their digits.
Problem 2
A finite set ![]() of distinct real numbers has the following properties: the mean of
of distinct real numbers has the following properties: the mean of ![]() is
is ![]() less than the mean of
less than the mean of ![]() , and the mean of
, and the mean of ![]() is
is ![]() more than the mean of
more than the mean of ![]() . Find the mean of
. Find the mean of ![]() .
.
Problem 3
Find the sum of the roots, real and non-real, of the equation ![]() , given that there are no multiple roots.
, given that there are no multiple roots.
Problem 4
In triangle ![]() , angles
, angles ![]() and
and ![]() measure
measure ![]() degrees and
degrees and ![]() degrees, respectively. The bisector of angle
degrees, respectively. The bisector of angle ![]() intersects
intersects ![]() at
at ![]() , and
, and ![]() . The area of triangle
. The area of triangle ![]() can be written in the form
can be written in the form ![]() , where
, where ![]() ,
, ![]() , and
, and ![]() are positive integers, and
are positive integers, and ![]() is not divisible by the square of any prime. Find
is not divisible by the square of any prime. Find ![]() .
.
Problem 5
An equilateral triangle is inscribed in the ellipse whose equation is ![]() . One vertex of the triangle is
. One vertex of the triangle is ![]() , one altitude is contained in the y-axis, and the length of each side is
, one altitude is contained in the y-axis, and the length of each side is ![]() , where
, where ![]() and
and ![]() are relatively prime positive integers. Find
are relatively prime positive integers. Find ![]() .
.
Problem 6
A fair die is rolled four times. The probability that each of the final three rolls is at least as large as the roll preceding it may be expressed in the form ![]() , where
, where ![]() and
and ![]() are relatively prime positive integers. Find
are relatively prime positive integers. Find ![]() .
.
Problem 7
Triangle ![]() has
has ![]() ,
, ![]() and
and ![]() . Points
. Points ![]() and
and ![]() are located on
are located on ![]() and
and ![]() , respectively, such that
, respectively, such that ![]() is parallel to
is parallel to ![]() and contains the center of the inscribed circle of triangle
and contains the center of the inscribed circle of triangle ![]() . Then
. Then ![]() , where
, where ![]() and
and ![]() are relatively prime positive integers. Find
are relatively prime positive integers. Find ![]() .
.
Problem 8
Call a positive integer ![]() a
a ![]() if the digits of the base-7 representation of
if the digits of the base-7 representation of ![]() form a base-10 number that is twice
form a base-10 number that is twice ![]() . For example,
. For example, ![]() is a 7-10 double because its base-7 representation is
is a 7-10 double because its base-7 representation is ![]() . What is the largest 7-10 double?
. What is the largest 7-10 double?
Problem 9
In triangle ![]() ,
, ![]() ,
, ![]() and
and ![]() . Point
. Point ![]() is on
is on ![]() ,
, ![]() is on
is on ![]() , and
, and ![]() is on
is on ![]() . Let
. Let ![]() ,
, ![]() , and
, and ![]() , where
, where ![]() ,
, ![]() , and
, and ![]() are positive and satisfy
are positive and satisfy ![]() and
and ![]() . The ratio of the area of triangle
. The ratio of the area of triangle ![]() to the area of triangle
to the area of triangle ![]() can be written in the form
can be written in the form ![]() , where
, where ![]() and
and ![]() are relatively prime positive integers. Find
are relatively prime positive integers. Find ![]() .
.
Problem 10
Let ![]() be the set of points whose coordinates
be the set of points whose coordinates ![]()
![]() and
and ![]() are integers that satisfy
are integers that satisfy ![]()
![]() and
and ![]() Two distinct points are randomly chosen from
Two distinct points are randomly chosen from ![]() The probability that the midpoint of the segment they determine also belongs to
The probability that the midpoint of the segment they determine also belongs to ![]() is
is ![]() where
where ![]() and
and ![]() are relatively prime positive integers. Find
are relatively prime positive integers. Find ![]()
Problem 11
In a rectangular array of points, with 5 rows and ![]() columns, the points are numbered consecutively from left to right beginning with the top row. Thus the top row is numbered 1 through
columns, the points are numbered consecutively from left to right beginning with the top row. Thus the top row is numbered 1 through ![]() the second row is numbered
the second row is numbered ![]() through
through ![]() and so forth. Five points,
and so forth. Five points, ![]() and
and ![]() are selected so that each
are selected so that each ![]() is in row
is in row ![]() Let
Let ![]() be the number associated with
be the number associated with ![]() Now renumber the array consecutively from top to bottom, beginning with the first column. Let
Now renumber the array consecutively from top to bottom, beginning with the first column. Let ![]() be the number associated with
be the number associated with ![]() after the renumbering. It is found that
after the renumbering. It is found that ![]()
![]()
![]()
![]() and
and ![]() Find the smallest possible value of
Find the smallest possible value of ![]()
Problem 12
A sphere is inscribed in the tetrahedron whose vertices are ![]() and
and ![]() The radius of the sphere is
The radius of the sphere is ![]() where
where ![]() and
and ![]() are relatively prime positive integers. Find
are relatively prime positive integers. Find ![]()
Problem 13
In a certain circle, the chord of a ![]() -degree arc is 22 centimeters long, and the chord of a
-degree arc is 22 centimeters long, and the chord of a ![]() -degree arc is 20 centimeters longer than the chord of a
-degree arc is 20 centimeters longer than the chord of a ![]() -degree arc, where
-degree arc, where ![]() The length of the chord of a
The length of the chord of a ![]() -degree arc is
-degree arc is ![]() centimeters, where
centimeters, where ![]() and
and ![]() are positive integers. Find
are positive integers. Find ![]()
Problem 14
A mail carrier delivers mail to the nineteen houses on the east side of Elm Street. The carrier notices that no two adjacent houses ever get mail on the same day, but that there are never more than two houses in a row that get no mail on the same day. How many different patterns of mail delivery are possible?
Problem 15
The numbers 1, 2, 3, 4, 5, 6, 7, and 8 are randomly written on the faces of a regular octahedron so that each face contains a different number. The probability that no two consecutive numbers, where 8 and 1 are considered to be consecutive, are written on faces that share an edge is ![]() where
where ![]() and
and ![]() are relatively prime positive integers. Find
are relatively prime positive integers. Find ![]()
2001AIME I详细解析
- Let our number be
 ,
,  . Then we have two conditions:
. Then we have two conditions:  and
and  , or
, or  divides into
divides into  and
and  divides into
divides into  . Thus
. Thus  or
or  (note that if
(note that if  , then
, then  would not be a digit).
would not be a digit).
- For
 , we have
, we have  for nine possibilities, giving us a sum of
for nine possibilities, giving us a sum of  .
. - For
 , we have
, we have  for four possibilities (the higher ones give
for four possibilities (the higher ones give  ), giving us a sum of
), giving us a sum of  .
. - For
 , we have
, we have  for one possibility (again, higher ones give
for one possibility (again, higher ones give  ), giving us a sum of
), giving us a sum of  .
.
If we ignore the case
 as we have been doing so far, then the sum is
as we have been doing so far, then the sum is  .
. - For
- Let
 be the mean of
be the mean of  . Let
. Let  be the number of elements in
be the number of elements in  . Then, the given tells us that
. Then, the given tells us that  and
and  . Subtracting, we have
. Subtracting, we have We plug that into our very first formula, and get:
We plug that into our very first formula, and get:
- From Vieta's formulas, in a polynomial of the form
 , then the sum of the roots is
, then the sum of the roots is  .From the Binomial Theorem, the first term of
.From the Binomial Theorem, the first term of  is
is  , but
, but  , so the term with the largest degree is
, so the term with the largest degree is  . So we need the coefficient of that term, as well as the coefficient of
. So we need the coefficient of that term, as well as the coefficient of  .
. Applying Vieta's formulas, we find that the sum of the roots is
Applying Vieta's formulas, we find that the sum of the roots is  .We find that the given equation has a
.We find that the given equation has a  degree polynomial. Note that there are no multiple roots. Thus, if
degree polynomial. Note that there are no multiple roots. Thus, if  is a root,
is a root,  is also a root. Thus, we pair up
is also a root. Thus, we pair up  pairs of roots that sum to
pairs of roots that sum to  to get a sum of
to get a sum of  .
. - After chasing angles,
 and
and  , meaning
, meaning  is an isosceles triangle and
is an isosceles triangle and  .Using law of sines on
.Using law of sines on  , we can create the following equation:
, we can create the following equation:


 and
and  , so
, so  .We can then use the Law of Sines area formula
.We can then use the Law of Sines area formula  to find the area of the triangle.
to find the area of the triangle.
 can be found through the sin addition formula.
can be found through the sin addition formula.


Therefore, the area of the triangle is










- Denote the vertices of the triangle
 and
and  where
where  is in quadrant 4 and
is in quadrant 4 and  is in quadrant
is in quadrant  Note that the slope of
Note that the slope of  is
is  Hence, the equation of the line containing
Hence, the equation of the line containing  is
is![[y = xsqrt {3} + 1.]](https://latex.artofproblemsolving.com/6/4/7/647cf5f03f729a69f551bc8dfd47692937940287.png) This will intersect the ellipse when
This will intersect the ellipse when We ignore the
We ignore the  solution because it is not in quadrant 3.Since the triangle is symmetric with respect to the y-axis, the coordinates of
solution because it is not in quadrant 3.Since the triangle is symmetric with respect to the y-axis, the coordinates of  and
and  are now
are now  and
and  respectively, for some value of
respectively, for some value of  It is clear that the value of
It is clear that the value of  is irrelevant to the length of
is irrelevant to the length of  . Our answer is
. Our answer is![[BC = 2*frac {8sqrt {3}}{13}=sqrt {4left(frac {8sqrt {3}}{13}right)^{2}} = sqrt {frac {768}{169}}implies m + n = boxed{937}.]](https://latex.artofproblemsolving.com/2/9/b/29b9d7afe82598f64d5bca3ec2347f63ae6245c4.png)
- Recast the problem entirely as a block-walking problem. Call the respective dice
 . In the diagram below, the lowest
. In the diagram below, the lowest  -coordinate at each of
-coordinate at each of  ,
,  ,
,  , and
, and  corresponds to the value of the roll.
corresponds to the value of the roll.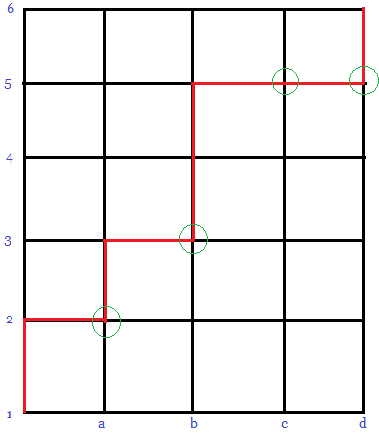 The red path corresponds to the sequence of rolls
The red path corresponds to the sequence of rolls  . This establishes a one-to-one correspondence between valid dice roll sequences and block walking paths.The solution to this problem is therefore
. This establishes a one-to-one correspondence between valid dice roll sequences and block walking paths.The solution to this problem is therefore  . So the answer is
. So the answer is  .
. ![[asy] pointpen = black; pathpen = black+linewidth(0.7); pair B=(0,0), C=(20,0), A=IP(CR(B,21),CR(C,22)), I=incenter(A,B,C), D=IP((0,I.y)--(20,I.y),A--B), E=IP((0,I.y)--(20,I.y),A--C); D(MP("A",A,N)--MP("B",B)--MP("C",C)--cycle); D(MP("I",I,NE)); D(MP("E",E,NE)--MP("D",D,NW)); // D((A.x,0)--A,linetype("4 4")+linewidth(0.7)); D((I.x,0)--I,linetype("4 4")+linewidth(0.7)); D(rightanglemark(B,(A.x,0),A,30)); D(B--I--C); MP("20",(B+C)/2); MP("21",(A+B)/2,NW); MP("22",(A+C)/2,NE); [/asy]](https://latex.artofproblemsolving.com/a/e/4/ae47e3711da65c309f6b7dc3c0e55287b8c8a5fc.png)
 be the incenter of
be the incenter of  , so that
, so that  and
and  are angle bisectors of
are angle bisectors of  and
and  respectively. Then,
respectively. Then,  so
so  is isosceles, and similarly
is isosceles, and similarly  is isosceles. It follows that
is isosceles. It follows that  , so the perimeter of
, so the perimeter of  is
is  . Hence, the ratio of the perimeters of
. Hence, the ratio of the perimeters of  and
and  is
is  , which is the scale factor between the two similar triangles, and thus
, which is the scale factor between the two similar triangles, and thus  . Thus,
. Thus,  .
.- We let
 ; we are given that
; we are given that![[2(a_na_{n-1}cdots a_0)_7 = (a_na_{n-1}cdots a_0)_{10}]](https://latex.artofproblemsolving.com/8/0/e/80e28e31aaf18ab0eafe5466283a750f9e062edd.png) m (This is because the digits in
m (This is because the digits in  ' s base 7 representation make a number with the same digits in base 10 when multiplied by 2)Expanding, we find that
' s base 7 representation make a number with the same digits in base 10 when multiplied by 2)Expanding, we find that![[2 cdot 7^n a_n + 2 cdot 7^{n-1} a_{n-1} + cdots + 2a_0 = 10^na_n + 10^{n-1}a_{n-1} + cdots + a_0]](https://latex.artofproblemsolving.com/8/3/c/83c32c1f5624df09afd480557f3483b8f0ed4190.png) or re-arranging,
or re-arranging,
![[a_0 + 4a_1 = 2a_2 + 314a_3 + cdots + (10^n - 2 cdot 7^n)a_n]](https://latex.artofproblemsolving.com/4/5/7/4574738c53d25fa109c96f5340f1e386649da59d.png)
Since the
 s are base-
s are base- digits, it follows that
digits, it follows that  , and the LHS is less than or equal to
, and the LHS is less than or equal to  . Hence our number can have at most
. Hence our number can have at most  digits in base-
digits in base- . Letting
. Letting  , we find that
, we find that  is our largest 7-10 double.
is our largest 7-10 double. ![[asy] /* -- arbitrary values, I couldn't find nice values for pqr please replace if possible -- */ real p = 0.5, q = 0.1, r = 0.05; /* -- arbitrary values, I couldn't find nice values for pqr please replace if possible -- */ pointpen = black; pathpen = linewidth(0.7) + black; pair A=(0,0),B=(13,0),C=IP(CR(A,17),CR(B,15)), D=A+p*(B-A), E=B+q*(C-B), F=C+r*(A-C); D(D(MP("A",A))--D(MP("B",B))--D(MP("C",C,N))--cycle); D(D(MP("D",D))--D(MP("E",E,NE))--D(MP("F",F,NW))--cycle); [/asy]](https://latex.artofproblemsolving.com/a/a/5/aa532b18510960c694fe8213246465f219c7d942.png)
![$[ldots]$](https://latex.artofproblemsolving.com/7/1/0/710131907cfe6ede3e2195a1a5c68cffb5b203f4.png) denote area; then the desired value is
denote area; then the desired value is![$frac mn = frac{[DEF]}{[ABC]} = frac{[ABC] - [ADF] - [BDE] - [CEF]}{[ABC]}$](https://latex.artofproblemsolving.com/f/0/3/f031606bd0480fc8cb6ee9da5a70ec5dccdfcecb.png)
 , we find that
, we find that![$frac{[ADF]}{[ABC]} = frac{frac 12 cdot p cdot AB cdot (1-r) cdot AC cdot sin angle CAB}{frac 12 cdot AB cdot AC cdot sin angle CAB} = p(1-r)$](https://latex.artofproblemsolving.com/c/c/2/cc254e39ee583e6f0e7845a84cb9f1e473868472.png)
![$frac{[BDE]}{[ABC]} = q(1-p)$](https://latex.artofproblemsolving.com/3/1/4/314ac41a75f721b95a9cc9154ec38dc920614ec0.png) and
and ![$frac{[CEF]}{[ABC]} = r(1-q)$](https://latex.artofproblemsolving.com/f/3/8/f38dafeb4a3f76d640a9f865c68e5a6f001de43a.png) . Thus, we wish to find
. Thus, we wish to find![begin{align*}frac{[DEF]}{[ABC]} &= 1 - frac{[ADF]}{[ABC]} - frac{[BDE]}{[ABC]} - frac{[CEF]}{[ABC]} \ &= 1 - p(1-r) - q(1-p) - r(1-q)\ &= (pq + qr + rp) - (p + q + r) + 1 end{align*}](https://latex.artofproblemsolving.com/3/8/a/38a66c816b5f7aede400644c7f710603718a56f1.png) We know that
We know that  , and also that
, and also that  . Substituting, the answer is
. Substituting, the answer is  , and
, and  .
.- The distance between the
 ,
,  , and
, and  coordinates must be even so that the midpoint can have integer coordinates. Therefore,
coordinates must be even so that the midpoint can have integer coordinates. Therefore,
- For
 , we have the possibilities
, we have the possibilities  ,
,  ,
,  ,
,  , and
, and  ,
,  possibilities.
possibilities. - For
 , we have the possibilities
, we have the possibilities  ,
,  ,
,  ,
,  ,
,  ,
,  ,
,  , and
, and  ,
,  possibilities.
possibilities. - For
 , we have the possibilities
, we have the possibilities  ,
,  ,
,  ,
,  ,
,  ,
,  ,
,  ,
,  ,
,  ,
,  ,
,  ,
,  , and
, and  ,
,  possibilities.
possibilities.
However, we have
 cases where we have simply taken the same point twice, so we subtract those. Therefore, our answer is
cases where we have simply taken the same point twice, so we subtract those. Therefore, our answer is  .
. - For
- Let each point
 be in column
be in column  . The numberings for
. The numberings for  can now be defined as follows.
can now be defined as follows. We can now convert the five given equalities.
We can now convert the five given equalities. Equations
Equations  and
and  combine to form
combine to form![[N = 24c_2 - 19]](https://latex.artofproblemsolving.com/8/c/0/8c0aa65792f67c78862bb1083444f38fd0e6b590.png) Similarly equations
Similarly equations  ,
,  , and
, and  combine to form
combine to form![[117N +51 = 124c_3]](https://latex.artofproblemsolving.com/e/e/6/ee6cd01627090227c45a3e3ee787dbb771070220.png) Take this equation modulo 31
Take this equation modulo 31![[24N+20equiv 0 pmod{31}]](https://latex.artofproblemsolving.com/e/8/c/e8c350296fd13dab4773e30b3daaff14e6676891.png) And substitute for N
And substitute for N![[24 cdot 24 c_2 - 24 cdot 19 +20equiv 0 pmod{31}]](https://latex.artofproblemsolving.com/e/0/4/e0442e2a0d7ae32f22e5d309988184465edb7841.png)
![[18 c_2 equiv 2 pmod{31}]](https://latex.artofproblemsolving.com/9/8/9/98907cca004b6027b057c7a0ed5bd6548d25a10f.png) Thus the smallest
Thus the smallest  might be is
might be is  and by substitution
and by substitution  The column values can also easily be found by substitution
The column values can also easily be found by substitution As these are all positive and less than
As these are all positive and less than  ,
,  is the solution.
is the solution. ![[asy] import three; currentprojection = perspective(-2,9,4); triple A = (6,0,0), B = (0,4,0), C = (0,0,2), D = (0,0,0); triple E = (2/3,0,0), F = (0,2/3,0), G = (0,0,2/3), L = (0,2/3,2/3), M = (2/3,0,2/3), N = (2/3,2/3,0); triple I = (2/3,2/3,2/3); triple J = (6/7,20/21,26/21); draw(C--A--D--C--B--D--B--A--C); draw(L--F--N--E--M--G--L--I--M--I--N--I--J); label("$I$",I,W); label("$A$",A,S); label("$B$",B,S); label("$C$",C,W*-1); label("$D$",D,W*-1); [/asy]](https://latex.artofproblemsolving.com/a/d/5/ad592fa5d796f97d2c229a092105e33dc3dfc1ed.png) The center
The center  of the insphere must be located at
of the insphere must be located at  where
where  is the sphere's radius.
is the sphere's radius.  must also be a distance
must also be a distance  from the plane
from the plane  The signed distance between a plane and a point
The signed distance between a plane and a point  can be calculated as
can be calculated as  , where G is any point on the plane, and P is a vector perpendicular to ABC.A vector
, where G is any point on the plane, and P is a vector perpendicular to ABC.A vector  perpendicular to plane
perpendicular to plane  can be found as
can be found as  Thus
Thus  where the negative comes from the fact that we want
where the negative comes from the fact that we want  to be in the opposite direction of
to be in the opposite direction of 

Finally


 -degree arcs and one chord of one
-degree arcs and one chord of one  -degree arc. The diagonals of this trapezoid turn out to be two chords of two
-degree arc. The diagonals of this trapezoid turn out to be two chords of two  -degree arcs. Let
-degree arcs. Let  ,
,  , and
, and  be the chords of the
be the chords of the  -degree arcs, and let
-degree arcs, and let  be the chord of the
be the chord of the  -degree arc. Also let
-degree arc. Also let  be equal to the chord length of the
be equal to the chord length of the  -degree arc. Hence, the length of the chords,
-degree arc. Hence, the length of the chords,  and
and  , of the
, of the  -degree arcs can be represented as
-degree arcs can be represented as  , as given in the problem.Using Ptolemy's theorem,
, as given in the problem.Using Ptolemy's theorem,![[AB(CD) + AC(BD) = AD(BC)]](https://latex.artofproblemsolving.com/e/4/9/e49713edcd6de6361f8d5ecf5df57120b91b00e0.png)
![[22x + 22(22) = (x + 20)^2]](https://latex.artofproblemsolving.com/7/3/d/73d80bfed17d4f79cb1eb5e69a10849cd802677f.png)
![[22x + 484 = x^2 + 40x + 400]](https://latex.artofproblemsolving.com/e/2/3/e231d9b344d55300e625575317f4da19463a6f1a.png)
![[0 = x^2 + 18x - 84]](https://latex.artofproblemsolving.com/f/1/5/f15dd545e67e4393fd29d2a684400d699c39d06d.png) We can then apply the quadratic formula to find the positive root to this equation since polygons obviously cannot have sides of negative length.
We can then apply the quadratic formula to find the positive root to this equation since polygons obviously cannot have sides of negative length.![[x = frac{-18 + sqrt{18^2 + 4(84)}}{2}]](https://latex.artofproblemsolving.com/b/9/5/b95cc316dc3871bbea07f30f421c37e37867f7a8.png)
![[x = frac{-18 + sqrt{660}}{2}]](https://latex.artofproblemsolving.com/7/1/b/71b7033bbcda8f696de0b52090b9fa78467c4937.png)
 simplifies to
simplifies to  which equals
which equals  Thus, the answer is
Thus, the answer is  .
.- Let
 represent a house that does not receive mail and
represent a house that does not receive mail and  represent a house that does receive mail. This problem is now asking for the number of
represent a house that does receive mail. This problem is now asking for the number of  -digit strings of
-digit strings of  's and
's and  's such that there are no two consecutive
's such that there are no two consecutive  's and no three consecutive
's and no three consecutive  's.The last two digits of any
's.The last two digits of any  -digit string can't be
-digit string can't be  , so the only possibilities are
, so the only possibilities are  ,
,  , and
, and  .Let
.Let  be the number of
be the number of  -digit strings ending in
-digit strings ending in  ,
,  be the number of
be the number of  -digit strings ending in
-digit strings ending in  , and
, and  be the number of
be the number of  -digit strings ending in
-digit strings ending in  .If an
.If an  -digit string ends in
-digit string ends in  , then the previous digit must be a
, then the previous digit must be a  , and the last two digits of the
, and the last two digits of the  digits substring will be
digits substring will be  . So
. So![[a_{n} = c_{n-1}.]](https://latex.artofproblemsolving.com/6/8/1/68108633125c7bf502f39833be52b610d63efc09.png) If an
If an  -digit string ends in
-digit string ends in  , then the previous digit can be either a
, then the previous digit can be either a  or a
or a  , and the last two digits of the
, and the last two digits of the  digits substring can be either
digits substring can be either  or
or  . So
. So![[b_{n} = a_{n-1} + c_{n-1}.]](https://latex.artofproblemsolving.com/9/8/a/98adae5c08bd20b780cf60980dbe6f4bdb23557e.png)
If an
 -digit string ends in
-digit string ends in  , then the previous digit must be a
, then the previous digit must be a  , and the last two digits of the
, and the last two digits of the  digits substring will be
digits substring will be  . So
. So![[c_{n} = b_{n-1}.]](https://latex.artofproblemsolving.com/a/3/9/a390dc2649eb35e214f0c1c26dc68c40f7d551a2.png)
Clearly,
 . Using the recursive equations and initial values:
. Using the recursive equations and initial values:![[begin{array}{|c|c|c|c|c|c|c|c|c|c|c|c|c|c|c|c|c|c|c|} multicolumn{19}{c}{}\hline n&2&3&4&5&6&7&8&9&10&11&12&13&14&15&16&17&18&19\hline a_n&1&1&1&2&2&3&4&5&7&9&12&16&21&28&37&49&65&86\hline b_n&1&2&2&3&4&5&7&9&12&16&21&28&37&49&65&86&114&151\hline c_n&1&1&2&2&3&4&5&7&9&12&16&21&28&37&49&65&86&114\hline end{array}]](https://latex.artofproblemsolving.com/c/4/8/c48c70341c61ea0d217ee0af46832bf101da97af.png)
As a result
 .
. - Choose one face of the octahedron randomly and label it with
 . There are three faces adjacent to this one, which we will call A-faces. There are three faces adjacent to two of the A-faces, which we will call B-faces, and one face adjacent to the three B-faces, which we will call the C-face.Clearly, the labels for the A-faces must come from the set
. There are three faces adjacent to this one, which we will call A-faces. There are three faces adjacent to two of the A-faces, which we will call B-faces, and one face adjacent to the three B-faces, which we will call the C-face.Clearly, the labels for the A-faces must come from the set  , since these faces are all adjacent to
, since these faces are all adjacent to  . There are thus
. There are thus  ways to assign the labels for the A-faces.The labels for the B-faces and C-face are the two remaining numbers from the above set, plus
ways to assign the labels for the A-faces.The labels for the B-faces and C-face are the two remaining numbers from the above set, plus  and
and  . The number on the C-face must not be consecutive to any of the numbers on the B-faces.From here it is easiest to brute force the
. The number on the C-face must not be consecutive to any of the numbers on the B-faces.From here it is easiest to brute force the  possibilities for the
possibilities for the  numbers on the B and C faces:
numbers on the B and C faces:
- 2348 (2678): 8(2) is the only one not adjacent to any of the others, so it goes on the C-face. 4(6) has only one B-face it can go to, while 2 and 3 (7 and 8) can be assigned randomly to the last two. 2 possibilities here.
- 2358 (2578): 5 cannot go on any of the B-faces, so it must be on the C-face. 3 and 8 (2 and 7) have only one allowable B-face, so just 1 possibility here.
- 2368 (2478): 6(4) cannot go on any of the B-faces, so it must be on the C-face. 3 and 8 (2 and 7) have only one allowable B-face, so 1 possibility here.
- 2458 (2568): All of the numbers have only one B-face they could go to. 2 and 4 (6 and 8) can go on the same, so one must go to the C-face. Only 2(8) is not consecutive with any of the others, so it goes on the C-face. 1 possibility.
- 2378: None of the numbers can go on the C-face because they will be consecutive with one of the B-face numbers. So this possibility is impossible.
- 2468: Both 4 and 6 cannot go on any B-face. They cannot both go on the C-face, so this possibility is impossible.
There is a total of
 possibilities. There are
possibilities. There are  permutations (more like "rotations") of each, so
permutations (more like "rotations") of each, so  acceptable ways to fill in the rest of the octahedron given the
acceptable ways to fill in the rest of the octahedron given the  . There are
. There are  ways to randomly fill in the rest of the octahedron. So the probability is
ways to randomly fill in the rest of the octahedron. So the probability is  . The answer is
. The answer is  .
.
以上解析方式仅供参考
学术活动报名扫码了解!免费领取历年真题!
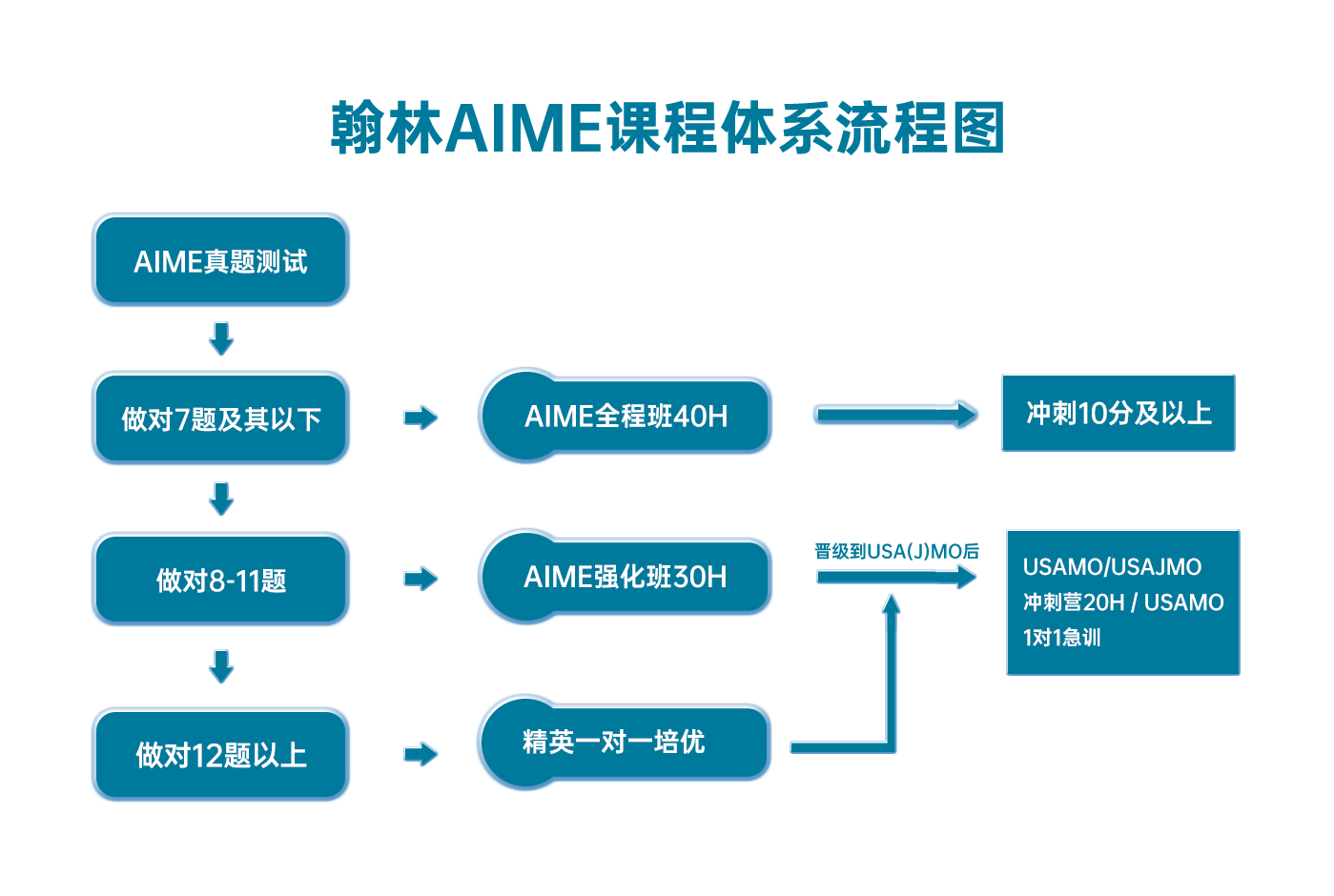
翰林AIME课程体系流程图

早鸟钜惠!翰林2025暑期班课上线

最新发布
© 2025. All Rights Reserved. 沪ICP备2023009024号-1








