- 翰林提供学术活动、国际课程、科研项目一站式留学背景提升服务!
- 400 888 0080
2004 AMC12B 真题及答案详细解析
2004 AMC 12B 真题
Problem 1
At each basketball practice last week, Jenny made twice as many free throws as she made at the previous practice. At her fifth practice she made 48 free throws. How many free throws did she make at the first practice?
![]()
Problem 2
In the expression ![]() , the values of
, the values of ![]() ,
, ![]() ,
, ![]() , and
, and ![]() are 0, 1, 2, and 3, although not necessarily in that order. What is the maximum possible value of the result?
are 0, 1, 2, and 3, although not necessarily in that order. What is the maximum possible value of the result?
![]()
Problem 3
If ![]() and
and ![]() are positive integers for which
are positive integers for which ![]() , what is the value of
, what is the value of ![]() ?
?
![]()
Problem 4
An integer ![]() , with
, with ![]() , is to be chosen. If all choices are equally likely, what is the probability that at least one digit of
, is to be chosen. If all choices are equally likely, what is the probability that at least one digit of ![]() is a 7?
is a 7?
![]()
Problem 5
On a trip from the United States to Canada, Isabella took ![]() U.S. dollars. At the border she exchanged them all, receiving 10 Canadian dollars for every 7 U.S. dollars. After spending 60 Canadian dollars, she had
U.S. dollars. At the border she exchanged them all, receiving 10 Canadian dollars for every 7 U.S. dollars. After spending 60 Canadian dollars, she had ![]() Canadian dollars left. What is the sum of the digits of
Canadian dollars left. What is the sum of the digits of ![]() ?
?
![]()
Problem 6
Minneapolis-St. Paul International Airport is 8 miles southwest of downtown St. Paul and 10 miles southeast of downtown Minneapolis. Which of the following is closest to the number of miles between downtown St. Paul and downtown Minneapolis?
![]()
Problem 7
A square has sides of length 10, and a circle centered at one of its vertices has radius 10. What is the area of the union of the regions enclosed by the square and the circle?
![]()
Problem 8
A grocer makes a display of cans in which the top row has one can and each lower row has two more cans than the row above it. If the display contains 100 cans, how many rows does it contain?
![]()
Problem 9
The point ![]() is rotated
is rotated ![]() clockwise around the origin to point
clockwise around the origin to point ![]() . Point
. Point ![]() is then reflected over the line
is then reflected over the line ![]() to point
to point ![]() . What are the coordinates of
. What are the coordinates of ![]() ?
?
![]()
Problem 10
An annulus is the region between two concentric circles. The concentric circles in the figure have radii ![]() and
and ![]() , with
, with ![]() . Let
. Let ![]() be a radius of the larger circle, let
be a radius of the larger circle, let ![]() be tangent to the smaller circle at
be tangent to the smaller circle at ![]() , and let
, and let ![]() be the radius of the larger circle that contains
be the radius of the larger circle that contains ![]() . Let
. Let ![]() ,
, ![]() , and
, and ![]() . What is the area of the annulus?
. What is the area of the annulus?
![[asy] import graph; unitsize(1.5cm); defaultpen(0.8); real r1=1.5, r2=2.5; pair O=(0,0); path inner=Circle(O,r1), outer=Circle(O,r2); pair Y=(0,r2), Z=(0,r1), X=intersectionpoint( Z--(Z+(10,0)), outer ); filldraw(outer,lightgray,black); filldraw(inner,white,black); draw(X--O--Y); draw(Y--X--Z); label("$O$",O,SW); label("$X$",X,E); label("$Y$",Y,N); label("$Z$",Z,SW); label("$a$",X--Z,N); label("$b$",0.25*X,SE); label("$c$",O--Z,E); label("$d$",Y--Z,W); label("$e$",Y*0.65 + X*0.35,SW); defaultpen(0.5); dot(O); dot(X); dot(Z); dot(Y); [/asy]](https://latex.artofproblemsolving.com/e/8/1/e810cc7b6572d11a1cb77c70ea93bf73a9411484.png)
![]()
Problem 11
All the students in an algebra class took a ![]() -point test. Five students scored
-point test. Five students scored ![]() , each student scored at least
, each student scored at least ![]() , and the mean score was
, and the mean score was ![]() . What is the smallest possible number of students in the class?
. What is the smallest possible number of students in the class?
![]()
Problem 12
In the sequence ![]() ,
, ![]() ,
, ![]() ,
, ![]() , each term after the third is found by subtracting the previous term from the sum of the two terms that precede that term. For example, the fourth term is
, each term after the third is found by subtracting the previous term from the sum of the two terms that precede that term. For example, the fourth term is ![]() . What is the
. What is the ![]() term in this sequence?
term in this sequence?
![]()
Problem 13
If ![]() and
and ![]() with
with ![]() and
and ![]() real, what is the value of
real, what is the value of ![]() ?
?
![]()
Problem 14
In ![]() ,
, ![]() ,
, ![]() , and
, and ![]() . Points
. Points ![]() and
and ![]() lie on
lie on ![]() and
and ![]() , respectively, with
, respectively, with ![]() . Points
. Points ![]() and
and ![]() are on
are on ![]() so that
so that ![]() and
and ![]() are perpendicular to
are perpendicular to ![]() . What is the area of pentagon
. What is the area of pentagon ![]() ?
?
![[asy] unitsize(0.5cm); defaultpen(0.8); pair C=(0,0), A=(0,5), B=(12,0), M=(0,4), N=(4,0); pair J=intersectionpoint(A--B, M--(M+rotate(90)*(B-A)) ); pair K=intersectionpoint(A--B, N--(N+rotate(90)*(B-A)) ); draw( A--B--C--cycle ); draw( M--J ); draw( N--K ); label("$A$",A,NW); label("$B$",B,SE); label("$C$",C,SW); label("$M$",M,SW); label("$N$",N,S); label("$J$",J,NE); label("$K$",K,NE); [/asy]](https://latex.artofproblemsolving.com/a/0/5/a0578d737aee0aa9a0c6e400c18538bade106520.png)
![]()
Problem 15
The two digits in Jack's age are the same as the digits in Bill's age, but in reverse order. In five years Jack will be twice as old as Bill will be then. What is the difference in their current ages?
![]()
Problem 16
A function ![]() is defined by
is defined by ![]() , where
, where ![]() and
and ![]() is the complex conjugate of
is the complex conjugate of ![]() . How many values of
. How many values of ![]() satisfy both
satisfy both ![]() and
and ![]() ?
?
![]()
Problem 17
For some real numbers ![]() and
and ![]() , the equation
, the equation![]() has three distinct positive roots. If the sum of the base-
has three distinct positive roots. If the sum of the base-![]() logarithms of the roots is
logarithms of the roots is ![]() , what is the value of
, what is the value of ![]() ?
?
![]()
Problem 18
Points ![]() and
and ![]() are on the parabola
are on the parabola ![]() , and the origin is the midpoint of
, and the origin is the midpoint of ![]() . What is the length of
. What is the length of ![]() ?
?
![]()
Problem 19
A truncated cone has horizontal bases with radii ![]() and
and ![]() . A sphere is tangent to the top, bottom, and lateral surface of the truncated cone. What is the radius of the sphere?
. A sphere is tangent to the top, bottom, and lateral surface of the truncated cone. What is the radius of the sphere?
![]()
Problem 20
Each face of a cube is painted either red or blue, each with probability ![]() . The color of each face is determined independently. What is the probability that the painted cube can be placed on a horizontal surface so that the four vertical faces are all the same color?
. The color of each face is determined independently. What is the probability that the painted cube can be placed on a horizontal surface so that the four vertical faces are all the same color?
![]()
Problem 21
The graph of ![]() is an ellipse in the first quadrant of the
is an ellipse in the first quadrant of the ![]() -plane. Let
-plane. Let ![]() and
and ![]() be the maximum and minimum values of
be the maximum and minimum values of ![]() over all points
over all points ![]() on the ellipse. What is the value of
on the ellipse. What is the value of ![]() ?
?
![]()
Problem 22
The square

![]()
Problem 23
The polynomial ![]() has integer coefficients and three distinct positive zeros. Exactly one of these is an integer, and it is the sum of the other two. How many values of
has integer coefficients and three distinct positive zeros. Exactly one of these is an integer, and it is the sum of the other two. How many values of ![]() are possible?
are possible?
![]()
Problem 24
In ![]() ,
, ![]() , and
, and ![]() is an altitude. Point
is an altitude. Point ![]() is on the extension of
is on the extension of ![]() such that
such that ![]() . The values of
. The values of ![]() ,
, ![]() , and
, and ![]() form a geometric progression, and the values of
form a geometric progression, and the values of ![]()
![]()
![]() form an arithmetic progression. What is the area of
form an arithmetic progression. What is the area of ![]() ?
?
![[asy] size(120); defaultpen(0.7); pair A = (0,0), D = (5*2^.5/3,0), C = (10*2^.5/3,0), B = (5*2^.5/3,5*2^.5), E = (13*2^.5/3,0); draw(A--D--C--E--B--C--D--B--cycle); label("(A)",A,S); label("(B)",B,N); label("(C)",C,S); label("(D)",D,S); label("(E)",E,S); [/asy]](https://latex.artofproblemsolving.com/c/5/a/c5ae6e04f0710cb08f0e10ffc47a0f257844910e.png)
Problem 25
Given that ![]() is a
is a ![]() -digit number whose first digit is
-digit number whose first digit is ![]() , how many elements of the set
, how many elements of the set ![]() have a first digit of
have a first digit of ![]() ?
?
![]()
- Each day Jenny makes half as many free throws as she does at the next practice. Hence on the fourth day she made
 free throws, on the third
free throws, on the third  , on the second
, on the second  , and on the first
, and on the first  .
.
Because there are five days, or four transformations between days (day 1 day 2
day 2  day 3
day 3  day 4
day 4  day 5), she makes
day 5), she makes 
- If
 or
or  , the expression evaluates to
, the expression evaluates to  .
.
If , the expression evaluates to
, the expression evaluates to  .
.
Case remains. In that case, we want to maximize
remains. In that case, we want to maximize  where
where  . Trying out the six possibilities we get that the best one is
. Trying out the six possibilities we get that the best one is  , where
, where  .
.  and
and  .
.- The digit
 can be either the tens digit (
can be either the tens digit ( :
:  possibilities), or the ones digit (
possibilities), or the ones digit ( :
:  possibilities), but we counted the number
possibilities), but we counted the number  twice. This means that out of the
twice. This means that out of the  two-digit numbers,
two-digit numbers,  have at least one digit equal to
have at least one digit equal to  . Therefore the probability is
. Therefore the probability is  .By complementary counting, we count the numbers that do not contain a
.By complementary counting, we count the numbers that do not contain a  , then subtract from the total. There is a
, then subtract from the total. There is a  probability of choosing a number that does NOT contain a
probability of choosing a number that does NOT contain a  . Subtract this from
. Subtract this from  and simplify yields
and simplify yields  .
. -
Solution 1
Isabella had
 Canadian dollars. Setting up an equation we get
Canadian dollars. Setting up an equation we get  , which solves to
, which solves to  , and the sum of digits of
, and the sum of digits of  is
is  .
.Solution 2
Each time Isabella exchanges
 U.S. dollars, she gets
U.S. dollars, she gets  Canadian dollars and
Canadian dollars and  Canadian dollars extra. Isabella received a total of
Canadian dollars extra. Isabella received a total of  Canadian dollars extra, therefore she exchanged
Canadian dollars extra, therefore she exchanged  U.S. dollars
U.S. dollars  times. Thus
times. Thus  , and the sum of the digits is
, and the sum of the digits is  .
. - The directions "southwest" and "southeast" are orthogonal. Thus the described situation is a right triangle with legs
 miles and
miles and  miles long. The hypotenuse length is
miles long. The hypotenuse length is  , and thus the answer is
, and thus the answer is  .Without a calculator one can note that
.Without a calculator one can note that  .
. - The area of the circle is
 ; the area of the square is
; the area of the square is  .Exactly
.Exactly  of the circle lies inside the square. Thus the total area is
of the circle lies inside the square. Thus the total area is  .
.![[asy] Draw(Circle((0,0),10)); Draw((0,0)--(10,0)--(10,10)--(0,10)--(0,0)); label("$10$",(5,0),S); label("$10$",(0,5),W); dot((0,0)); [/asy]](https://latex.artofproblemsolving.com/9/f/c/9fcea2965eceab15b23e988726a4b3446b01b59f.png)
- The sum of the first
 odd numbers is
odd numbers is  . As in our case
. As in our case  , we have
, we have  .
. - The entire situation is in the picture below. The correct answer is
 .
.![[asy] unitsize(1cm); defaultpen(0.8); pair A=(-3,2), B=rotate(-90)*A, C=(3,2); dot(A); dot(B); dot(C); draw( A -- (0,0) -- B -- C, Dotted ); draw( (-3,-3) -- (4,4), dashed ); label("$A=(-3,2)$", A, NW ); label("$B=(2,3)$", B, N ); label("$C=(3,2)$", C, E ); label("$x=y$",(4,4),NE); dot((0,0)); label("$(0,0)$", (0,0), SE); [/asy]](https://oss.linstitute.net/wechatimg/2023/09/5c86b2e7e7f1e8eda5185b38ddd190d011cf750a.png)
- The area of the large circle is
 , the area of the small one is
, the area of the small one is  , hence the shaded area is
, hence the shaded area is  .From the Pythagorean Theorem for the right triangle
.From the Pythagorean Theorem for the right triangle  we have
we have  , hence
, hence  and thus the shaded area is
and thus the shaded area is 
- Let the number of students be
 . Then the sum of their scores is at least
. Then the sum of their scores is at least  . At the same time, we need to achieve the mean
. At the same time, we need to achieve the mean  , which is equivalent to achieving the sum
, which is equivalent to achieving the sum  .Hence we get a necessary condition on
.Hence we get a necessary condition on  : we must have
: we must have  . This can be simplified to
. This can be simplified to  . The smallest integer
. The smallest integer  for which this is true is
for which this is true is  .To finish our solution, we now need to find one way how
.To finish our solution, we now need to find one way how  students could have scored on the test. We have
students could have scored on the test. We have  points to divide among them. The five
points to divide among them. The five  s make
s make  , hence we must divide the remaining
, hence we must divide the remaining  points among the other
points among the other  students. This can be done e.g. by giving
students. This can be done e.g. by giving  points to each of them.Hence the smallest possible number of students is
points to each of them.Hence the smallest possible number of students is  .
. -
Solution 1
We already know that
 ,
,  ,
,  , and
, and  . Let's compute the next few terms to get the idea how the sequence behaves. We get
. Let's compute the next few terms to get the idea how the sequence behaves. We get  ,
,  ,
,  , and so on.
, and so on.We can now discover the following pattern:
 and
and  . This is easily proved by induction. It follows that
. This is easily proved by induction. It follows that  .
.Solution 2
Note that the recurrence
 can be rewritten as
can be rewritten as  .
.Hence we get that
 and also
and also 
From the values given in the problem statement we see that
 .
.From
 we get that
we get that  .
.From
 we get that
we get that  .
.Following this pattern, we get
 .
. - By the definition of an inverse function,
 . By comparing coefficients, we have
. By comparing coefficients, we have  and
and  . Simplifying,
. Simplifying,![[a^3 + 1 = 0]](https://latex.artofproblemsolving.com/c/b/e/cbefb3a23c45a0832c72319868fb12890d07d033.png) and
and  . Thus
. Thus  .
. -
Solution 1
The triangle
 is clearly a right triangle, its area is
is clearly a right triangle, its area is  . If we knew the areas of triangles
. If we knew the areas of triangles  and
and  , we could subtract them to get the area of the pentagon.
, we could subtract them to get the area of the pentagon.Draw the height
 from
from  onto
onto  . As
. As  and the area is
and the area is  , we get
, we get  . The situation is shown in the picture below:
. The situation is shown in the picture below:![[asy] unitsize(0.5cm); defaultpen(0.8); pair C=(0,0), A=(0,5), B=(12,0), M=(0,4), N=(4,0); pair J=intersectionpoint(A--B, M--(M+rotate(90)*(B-A)) ); pair K=intersectionpoint(A--B, N--(N+rotate(90)*(B-A)) ); pair L=intersectionpoint(A--B, C--(C+rotate(90)*(B-A)) ); draw( A--B--C--cycle ); draw( M--J ); draw( N--K ); draw( C--L, dashed ); label("$A$",A,NW); label("$B$",B,SE); label("$C$",C,SW); label("$M$",M,SW); label("$N$",N,S); label("$J$",J,NE); label("$K$",K,NE); label("$L$",L,NE); [/asy]](https://latex.artofproblemsolving.com/5/c/5/5c537489018db33f3318c7e0d03fee0274596fd2.png)
Now note that the triangles
 ,
,  ,
,  ,
,  and
and  all have the same angles and therefore they are similar. We already know some of their sides, and we will use this information to compute their areas. Note that if two polygons are similar with ratio
all have the same angles and therefore they are similar. We already know some of their sides, and we will use this information to compute their areas. Note that if two polygons are similar with ratio  , their areas have ratio
, their areas have ratio  . We will use this fact repeatedly. Below we will use
. We will use this fact repeatedly. Below we will use ![$[XYZ]$](https://latex.artofproblemsolving.com/c/7/6/c7608a3e97e44fc5bcf0cd62a1f7dfc0a0a2e7d4.png) to denote the area of the triangle
to denote the area of the triangle  .
.We have
 , hence
, hence ![$[ACL] = frac{ 25[ABC] }{169} = frac{750}{169}$](https://latex.artofproblemsolving.com/6/b/0/6b05eca7817305831f89c8c32b095fc744d0f3ce.png) .
.Also,
 , hence
, hence ![$[CBL] = frac{ 144[ABC] }{169} = frac{4320}{169}$](https://latex.artofproblemsolving.com/b/a/d/bad2c53ab712a9f04b729fc778a0a5c8ab76af1f.png) .
.Now for the smaller triangles:
We know that
 , hence
, hence ![$[AMJ] = frac{[ACL]}{25} = frac{30}{169}$](https://latex.artofproblemsolving.com/a/f/9/af91c0d6d38fd841753aeb4ad6a1d2c935a07d86.png) .
.Similarly,
 , hence
, hence ![$[NBK] = frac{4[CBL]}9 = frac{1920}{169}$](https://latex.artofproblemsolving.com/0/5/b/05bc3ab44774189bb5da99a6a8bf30767fcb0b84.png) .
.Finally, the area of the pentagon is
 .
.Solution 2
Split the pentagon along a different diagonal as follows:
![[asy] unitsize(0.5cm); defaultpen(0.8); pair C=(0,0), A=(0,5), B=(12,0), M=(0,4), N=(4,0); pair J=intersectionpoint(A--B, M--(M+rotate(90)*(B-A)) ); pair K=intersectionpoint(A--B, N--(N+rotate(90)*(B-A)) ); pair L=intersectionpoint(A--B, C--(C+rotate(90)*(B-A)) ); draw( A--B--C--cycle ); draw( M--J ); draw( N--K ); draw( M--N, dashed ); label("$A$",A,NW); label("$B$",B,SE); label("$C$",C,SW); label("$M$",M,SW); label("$N$",N,S); label("$J$",J,NE); label("$K$",K,NE); label("$L$",L,NE); [/asy]](https://latex.artofproblemsolving.com/b/0/8/b08283bf71f207d83324f9593120f3bfd6470fe1.png)
The area of the pentagon is then the sum of the areas of the resulting right triangle and trapezoid. As before, triangles ,
,  , and
, and  are all similar.
are all similar.Since
 ,
,  and
and  . Since
. Since  ,
,  and
and  .
.The trapezoid's height is therefore
 , and its area is
, and its area is  .
.Triangle
 has area
has area  , and the total area is
, and the total area is  .
.Solution 3
Because triangle ABC, triangle NBK, and triangle AMJ are similar right triangles whose hypotenuses are in the ratio 13 : 8 : 1, their areas are in the ratio 169 : 64 : 1. The area of triangle ABC is 1/2 (12)(5) = 30, so the areas of triangle NBK and triangle AMJ are (64/169) (30) and (1/169)(30), respectively. Thus the area of pentagon CMJKN is (1 − 64/169 - 1/169)(30)=

-
Solution 1
If Jack's current age is
 , then Bill's current age is
, then Bill's current age is  .
.In five years, Jack's age will be
 and Bill's age will be
and Bill's age will be  .
.We are given that
 . Thus
. Thus  .
.For
 we get
we get  . For
. For  and
and  the value
the value  is not an integer, and for
is not an integer, and for  it is more than
it is more than  . Thus the only solution is
. Thus the only solution is  , and the difference in ages is
, and the difference in ages is  .
.Solution 2
Age difference does not change in time. Thus in five years Bill's age will be equal to their age difference.
The age difference is
 , hence it is a multiple of
, hence it is a multiple of  . Thus Bill's current age modulo
. Thus Bill's current age modulo  must be
must be  .
.Thus Bill's age is in the set
 .
.As Jack is older, we only need to consider the cases where the tens digit of Bill's age is smaller than the ones digit. This leaves us with the options
 .
.Checking each of them, we see that only
 works, and gives the solution
works, and gives the solution  .
. - Let
 , so
, so  . By definition,
. By definition,  , which implies that all solutions to
, which implies that all solutions to  lie on the line
lie on the line  on the complex plane. The graph of
on the complex plane. The graph of  is a circle centered at the origin, and there are
is a circle centered at the origin, and there are  intersections.
intersections. - Let the three roots be
 .
.![[log_2 x_1 + log_2 x_2 + log_2 x_3 = log_2 x_1x_2x_3= 5 Longrightarrow x_1x_2x_3 = 32]](https://latex.artofproblemsolving.com/9/e/a/9eaba1d48b6d1b7d491b25a3503629ff84a91bee.png) By Vieta’s formulas,
By Vieta’s formulas,![[8(x-x_1)(x-x_2)(x-x_3) = 8x^3 + 4ax^2 + 2bx + a]](https://latex.artofproblemsolving.com/0/6/b/06b76979c050b8824bb4a74150704bf5dd0867a8.png) gives us that
gives us that  .
. - Let the coordinates of
 be
be  . As
. As  lies on the parabola, we have
lies on the parabola, we have  . As the origin is the midpoint of
. As the origin is the midpoint of  , the coordinates of
, the coordinates of  are
are  . We need to choose
. We need to choose  so that
so that  will lie on the parabola as well. In other words, we need
will lie on the parabola as well. In other words, we need  .Substituting for
.Substituting for  , we get:
, we get:  .This simplifies to
.This simplifies to  , which solves to
, which solves to  . Both roots lead to the same pair of points:
. Both roots lead to the same pair of points:  and
and  . Their distance is
. Their distance is  .
.
Alternate Solution
Let the coordinates of
 and
and  be
be  and
and  , respectively. Since the median of the points lies on the origin,
, respectively. Since the median of the points lies on the origin,  and expanding
and expanding  , we find:
, we find:![[4x_A^2 + 7x_A - 1 + 4x_B^2 + 7x_B - 1 = 0]](https://latex.artofproblemsolving.com/3/3/2/332d505f905b0df56bda847ab6cb771e9a8ed25f.png)
![[4(x_A^2 + x_B^2) + 7(x_A + x_B) = 2]](https://latex.artofproblemsolving.com/b/0/d/b0d8ec99843741914f691865bf38b51cdde05739.png)
![[x_A^2 + x_B^2 = frac{1}{2}.]](https://latex.artofproblemsolving.com/d/5/d/d5da12f63c06b93e5c90bbcb57627c12a34280ac.png)
It also follows that
 . Expanding this, we find:
. Expanding this, we find:![[x_A^2 + 2x_A x_B + x_B^2 = 0]](https://latex.artofproblemsolving.com/5/8/8/588f3eb919a7f639c0c37137ee37b6a2892b7b00.png)
![[frac{1}{2} + 2x_A x_B = 0]](https://latex.artofproblemsolving.com/1/2/e/12e864113eedb4a4d33977aa8cb2cfefa220e57a.png)
![[x_A x_B = -frac{1}{4}.]](https://latex.artofproblemsolving.com/b/3/7/b374b51e27ff7539c33dcac9f9c0620ccb0573e5.png)
To find the distance between the points,
 must be found. Expanding
must be found. Expanding  :
:![[y_A - y_B = 4x_A^2 + 7x_A - 1 - 4x_B^2 - 7x_B + 1]](https://latex.artofproblemsolving.com/d/a/d/dadbe8da4462f5b9d9a4b745d6a625682cfe1df0.png)
![[= 4(x_A^2 - x_B^2) + 7(x_A - x_B)]](https://latex.artofproblemsolving.com/d/b/c/dbcc4502200a4616a57fd956c4037550c25bbe0a.png)
![[= 4(x_A + x_B)(x_A - x_B) + 7(x_A - x_B)]](https://latex.artofproblemsolving.com/c/6/e/c6e6eb17e122fef3e50b985d3f341fd87e7d489f.png)
![[= 7(x_A - x_B)]](https://latex.artofproblemsolving.com/3/9/0/390303e0446e8287104471c28a97b3f7d47b5544.png) we find the distance to be
we find the distance to be  . Expanding this yields
. Expanding this yields  .
. -
Solution #1
Consider a trapezoid (label it
 as follows) cross-section of the truncate cone along a diameter of the bases:
as follows) cross-section of the truncate cone along a diameter of the bases:![[asy] import olympiad; size(220); defaultpen(0.7); pair A = (0,0), B = (36,0), C = (20,12), D = (16,12), E=(A+B)/2, F=(20+1.6,12-1.2), G = (C+D)/2; draw(A--B--C--D--cycle); draw(circumcircle(E,F,G)); dot(E); dot(F); dot(G); label("(A)",A,S); label("(B)",B,S); label("(C)",C,NE); label("(D)",D,NW); label("(E)",E,S); label("(F)",F,NE); label("(G)",G,N); [/asy]](https://latex.artofproblemsolving.com/7/9/b/79b290d343dc7c6049f7d45f5f1abc749c2af3d2.png)
 and
and  are points of tangency. By the Two Tangent Theorem,
are points of tangency. By the Two Tangent Theorem,  and
and  , so
, so  . We draw
. We draw  such that it is the foot of the altitude
such that it is the foot of the altitude  to
to  :
:
![[asy] import olympiad; size(220); defaultpen(0.7); pair A = (0,0), B = (36,0), C = (20,12), D = (16,12), E=(A+B)/2, F=(20+1.6,12-1.2), G = (C+D)/2, H=(16,0); pair P=(D+G)/2, Q=(D+H)/2, R=(B+E)/2, T=(A+H)/2, O=(E+G)/2; draw(A--B--C--D--cycle); draw(G--E--H--D); draw(circumcircle(E,F,G)); dot(E);dot(F);dot(G);dot(H);dot(O); label("(A)",A,S); label("(B)",B,S); label("(C)",C,NE); label("(D)",D,NW); label("(E)",E,S); label("(F)",F,NE); label("(G)",G,N); label("(H)",H,S); label("(O)",O,NE); label("(2)",P,N); label("(12)",Q,W); label("(18)",R,S); label("(16)",T,S); label("(20)",(A+D)/2,NW); label("(r)",(O+E)/2,SE); [/asy]](https://latex.artofproblemsolving.com/d/f/5/df55cbef93e6c3485f8c0d77176293f0fe2bcb3a.png)
![[r = frac{DH}2 = frac{sqrt{20^2 - 16^2}}2 = boxed{6} Rightarrow mathrm{(A)}.]](https://latex.artofproblemsolving.com/d/f/3/df3b7879ecc2ac8d3fff4c2b6c95853efdf10988.png)
Solution #2
Create a trapezoid with inscribed circle
 exactly like in Solution #1, and extend lines
exactly like in Solution #1, and extend lines  and
and  from the solution above and label the point at where they meet
from the solution above and label the point at where they meet  . Because
. Because  =
=  ,
,  =
=  . Let
. Let  and
and  .
.Because these are radii,
 .
.  so
so  . Plugging in, we get
. Plugging in, we get  so
so  .Triangles
.Triangles  and
and  are similar so
are similar so  which gives us
which gives us  . Solving for x, we get
. Solving for x, we get![[x = 1.5]](https://latex.artofproblemsolving.com/b/8/f/b8fdb45b0430f931c05a066416c1d0f44c38f43c.png) and
and![[4x = boxed{6} Rightarrow mathrm{(A)}.]](https://latex.artofproblemsolving.com/b/3/e/b3e9fa6e2bdc737c4e300852022c034c21b65881.png)
- There are
 possible colorings of the cube. Consider the color that appears with greater frequency. The property obviously holds true if
possible colorings of the cube. Consider the color that appears with greater frequency. The property obviously holds true if  or
or  of the faces are colored the same, which for each color can happen in
of the faces are colored the same, which for each color can happen in  ways. If
ways. If  of the faces are colored the same, there are
of the faces are colored the same, there are  possible cubes (corresponding to the
possible cubes (corresponding to the  possible ways to pick pairs of opposite faces for the other color). If
possible ways to pick pairs of opposite faces for the other color). If  of the faces are colored the same, the property obviously cannot be satisfied. Thus, there are a total of
of the faces are colored the same, the property obviously cannot be satisfied. Thus, there are a total of  ways for this to occur, and the desired probability is
ways for this to occur, and the desired probability is  .
. 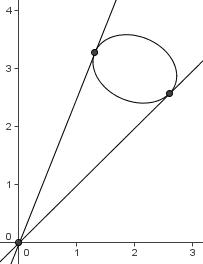
 represents the slope of a line passing through the origin. It follows that since a line
represents the slope of a line passing through the origin. It follows that since a line  intersects the ellipse at either
intersects the ellipse at either  or
or  points, the minimum and maximum are given when the line
points, the minimum and maximum are given when the line  is a tangent, with only one point of intersection. Substituting,
is a tangent, with only one point of intersection. Substituting,![[2x^2 + x(mx) + 3(mx)^2 - 11x - 20(mx) + 40 = 0]](https://latex.artofproblemsolving.com/7/8/1/7810f02a1d842903268ef4e400f6355a7262fa0b.png) Rearranging by the degree of
Rearranging by the degree of  ,
,![[(3m^2 + m + 2)x^2 - (20m + 11)x + 40 = 0]](https://latex.artofproblemsolving.com/3/d/b/3db7670b17d2532db53dee1c1c5e8c1cc7a786c5.png) Since the line
Since the line  , we want the discriminant,
, we want the discriminant,![[(20m+11)^2 - 4cdot 40 cdot (3m^2 + m + 2) = -80m^2 + 280m - 199]](https://latex.artofproblemsolving.com/1/0/b/10b41331a8f9d71b05e9dae9769abf4b648dce70.png) to be equal to
to be equal to  . We want
. We want  , which is the sum of the roots of the above quadratic. By Vieta’s formulas, that is
, which is the sum of the roots of the above quadratic. By Vieta’s formulas, that is  .
.-
Solution A
If the power of a prime
 other than
other than  divides
divides  , then from
, then from  it follows that
it follows that  , but then considering the product of the diagonals,
, but then considering the product of the diagonals,  but
but  , contradiction. So the only prime factors of
, contradiction. So the only prime factors of  are
are  and
and  .
.It suffices now to consider the two magic squares comprised of the powers of
 and
and  of the corresponding terms. These satisfy the normal requirement that the sums of rows, columns, and diagonals are the same, owing to our rules of exponents; additionally, all terms are non-negative.
of the corresponding terms. These satisfy the normal requirement that the sums of rows, columns, and diagonals are the same, owing to our rules of exponents; additionally, all terms are non-negative.The powers of
 :
:
 , so
, so  . Indeed, we have the magic squares
. Indeed, we have the magic squares

 :
:

 . However, if we let
. However, if we let  , then
, then  , which obviously gives us a contradiction, and similarly for
, which obviously gives us a contradiction, and similarly for  . For
. For  , we get
, we get

 can be
can be  , and their sum is
, and their sum is  .
.
Solution B
All the unknown entries can be expressed in terms of
 . Since
. Since  , it follows that
, it follows that  , and
, and  . Comparing rows
. Comparing rows  and
and  then gives
then gives  , from which
, from which  . Comparing columns
. Comparing columns  and
and  gives
gives  , from which
, from which  . Finally,
. Finally,  , and
, and  . All the entries are positive integers if and only if
. All the entries are positive integers if and only if  or
or  . The corresponding values for
. The corresponding values for  are
are  and
and  , and their sum is
, and their sum is  .
.Solution C
We know because this is a multiplicative magic square that each of the following are equal to each other:

From this we know that
 , thus
, thus  . Thus
. Thus  and
and  . Thus
. Thus  From this we know that
From this we know that  . Thus
. Thus  . Now we know from the very beginning that
. Now we know from the very beginning that  or
or  or
or  or
or  . Rearranging the equation
. Rearranging the equation  we have
we have  or
or  due to
due to  and
and  both being positive. Now that
both being positive. Now that  we find all pairs of positive integers that multiply to
we find all pairs of positive integers that multiply to  . There is
. There is  . Now we know that
. Now we know that  and b has to be a positive integer. Thus
and b has to be a positive integer. Thus  can only be
can only be  ,
,  , or
, or  . Thus
. Thus  can only be
can only be  ,
, ,or
,or  . Thus sum of
. Thus sum of  =
=  . The answer is
. The answer is  .
. - Letting the roots be
 ,
,  , and
, and  , where
, where  , we see that by Vieta's Formula's,
, we see that by Vieta's Formula's,  , and so
, and so  . Therefore,
. Therefore,  is a factor of
is a factor of  . Letting
. Letting  gives that
gives that  because
because  . Letting
. Letting  and noting that
and noting that  for some
for some  , we see that
, we see that  is the sum of the roots of
is the sum of the roots of  ,
,  and
and  , and so
, and so  . Now, we have that
. Now, we have that  has roots
has roots  and
and  , and we wish to find the number of possible values of
, and we wish to find the number of possible values of  . By the quadratic formula, we see that
. By the quadratic formula, we see that![[frac{1002 pm sqrt{1002^2 - 4a}}{2} = 501 pm sqrt{501^2 - a}]](https://latex.artofproblemsolving.com/9/1/0/910c7c622afd81998d260e3e8d4fe2b7fa178550.png) are the two values of noninteger positive real numbers
are the two values of noninteger positive real numbers  and
and  , neither of which is equal to
, neither of which is equal to  . This information gives us that
. This information gives us that  , and so since
, and so since  is evidently not a square, we have
is evidently not a square, we have  possible values of
possible values of  .
. - Let
 . Then the first condition tells us that
. Then the first condition tells us that![[tan^2 DBE = tan(DBE - alpha)tan(DBE + alpha) = frac {tan^2 DBE - tan^2 alpha}{1 - tan ^2 DBE tan^2 alpha},]](https://latex.artofproblemsolving.com/f/4/3/f43efdcb033513acd38738c89f90c9c23fb85ba3.png) and multiplying out gives us
and multiplying out gives us  . Since
. Since  , we have
, we have  .The second condition tells us that
.The second condition tells us that  . Expanding, we have
. Expanding, we have ![$1 + cot alpha = 2left[frac {cot alpha + 1}{cot alpha - 1}right] Longrightarrow (cot alpha - 3)(cot alpha + 1) = 0$](https://latex.artofproblemsolving.com/f/3/b/f3b767460b7483cbf73ecb3c2300510a7b60ea87.png) . Evidently
. Evidently  , so we get
, so we get  .Now
.Now  and
and  . Thus,
. Thus, ![$[ABC] = frac {1}{2} cdot 5sqrt {2} cdot frac {10sqrt {2}}{3} = frac {50}{3} mathrm{(B)}$](https://latex.artofproblemsolving.com/b/0/7/b077d0451ba673b17b24b6f4fe9a43f1bf374f32.png) .
. -
Solution 1
Given
 digits, there must be exactly one power of
digits, there must be exactly one power of  with
with  digits such that the first digit is
digits such that the first digit is  . Thus
. Thus  contains
contains  elements with a first digit of
elements with a first digit of  . For each number in the form of
. For each number in the form of  such that its first digit is
such that its first digit is  , then
, then  must either have a first digit of
must either have a first digit of  or
or  , and
, and  must have a first digit of
must have a first digit of  . Thus there are also
. Thus there are also  numbers with first digit
numbers with first digit  and
and  numbers with first digit
numbers with first digit  . By using complementary counting, there are
. By using complementary counting, there are  elements of
elements of  with a first digit of
with a first digit of  . Now,
. Now,  has a first digit of
has a first digit of  if and only if the first digit of
if and only if the first digit of  is
is  , so there are
, so there are  elements of
elements of  with a first digit of
with a first digit of  .
.Solution 2
We can make the following chart for the possible loops of leading digits:
![[1 rightarrow 2 rightarrow 4 rightarrow 8 rightarrow 1]](https://latex.artofproblemsolving.com/6/c/1/6c11f15077c70110ca7a652f919bd2bd983267e5.png)
![[1 rightarrow 2 rightarrow 4 rightarrow 9 rightarrow 1]](https://latex.artofproblemsolving.com/b/b/6/bb67144da4deeb76ccc5bb437383d5549f8971e1.png)
![[1 rightarrow 2 rightarrow 5 rightarrow 1]](https://latex.artofproblemsolving.com/7/0/1/70158a66276ee855e8cca206278f15bc1c99b7fe.png)
![[1 rightarrow 3 rightarrow 6 rightarrow 1]](https://latex.artofproblemsolving.com/8/2/8/828285adca86f8d1e7c385e61533d6cf738649a5.png)
![[1 rightarrow 3 rightarrow 7 rightarrow 1]](https://latex.artofproblemsolving.com/6/9/d/69dcf68d6120c8b0e64c1b971f1f6bdb9727fafa.png)
Thus each loop from
 can either have
can either have  or
or  numbers. Let there be
numbers. Let there be  of the sequences of
of the sequences of  numbers, and let there be
numbers, and let there be  of the sequences of
of the sequences of  numbers. We note that a
numbers. We note that a  appears only in the loops of
appears only in the loops of  , and also we are given that
, and also we are given that  has
has  digits.
digits.![[3x+4y=2004]](https://latex.artofproblemsolving.com/5/1/1/51179a87eae90f72464a3a8f1d212cd020f783ea.png)
![[x+y=603]](https://latex.artofproblemsolving.com/0/8/9/08976c6977d3e5a35c2d339360248bc00d9679b4.png) Solving gives
Solving gives  and
and  , thus the answer is
, thus the answer is  .
.
学术活动报名扫码了解!免费领取历年真题!

最新发布
© 2025. All Rights Reserved. 沪ICP备2023009024号-1









