Home » 国际竞赛 » 数学国际竞赛 » AMC美国数学竞赛 » Details

2003 AMC12A 真题及答案详细解析

Category: AMC美国数学竞赛, 国际竞赛 Date: 2019年12月5日上午10:54

2003 AMC 12A 真题

答案详细解析请参考文末

Problem 1

What is the difference between the sum of the first $2003$ even counting numbers and the sum of the first $2003$ odd counting numbers? $mathrm{(A) } 0qquad mathrm{(B) } 1qquad mathrm{(C) } 2qquad mathrm{(D) } 2003qquad mathrm{(E) } 4006$

Problem 2

Members of the Rockham Soccer League buy socks and T-shirts. Socks cost $4 per pair and each T-shirt costs $5 more than a pair of socks. Each member needs one pair of socks and a shirt for home games and another pair of socks and a shirt for away games. If the total cost is $2366, how many members are in the League? $mathrm{(A) } 77qquad mathrm{(B) } 91qquad mathrm{(C) } 143qquad mathrm{(D) } 182qquad mathrm{(E) } 286$

Problem 3

A solid box is $15$ cm by $10$ cm by $8$ cm. A new solid is formed by removing a cube $3$ cm on a side from each corner of this box. What percent of the original volume is removed? $mathrm{(A) } 4.5qquad mathrm{(B) } 9qquad mathrm{(C) } 12qquad mathrm{(D) } 18qquad mathrm{(E) } 24$

Problem 4

It takes Mary $30$ minutes to walk uphill $1$ km from her home to school, but it takes her only $10$ minutes to walk from school to her home along the same route. What is her average speed, in km/hr, for the round trip? $mathrm{(A) } 3qquad mathrm{(B) } 3.125qquad mathrm{(C) } 3.5qquad mathrm{(D) } 4qquad mathrm{(E) } 4.5$

Problem 5

The sum of the two 5-digit numbers $AMC10$ and $AMC12$ is $123422$ . What is $A+M+C$ ? $mathrm{(A) } 10qquad mathrm{(B) } 11qquad mathrm{(C) } 12qquad mathrm{(D) } 13qquad mathrm{(E) } 14$

Problem 6

Define $x heartsuit y$ to be $|x-y|$ for all real numbers $x$ and $y$ . Which of the following statements is not true? $mathrm{(A) } x heartsuit y = y heartsuit x$ for all $x$ and $y$ $mathrm{(B) } 2(x heartsuit y) = (2x) heartsuit (2y)$ for all $x$ and $y$ $mathrm{(C) } x heartsuit 0 = x$ for all $x$ $mathrm{(D) } x heartsuit x = 0$ for all $x$ $mathrm{(E) } x heartsuit y > 0$ if $x neq y$

Problem 7

How many non-congruent triangles with perimeter $7$ have integer side lengths? $mathrm{(A) } 1qquad mathrm{(B) } 2qquad mathrm{(C) } 3qquad mathrm{(D) } 4qquad mathrm{(E) } 5$

Problem 8

What is the probability that a randomly drawn positive factor of $60$ is less than $7$ ? $mathrm{(A) } frac{1}{10}qquad mathrm{(B) } frac{1}{6}qquad mathrm{(C) } frac{1}{4}qquad mathrm{(D) } frac{1}{3}qquad mathrm{(E) } frac{1}{2}$

Problem 9

A set $S$ of points in the $xy$ -plane is symmetric about the origin, both coordinate axes, and the line $y=x$ . If $(2,3)$ is in $S$ , what is the smallest number of points in $S$ ? $mathrm{(A) } 1qquad mathrm{(B) } 2qquad mathrm{(C) } 4qquad mathrm{(D) } 8qquad mathrm{(E) } 16$

Problem 10

Al, Bert, and Carl are the winners of a school drawing for a pile of Halloween candy, which they are to divide in a ratio of $3:2:1$ , respectively. Due to some confusion they come at different times to claim their prizes, and each assumes he is the first to arrive. If each takes what he believes to be the correct share of candy, what fraction of the candy goes unclaimed? $mathrm{(A) } frac{1}{18}qquad mathrm{(B) } frac{1}{6}qquad mathrm{(C) } frac{2}{9}qquad mathrm{(D) } frac{5}{18}qquad mathrm{(E) } frac{5}{12}$

Problem 11

A square and an equilateral triangle have the same perimeter. Let $A$ be the area of the circle circumscribed about the square and $B$ the area of the circle circumscribed around the triangle. Find $A/B$ . $mathrm{(A) } frac{9}{16}qquad mathrm{(B) } frac{3}{4}qquad mathrm{(C) } frac{27}{32}qquad mathrm{(D) } frac{3sqrt{6}}{8}qquad mathrm{(E) } 1$

Problem 12

Sally has five red cards numbered $1$ through $5$ and four blue cards numbered $3$ through $6$ . She stacks the cards so that the colors alternate and so that the number on each red card divides evenly into the number on each neighboring blue card. What is the sum of the numbers on the middle three cards? $mathrm{(A) } 8qquad mathrm{(B) } 9qquad mathrm{(C) } 10qquad mathrm{(D) } 11qquad mathrm{(E) } 12$

Problem 13

The polygon enclosed by the solid lines in the figure consists of 4 congruent squares joined edge-to-edge. One more congruent square is attached to an edge at one of the nine positions indicated. How many of the nine resulting polygons can be folded to form a cube with one face missing? 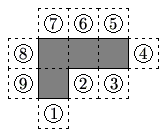$mathrm{(A) } 2qquad mathrm{(B) } 3qquad mathrm{(C) } 4qquad mathrm{(D) } 5qquad mathrm{(E) } 6$

Problem 14

Points $K, L, M,$ and $N$ lie in the plane of the square $ABCD$ such that $AKB$ , $BLC$ , $CMD$ , and $DNA$ are equilateral triangles. If $ABCD$ has an area of 16, find the area of $KLMN$ . $[asy] unitsize(2cm); defaultpen(fontsize(8)+linewidth(0.8)); pair A=(-0.5,0.5), B=(0.5,0.5), C=(0.5,-0.5), D=(-0.5,-0.5); pair K=(0,1.366), L=(1.366,0), M=(0,-1.366), N=(-1.366,0); draw(A--N--K--A--B--K--L--B--C--L--M--C--D--M--N--D--A); label("$A$",A,SE); label("$B$",B,SW); label("$C$",C,NW); label("$D$",D,NE); label("$K$",K,NNW); label("$L$",L,E); label("$M$",M,S); label("$N$",N,W); [/asy]$ $textrm{(A)} 32qquadtextrm{(B)} 16+16sqrt{3}qquadtextrm{(C)} 48qquadtextrm{(D)} 32+16sqrt{3}qquadtextrm{(E)} 64$

Problem 15

A semicircle of diameter $1$ sits at the top of a semicircle of diameter $2$ , as shown. The shaded area inside the smaller semicircle and outside the larger semicircle is called a lune. Determine the area of this lune. $mathrm{(A) } frac{1}{6}pi-frac{sqrt{3}}{4}qquad mathrm{(B) } frac{sqrt{3}}{4}-frac{1}{12}piqquad mathrm{(C) } frac{sqrt{3}}{4}-frac{1}{24}piqquad mathrm{(D) } frac{sqrt{3}}{4}+frac{1}{24}piqquad mathrm{(E) } frac{sqrt{3}}{4}+frac{1}{12}pi$

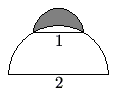
Problem 16

A point P is chosen at random in the interior of equilateral triangle $ABC$ . What is the probability that $triangle ABP$ has a greater area than each of $triangle ACP$ and $triangle BCP$ ? $textbf{(A)} frac{1}{6}qquadtextbf{(B)} frac{1}{4}qquadtextbf{(C)} frac{1}{3}qquadtextbf{(D)} frac{1}{2}qquadtextbf{(E)} frac{2}{3}$

Problem 17

Square $ABCD$ has sides of length $4$ , and $M$ is the midpoint of $overline{CD}$ . A circle with radius $2$ and center $M$ intersects a circle with radius $4$ and center $A$ at points $P$ and $D$ . What is the distance from $P$ to $overline{AD}$ ? $[asy] pair A,B,C,D,M,P; D=(0,0); C=(10,0); B=(10,10); A=(0,10); M=(5,0); P=(8,4); dot(M); dot(P); draw(A--B--C--D--cycle,linewidth(0.7)); draw((5,5)..D--C..cycle,linewidth(0.7)); draw((7.07,2.93)..B--A--D..cycle,linewidth(0.7)); label("$A$",A,NW); label("$B$",B,NE); label("$C$",C,SE); label("$D$",D,SW); label("$M$",M,S); label("$P$",P,N); [/asy]$ $textbf{(A)} 3 qquad textbf{(B)} frac {16}{5} qquad textbf{(C)} frac {13}{4} qquad textbf{(D)} 2sqrt {3} qquad textbf{(E)} frac {7}{2}$

Problem 18

Let $n$ be a $5$ -digit number, and let $q$ and $r$ be the quotient and the remainder, respectively, when $n$ is divided by $100$ . For how many values of $n$ is $q+r$ divisible by $11$ ? $mathrm{(A) } 8180qquad mathrm{(B) } 8181qquad mathrm{(C) } 8182qquad mathrm{(D) } 9000qquad mathrm{(E) } 9090$

Problem 19

A parabola with equation $y=ax^2+bx+c$ is reflected about the $x$ -axis. The parabola and its reflection are translated horizontally five units in opposite directions to become the graphs of $y=f(x)$ and $y=g(x)$ , respectively. Which of the following describes the graph of $y=(f+g)(x)$ ? $textbf{(A)} text{a parabola tangent to the }xtext{-axis}$ $textbf{(B)} text{a parabola not tangent to the }xtext{-axis}qquadtextbf{(C)} text{a horizontal line}$ $textbf{(D)} text{a non-horizontal line}qquadtextbf{(E)} text{the graph of a cubic function}$

Problem 20

How many $15$ -letter arrangements of $5$ A's, $5$ B's, and $5$ C's have no A's in the first $5$ letters, no B's in the next $5$ letters, and no C's in the last $5$ letters? $textrm{(A)} sum_{k=0}^{5}binom{5}{k}^{3}qquadtextrm{(B)} 3^{5}cdot 2^{5}qquadtextrm{(C)} 2^{15}qquadtextrm{(D)} frac{15!}{(5!)^{3}}qquadtextrm{(E)} 3^{15}$

Problem 21

The graph of the polynomial $P(x) = x^5 + ax^4 + bx^3 + cx^2 + dx + e$ has five distinct $x$ -intercepts, one of which is at $(0,0)$ . Which of the following coefficients cannot be zero? $textbf{(A)} a qquad textbf{(B)} b qquad textbf{(C)} c qquad textbf{(D)} d qquad textbf{(E)} e$

Problem 22

Objects $A$ and $B$ move simultaneously in the coordinate plane via a sequence of steps, each of length one. Object $A$ starts at $(0,0)$ and each of its steps is either right or up, both equally likely. Object $B$ starts at $(5,7)$ and each of its steps is either to the left or down, both equally likely. Which of the following is closest to the probability that the objects meet? $mathrm{(A)} 0.10 qquad mathrm{(B)} 0.15 qquad mathrm{(C)} 0.20 qquad mathrm{(D)} 0.25 qquad mathrm{(E)} 0.30 qquad$

Problem 23

How many perfect squares are divisors of the product $1! cdot 2! cdot 3! cdot hdots cdot 9!$ ? $textbf{(A)} 504qquadtextbf{(B)} 672qquadtextbf{(C)} 864qquadtextbf{(D)} 936qquadtextbf{(E)} 1008$

Problem 24

If $ageq b > 1,$ what is the largest possible value of $log_{a}(a/b) + log_{b}(b/a)?$ $mathrm{(A)} -2 qquad mathrm{(B)} 0 qquad mathrm{(C)} 2 qquad mathrm{(D)} 3 qquad mathrm{(E)} 4$

Problem 25

Let $f(x)= sqrt{ax^2+bx}$ . For how many real values of $a$ is there at least one positive value of $b$ for which the domain of $f$ and the range of $f$ are the same set? $mathrm{(A) 0 } qquad mathrm{(B) 1 } qquad mathrm{(C) 2 } qquad mathrm{(D) 3 } qquad mathrm{(E) mathrm{infinitely many} }$ <hr />

2003 AMC12 A 真题答案详细解析

Solution 1 The first $2003$ even counting numbers are $2,4,6,...,4006$ .
The first $2003$ odd counting numbers are $1,3,5,...,4005$ .
Thus, the problem is asking for the value of $(2+4+6+...+4006)-(1+3+5+...+4005)$ . $(2+4+6+...+4006)-(1+3+5+...+4005) = (2-1)+(4-3)+(6-5)+...+(4006-4005)$ $= 1+1+1+...+1 = boxed{mathrm{(D)} 2003}$ Solution 2 Using the sum of an arithmetic progression formula, we can write this as $frac{2003}{2}(2 + 4006) - frac{2003}{2}(1 + 4005) = frac{2003}{2} cdot 2 = boxed{mathrm{(D)} 2003}$ . Solution 3 The formula for the sum of the first $n$ even numbers, is $S_E=n^{2}+n$ , (E standing for even).
Sum of first $n$ odd numbers, is $S_O=n^{2}$ , (O standing for odd).
Knowing this, plug $2003$ for $n$ , $S_E-S_O= (2003^{2}+2003)-(2003^{2})=2003 Rightarrow$ $boxed{mathrm{(D)} 2003}$ .
Since T-shirts cost $5$ dollars more than a pair of socks, T-shirts cost $5+4=9$ dollars.Since each member needs $2$ pairs of socks and $2$ T-shirts, the total cost for $1$ member is $2(4+9)=26$ dollars.Since $2366$ dollars was the cost for the club, and $26$ was the cost per member, the number of members in the League is $2366div 26=boxed{mathrm{(B)} 91}$ .
The volume of the original box is $15cdot10cdot8=1200$ The volume of each cube that is removed is $3cdot3cdot3=27$ Since there are $8$ corners on the box, $8$ cubes are removed.So the total volume removed is $8cdot27=216$ .Therefore, the desired percentage is $frac{216}{1200}cdot100 = boxed{mathrm{(D)} 18%}$
Solution 1

Since she walked $1$ km to school and $1$ km back home, her total distance is $1+1=2$ km.
Since she spent $30$ minutes walking to school and $10$ minutes walking back home, her total time is $30+10=40$ minutes = $frac{40}{60}=frac{2}{3}$ hours.
Therefore her average speed in km/hr is $frac{2}{frac{2}{3}}=boxed{mathrm{(A)} 3}$ .

Solution 2

The average speed of two speeds that travel the same distance is the harmonic mean of the speeds, or $dfrac{2}{dfrac{1}{x}+dfrac{1}{y}}=dfrac{2xy}{x+y}$ (for speeds $x$ and $y$ ). Mary's speed going to school is $2,text{km/hr}$ , and her speed coming back is $6,text{km/hr}$ . Plugging the numbers in, we get that the average speed is $dfrac{2times 6times 2}{6+2}=dfrac{24}{8}=boxed{mathrm{(A)} 3}$ .
$AMC10+AMC12=123422$ $AMC00+AMC00=123400$ $AMC+AMC=1234$ $2cdot AMC=1234$ $AMC=frac{1234}{2}=617$ Since $A$ , $M$ , and $C$ are digits, $A=6$ , $M=1$ , $C=7$ .Therefore, $A+M+C = 6+1+7 = boxed{mathrm{(E)} 14}$ .
Examining statement C: $x heartsuit 0 = |x-0| = |x|$ $|x| neq x$ when $x<0$ , but statement C says that it does for all $x$ .Therefore the statement that is not true is $boxed{mathrm{(C)} xheartsuit 0=x text{for all} x}$
By the triangle inequality, no side may have a length greater than the semiperimeter, which is $frac{1}{2}cdot7=3.5$ .Since all sides must be integers, the largest possible length of a side is $3$ . Therefore, all such triangles must have all sides of length $1$ , $2$ , or $3$ . Since $2+2+2=6<7$ , at least one side must have a length of $3$ . Thus, the remaining two sides have a combined length of $7-3=4$ . So, the remaining sides must be either $3$ and $1$ or $2$ and $2$ . Therefore, the number of triangles is $boxed{mathrm{(B)} 2}$ .
Solution 1

For a positive number $n$ which is not a perfect square, exactly half of the positive factors will be less than $sqrt{n}$ .
Since $60$ is not a perfect square, half of the positive factors of $60$ will be less than $sqrt{60}approx 7.746$ .
Clearly, there are no positive factors of $60$ between $7$ and $sqrt{60}$ .
Therefore half of the positive factors will be less than $7$ .
So the answer is $boxed{mathrm{(E)} frac{1}{2}}$ .

Solution 2

Testing all numbers less than $7$ , numbers $1, 2, 3, 4, 5$ , and $6$ divide $60$ . The prime factorization of $60$ is $2^2cdot 3 cdot 5$ . Using the formula for the number of divisors, the total number of divisors of $60$ is $(3)(2)(2) = 12$ . Therefore, our desired probability is $frac{6}{12} = boxed{mathrm{(E)} frac{1}{2}}$
If $(2,3)$ is in $S$ , then $(3,2)$ is also, and quickly we see that every point of the form $(pm 2, pm 3)$ or $(pm 3, pm 2)$ must be in $S$ . Now note that these $8$ points satisfy all of the symmetry conditions. Thus the answer is $boxed{mathrm{(D)} 8}$ .
Because the ratios are $3:2:1$ , Al, Bert, and Carl believe that they need to take $1/2$ , $1/3$ , and $1/6$ of the pile when they each arrive, respectively. After each person comes, $1/2$ , $2/3$ , and $5/6$ of the pile's size (just before each came) remains. The pile starts at $1$ , and at the end $frac{1}{2}cdot frac{2}{3}cdot frac{5}{6}cdot 1 = frac{5}{18}$ of the original pile goes unclaimed. (Note that because of the properties of multiplication, it does not matter what order the three come in.) Hence the answer is $boxed{mathrm{(D)} dfrac{5}{18}}$ .
Suppose that the common perimeter is $P$ . Then, the side lengths of the square and triangle, respectively, are $frac{P}{4}$ and $frac{P}{3}$ The circle circumscribed about the square has a diameter equal to the diagonal of the square, which is $frac{Psqrt{2}}{4}$ Therefore, the radius is $frac{Psqrt{2}}{8}$ and the area of the circle is $pi cdot left(frac{Psqrt{2}}{8}right)^2 = pi cdot frac{2P^2}{64}=frac{P^2 pi}{32}=A$ Now consider the circle circumscribed around the equilateral triangle. Due to symmetry, the circle must share a center with the equilateral triangle. The radius of the circle is simply the distance from the center of the triangle to a vertex. This distance is $frac{2}{3}$ of an altitude. By $30-60-90$ right triangle properties, the altitude is $frac{sqrt{3}}{2} cdot s$ where s is the side. So, the radius is $frac{2}{3} cdot frac{sqrt{3}}{2} cdot frac{P}{3} = frac{Psqrt{3}}{9}$ The area of the circle is $pi cdot left(frac{Psqrt{3}}{9}right)^2=pi cdot frac{3P^2}{81}=frac{P^2pi}{27}=B$ So, $frac{A}{B}=frac{frac{P^2 pi}{32}}{frac{P^2 pi}{27}}=frac{P^2 pi}{32} cdot frac{27}{P^2pi}=boxed{frac{27}{32} implies mathrm{(C) } frac{27}{32}}$
Let $R_i$ and $B_j$ designate the red card numbered $i$ and the blue card numbered $j$ , respectively. $B_5$ is the only blue card that $R_5$ evenly divides, so $R_5$ must be at one end of the stack and $B_5$ must be the card next to it. $R_1$ is the only other red card that evenly divides $B_5$ , so $R_1$ must be the other card next to $B_5$ . $B_4$ is the only blue card that $R_4$ evenly divides, so $R_4$ must be at one end of the stack and $B_4$ must be the card next to it. $R_2$ is the only other red card that evenly divides $B_4$ , so $R_2$ must be the other card next to $B_4$ . $R_2$ doesn't evenly divide $B_3$ , so $B_3$ must be next to $R_1$ , $B_6$ must be next to $R_2$ , and $R_3$ must be in the middle.This yields the following arrangement from top to bottom: ${R_5,B_5,R_1,B_3,R_3,B_6,R_2,B_4,R_4}$ Therefore, the sum of the numbers on the middle three cards is $3+3+6=boxed{mathrm{(E)} 12}$ .
Solution 1

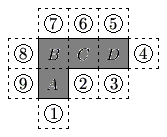Let the squares be labeled $A$ , $B$ , $C$ , and $D$ .
When the polygon is folded, the "right" edge of square $A$ becomes adjacent to the "bottom edge" of square $C$ , and the "bottom" edge of square $A$ becomes adjacent to the "bottom" edge of square $D$ .
So, any "new" square that is attatched to those edges will prevent the polygon from becoming a cube with one face missing.
Therefore, squares $1$ , $2$ , and $3$ will prevent the polygon from becoming a cube with one face missing.
Squares $4$ , $5$ , $6$ , $7$ , $8$ , and $9$ will allow the polygon to become a cube with one face missing when folded.
Thus the answer is $boxed{mathrm{(E)} 6}$ .

Solution 2

Another way to think of it is that a cube missing one face has $5$ of its $6$ faces. Since the shape has $4$ faces already, we need another face. The only way to add another face is if the added square does not overlap any of the others. $1$ , $2$ , and $3$ overlap, while squares $4$ to $9$ do not. The answer is $boxed{mathrm{(E)} 6}$
Solution 1

Since the area of square ABCD is 16, the side length must be 4. Thus, the side length of triangle AKB is 4, and the height of AKB, and thus DMC, is $2sqrt{3}$ .
The diagonal of the square KNML will then be $4+4sqrt{3}$ . From here there are 2 ways to proceed:
First: Since the diagonal is $4+4sqrt{3}$ , the side length is $frac{4+4sqrt{3}}{sqrt{2}}$ , and the area is thus $frac{16+48+32sqrt{3}}{2}=boxed{mathrm{(D)} 32+16sqrt{3}}$ .
Second: Since a square is a rhombus, the area of the square is $frac{d_1d_2}{2}$ , where $d_1$ and $d_2$ are the diagonals of the rhombus. Since the diagonal is $4+4sqrt{3}$ , the area is $frac{(4+4sqrt{3})^2}{2}=boxed{mathrm{(D)} 32+16sqrt{3}}$ .

Solution 2

Because $ABCD$ has area $16$ , its side length is simply $sqrt{16}implies 4$ .
Angle chasing, we find that the angle of $KBL=360-(90+2(60))=360-(210)=150$ .
We also know that $KB=4, BL=4$ .
Using Law of Cosines, we find that side $(KL)^2=16+16-2(16)cos{150}implies (KL)^2=32+frac{32sqrt{3}}{2}implies (KL)^2=32+16sqrt{3}$ .
However, the area of $KLMN$ is simply $(KL)^2$ , hence the answer is $32+16sqrt{3}implies {D}$ .
$[asy] import graph; size(150); defaultpen(fontsize(8)); pair A=(-2,0), B=(2,0); filldraw(Arc((0,sqrt(3)),1,0,180)--cycle,mediumgray); fill(Arc((0,0),2,0,180)--cycle,white); draw(Arc((0,0),2,0,180)--cycle); draw((0,0)--2*expi(2*pi/6)--2*expi(2*pi/6*2)--(0,0)); label("A",(0,2),(0,4)); label("B",(0,2),(0,-1)); label("C",(0,sqrt(3)/2),(0,2)); label("1",(-0.5,sqrt(3)/2),(-1,0)); label("1",(0.5,sqrt(3)/2),(1,0)); [/asy]$ Let $[X]$ denote the area of region $X$ in the figure above.The shaded area $[A]$ is equal to the area of the smaller semicircle $[A+B]$ minus the area of a sector of the larger circle $[B+C]$ plus the area of a triangle formed by two radii of the larger semicircle and the diameter of the smaller semicircle $[C]$ .The area of the smaller semicircle is $[A+B] = frac{1}{2}picdotleft(frac{1}{2}right)^{2}=frac{1}{8}pi$ .Since the radius of the larger semicircle is equal to the diameter of the smaller semicircle, the triangle is an equilateral triangle and the sector measures $60^circ$ .The area of the $60^circ$ sector of the larger semicircle is $[B+C] = frac{60}{360}picdotleft(frac{2}{2}right)^{2}=frac{1}{6}pi$ .The area of the triangle is $[C] = frac{1^{2}sqrt{3}}{4}=frac{sqrt{3}}{4}$ .So the shaded area is $[A] = [A+B]-[B+C]+[C] = left(frac{1}{8}piright)-left(frac{1}{6}piright)+left(frac{sqrt{3}}{4}right)=boxed{mathrm{(C)} frac{sqrt{3}}{4}-frac{1}{24}pi}$ .
$[asy] draw((0,10)--(8.660254037844385792,-5)--(-8.660254037844385792,-5)--cycle); dot((0,0)); label("$P$",(0,0),N); [/asy]$

Solution 1

After we pick point $P$ , we realize that $ABC$ is symmetric for this purpose, and so the probability that $ACP$ is the greatest area, or $ABP$ or $BCP$ , are all the same. Since they add to $1$ , the probability that $ACP$ has the greatest area is $boxed{mathrm{(C)} dfrac{1}{3}}$

Solution 2

We will use geometric probability. Let us take point $P$ , and draw the perpendiculars to $BC$ , $CA$ , and $AB$ , and call the feet of these perpendiculars $D$ , $E$ , and $F$ respectively. The area of $triangle ACP$ is simply $frac{1}{2} * AC * PF$ . Similarly we can find the area of triangles $BCP$ and $ABP$ . If we add these up and realize that it equals the area of the entire triangle, we see that no matter where we choose $P, PD + PE + PF$ = the height of the triangle. Setting the area of triangle $ABP$ greater than $ACP$ and $BCP$ , we want $PF$ to be the largest of $PF$ , $PD$ , and $PE$ . We then realize that $PF = PD = PE$ when $P$ is the in center of $triangle ABC$ . Let us call the in center of the triangle $Q$ . If we want $PF$ to be the largest of the three, by testing points we realize that $P$ must be in the interior of quadrilateral $QDCE$ . So our probability (using geometric probability) is the area of $QDCE$ divided by the area of $ABC$ . We will now show that the three quadrilaterals, $QDCE$ , $QEAF$ , and $QFBD$ are congruent. As the definition of point $Q$ yields, $QF$ = $QD$ = $QE$ . Since $ABC$ is equilateral, $Q$ is also the circum center of $triangle ABC$ , so $QA = QB = QC$ . By the Pythagorean Theorem, $BD = DC = CE = EA = AF = FB$ . Also, angles $BDQ, BFQ, CEQ, CDQ, AFQ$ , and $AEQ$ are all equal to $90^circ$ . Angles $DBF, FAE, ECD$ are all equal to $60$ degrees, so it is now clear that quadrilaterals $QDCE, QEAF, QFBD$ are all congruent. Summing up these areas gives us the area of $triangle ABC$ . $QDCE$ contributes to a third of that area so $frac{[QDCE]}{[ABC]}=boxed{mathrm{(C)} dfrac{1}{3}}$ .
Solution 1

Let $D$ be the origin. $A$ is the point $(0,4)$ and $M$ is the point $(2,0)$ . We are given the radius of the quarter circle and semicircle as $4$ and $2$ , respectively, so their equations, respectively, are: $x^2 + (y-4)^2 = 4^2$ $(x-2)^2 + y^2 = 2^2$ Subtract the second equation from the first: $x^2 + (y - 4)^2 - (x - 2)^2 - y^2 = 12$ $4x - 8y + 12 = 12$ $x = 2y.$ Then substitute: $(2y)^2 + (y - 4)^2 = 16$ $4y^2 + y^2 - 8y + 16 = 16$ $5y^2 - 8y = 0$ $y(5y - 8) = 0.$ Thus $y = 0$ and $y = frac{8}{5}$ making $x = 0$ and $x = frac{16}{5}$ .
The first value of $0$ is obviously referring to the x-coordinate of the point where the circles intersect at the origin, $D$ , so the second value must be referring to the x coordinate of $P$ . Since $overline{AD}$ is the y-axis, the distance to it from $P$ is the same as the x-value of the coordinate of $P$ , so the distance from $P$ to $overline{AD}$ is $frac{16}{5} Rightarrow B$

Solution 2

$APMD$ obviously forms a kite. Let the intersection of the diagonals be $E$ . $AE+EM=AM=2sqrt{5}$ Let $AE=x$ . Then, $EM=2sqrt{5}-x$ .
By Pythagorean Theorem, $DE^2=4^2-AE^2=2^2-EM^2$ . Thus, $16-x^2=4-(2sqrt{5}-x)^2$ . Simplifying, $x=frac{8}{sqrt{5}}$ . By Pythagoras again, $DE=frac{4}{sqrt{5}}$ . Then, the area of $ADP$ is $DEcdot AE=frac{32}{5}$ .
Using $4$ instead as the base, we can drop a altitude from P. $frac{32}{5}=frac{bh}{2}$ . $frac{32}{5}=frac{4h}{2}$ . Thus, the horizontal distance is $frac{16}{5} implies boxed{textbf{(E)}frac{16}{5}}$
Solution 1

When a $5$ -digit number is divided by $100$ , the first $3$ digits become the quotient, $q$ , and the last $2$ digits become the remainder, $r$ .
Therefore, $q$ can be any integer from $100$ to $999$ inclusive, and $r$ can be any integer from $0$ to $99$ inclusive.
For each of the $9cdot10cdot10=900$ possible values of $q$ , there are at least $leftlfloor frac{100}{11} rightrfloor = 9$ possible values of $r$ such that $q+r equiv 0pmod{11}$ .
Since there is $1$ "extra" possible value of $r$ that is congruent to $0pmod{11}$ , each of the $leftlfloor frac{900}{11} rightrfloor = 81$ values of $q$ that are congruent to $0pmod{11}$ have $1$ more possible value of $r$ such that $q+r equiv 0pmod{11}$ .
Therefore, the number of possible values of $n$ such that $q+r equiv 0pmod{11}$ is $900cdot9+81cdot1=8181 Rightarrowboxed{(B)}$ .

Solution 2

Notice that $q+requiv0pmod{11}Rightarrow100q+requiv0pmod{11}$ . This means that any number whose quotient and remainder sum is divisible by 11 must also be divisible by 11. Therefore, there are $frac{99990-10010}{11}+1=8181$ possible values. The answer is $boxed{textbf{(B) }8181}$ .

Solution 3

Let $abcde$ be the five digits of $n$ . Then $q = abc$ and $r = de$ . By the divisibility rules of $11$ , $q = a - b + c pmod{11}$ and $r = -d + e pmod{11}$ , so $q + r = a - b + c - d + e = abcde = n pmod{11}$ . Thus, $n$ must be divisble by $11$ . There are $frac{99990 - 10010}{11} + 1 = 8181$ five-digit multiples of $11$ , so the answer is $boxed{textbf{(B) }8181}$ .
Solution 1

If we take the parabola $ax^2 + bx + c$ and reflect it over the x - axis, we have the parabola $-ax^2 - bx - c$ . Without loss of generality, let us say that the parabola is translated 5 units to the left, and the reflection to the right. Then: $begin{align*} f(x) = a(x+5)^2 + b(x+5) + c = ax^2 + (10a+b)x + 25a + 5b + c \ g(x) = -a(x-5)^2 - b(x-5) - c = -ax^2 + 10ax -bx - 25a + 5b - c end{align*}$ Adding them up produces: $begin{align*} (f + g)(x) = ax^2 + (10a+b)x + 25a + 5b + c - ax^2 + 10ax -bx - 25a + 5b - c = 20ax + 10b end{align*}$ This is a line with slope $20a$ . Since $a$ cannot be $0$ (because $ax^2 + bx + c$ would be a line) we end up with $boxed{textbf{(D)} text{ a non-horizontal line }}$

Solution 2: less computation

WLOG let the parabola be $y=x^2$ , and the reflected parabola is thus $y=-x^2$ . We can also assume $[f(x) = (x+5)^2 = x^2 + 10x + 25]$ and $[g(x) = -(x-5)^2 = -x^2 + 10x - 25.]$ Therefore, $[(f+g)(x) = x^2 + 10x + 25 - x^2 + 10x - 25 = 20x,]$ which is a non-horizontal line $Rightarrow boxed{textbf{D}}$ .
The answer is $boxed{textrm{(A)}}$ .Note that the first five letters must be B's or C's, the next five letters must be C's or A's, and the last five letters must be A's or B's. If there are $k$ B's in the first five letters, then there must be $5-k$ C's in the first five letters, so there must be $k$ C's and $5-k$ A's in the next five letters, and $k$ A's and $5-k$ B's in the last five letters. Therefore the number of each letter in each group of five is determined completely by the number of B's in the first 5 letters, and the number of ways to arrange these 15 letters with this restriction is $binom{5}{k}^3$ (since there are $binom{5}{k}$ ways to arrange $k$ B's and $5-k$ C's). Therefore the answer is $sum_{k=0}^{5}binom{5}{k}^{3}$ .
Solution 1

Let the roots be $r_1=0, r_2, r_3, r_4, r_5$ . According to Vieta's formulas, we have $d=r_1r_2r_3r_4 + r_1r_2r_3r_5 + r_1r_2r_4r_5 + r_1r_3r_4r_5 + r_2r_3r_4r_5$ . The first four terms contain $r_1=0$ and are therefore zero, thus $d=r_2r_3r_4r_5$ . This is a product of four non-zero numbers, therefore $d$ must be non-zero $Longrightarrow mathrm{(D)}$ .

Solution 2

Clearly, since $(0,0)$ is an intercept, $e$ must be $0$ . But if $d$ was $0$ , $x^2$ would divide the polynomial, which means it would have a double root at $0$ , which is impossible, since all five roots are distinct.
If $A$ and $B$ meet, their paths connect $(0,0)$ and $(5,7).$ There are $binom{12}{5}=792$ such paths. Since the path is $12$ units long, they must meet after each travels $6$ units, so the probability is $frac{792}{2^{6}cdot 2^{6}} approx 0.20 Rightarrow boxed{C}$ .
Solution 1

We want to find the number of perfect square factors in the product of all the factorials of numbers from $1 - 9$ . We can write this out and take out the factorials, and then find a prime factorization of the entire product. We can also find this prime factorization by finding the number of times each factor is repeated in each factorial. This comes out to be equal to $2^{30}$ $*$ $3^{13}$ $*$ $5^5$ $*$ $7^3$ . To find the amount of perfect square factors, we realize that each exponent in the prime factorization must be even: $2^{15}$ $*$ $3^{6}$ $*$ $5^2$ $*$ $7^1$ . To find the total number of possibilities, we add $1$ to each exponent and multiply them all together. This gives us $16*7*3*2$ $=$ $672$ $Rightarrowboxed{mathrm{(B)}}$ .

Solution 2

( Just Explanation Of 1 )
We can easily find that factorials upto 9 in product lead to prime factorization $2^{30}$ $*$ $3^{13}$ $*$ $5^5$ $*$ $7^3$ So number of pairs possible = { $2^{0}$ $,$ $2^{2}$ $,$ ... $2^{30}$ }{ $3^{0}$ $,$ $3^{2}$ $,$ ... $3^{12}$ }{ $5^{0}$ $,$ $5^{2}$ $,$ ... $5^{4}$ }{ $7^{0}$ $,$ $7^{2}$ }
Which leads to resulting number of pairs = $16*7*3*2$ $=$ $672$ $Rightarrowboxed{mathrm{(B)}}$ .
Using logarithmic rules, we see that $[log_{a}a-log_{a}b+log_{b}b-log_{b}a = 2-(log_{a}b+log_{b}a)]$ $[=2-(log_{a}b+frac {1}{log_{a}b})]$ Since $a$ and $b$ are both positive, using AM-GM gives that the term in parentheses must be at least $2$ , so the largest possible values is $2-2=0 Rightarrow boxed{textbf{B}}.$ Note that the maximum occurs when $a=b$ .
Solution 1

The function $f(x) = sqrt{x(ax+b)}$ has a codomain of all non-negative numbers, or $0 le f(x)$ . Since the domain and the range of $f$ are the same, it follows that the domain of $f$ also satisfies $0 le x$ .
The function has two zeroes at $x = 0, frac{-b}{a}$ , which must be part of the domain. Since the domain and the range are the same set, it follows that $frac{-b}{a}$ is in the codomain of $f$ , or $0 le frac{-b}{a}$ . This implies that one (but not both) of $a,b$ is non-positive. The problem states that there is at least one positive value of b that works, thus $a$ must be non-positive, $b$ is non-negative, and the domain of the function occurs when $x(ax+b) > 0$ , or $0 le x le frac{-b}{a}.$ Completing the square, $f(x) = sqrt{aleft(x + frac{b}{2a}right)^2 - frac{b^2}{4a}} le sqrt{frac{-b^2}{4a}}$ by the Trivial Inequality (remember that $a le 0$ ). Since $f$ is continuous and assumes this maximal value at $x = frac{-b}{2a}$ , it follows that the range of $f$ is $0 le f(x) le sqrt{frac{-b^2}{4a}}.$ As the domain and the range are the same, we have that $frac{-b}{a} = sqrt{frac{-b^2}{4a}} = frac{b}{2sqrt{-a}} Longrightarrow a(a+4) = 0$ (we can divide through by $b$ since it is given that $b$ is positive). Hence $a = 0, -4$ , which both we can verify work, and the answer is $mathbf{(C)}$ .

Solution 2

If $f(x)=y$ , then squaring both sides of the given equation and subtracting $ax^2$ and $bx$ yields $y^2-ax^2-bx=0$ . Completing the square, we get $(x+frac{b}{2a})^2-frac{y^2}{a}=frac{b^2}{4a^2}$ where $ygeq 0$ . Divide out by $frac{b^2}{4a^2}$ to put the equation in the standard form of an ellipse or hyperbola (depending on the sign of $a$ ) to get $frac{(x+frac{b}{2a})^2}{(frac{b}{2a})^2}-frac{y^2}{(frac{b}{2sqrt {pm a}})^2}=1$ .
Before continuing, it is important to note that because $f(x)=sqrt{ax^2+bx}=sqrt{ax(x+frac{b}{a})}$ , $f(x)$ has roots 0 and $-frac{b}{a}$ . Now, we can use the function we deduced to figure out some of its properties when: $a>0$ : A semi-hyperbola above or on the x-axis. Therefore, no positive value of $a$ allows the domain and range to be the same set because the range will always be $[0, infty)$ and the domain will always be undefined on some finite range between some value and zero. $a=0$ : We must refer back to the original function; this results in a horizontal semiparabola in the first quadrant, which satisfies that the domain and range of the function are equal. Specifically, both sets are $[0, infty )$ . $a=0$ is the only case where this happens. $a<0$ : A semi-ellipse in quadrant one. Since its roots are 0 and $-frac{b}{a}$ , its domain must be $[0, -frac{b}{a}]$ . To make its domain and range equal, the maximum value of the ellipse must then be $-frac{b}{a}$ . But we have another expression for the maximum value of the ellipse, which is $0+frac{b}{2sqrt {pm a}}$ . Setting these two expressions equal to each other will find us the final value of $a$ that satisfies the question. $frac{b}{-a}=frac{b}{2sqrt {pm a}}$ $-a=2sqrt {pm a}$ $a^2=pm 4a$ $a=0,pm 4$ We already knew 0 was a solution from earlier, so -4 is our only new solution (we already ruled out any positive value of $a$ as a solution, so 4 does not work). Thus there are $boxed {2implies C}$ values of $a$ that make the domain and range of $f(x)$ the same set.